filmov
tv
Simple Harmonic Motion Derivations using Calculus (Mass-Spring System)
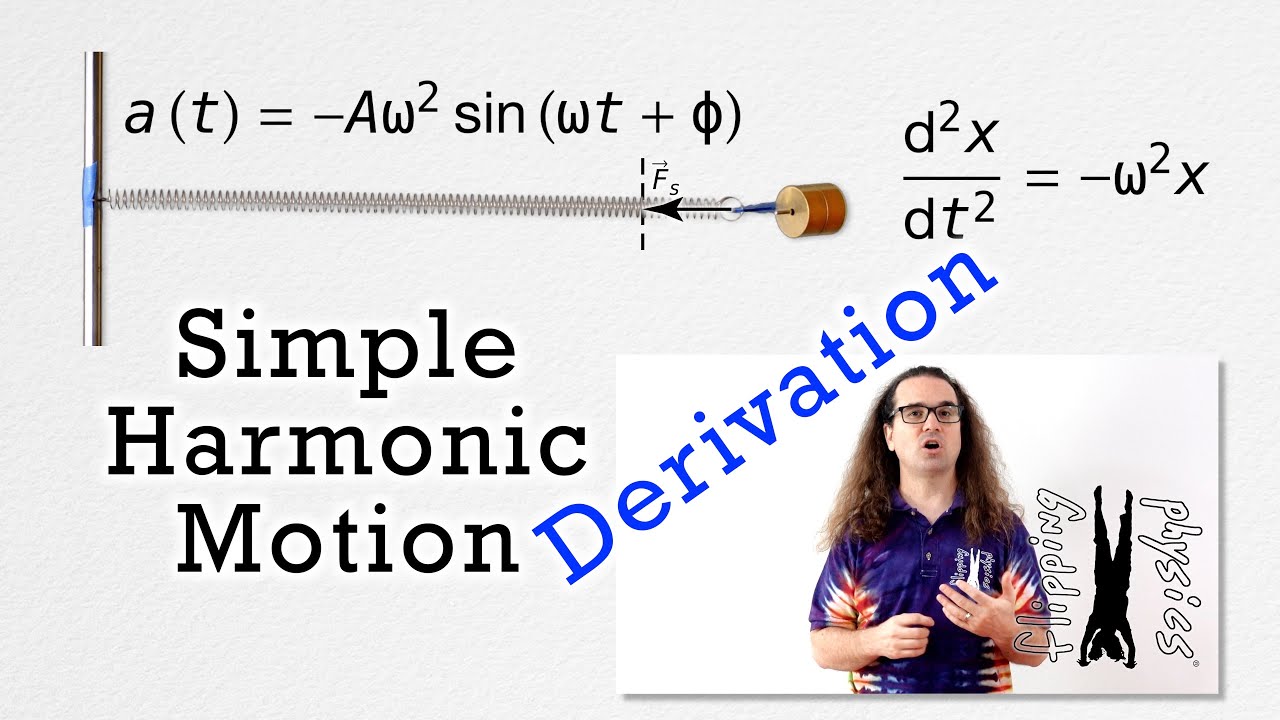
Показать описание
Content Times:
0:00 Simple Harmonic Motion (SHM) Review
0:28 Mass-Spring System
1:44 The SHM Mathematical Condition
3:06 Position Equation & Phase Constant
4:28 Velocity Equation
5:37 Acceleration Equation
6:36 Showing this is SHM
7:47 Period and Angular Frequency
10:41 Period of a Mass-Spring System
11:12 Review
Next Video: Simple Pendulum - Simple Harmonic Motion Derivation using Calculus
Previous Video: Simple Harmonic Motion Creating Circular Motion from Sine and Cosine Curves
#Derivation #SimpleHarmonicMotion #SHM
Simple Harmonic Motion Derivations using Calculus (Mass-Spring System)
Introduction to Simple Harmonic Motion (SHM) | General Equation and Derivation
Simple Harmonic Motion(SHM) - Position Equation Derivation
best simple harmonic motion equation.🌠
Simple Harmonic Motion: Hooke's Law
SHM Differential Equation of Simple Harminic Motion 2 VTU
Simple Pendulum - Simple Harmonic Motion Derivation using Calculus
Undamped Mechanical Vibrations & Hooke's Law // Simple Harmonic Motion
WAVES AND OPTICS (Long questions)
Simple Harmonic Motion: Crash Course Physics #16
MOST can't derive this equation
Where do the simple harmonic equations come from?
Simple Harmonic Motion
Simple Harmonic Motion(SHM) - Velocity and Acceleration Equation Derivations
How To Solve Simple Harmonic Motion Problems In Physics
How do you derive the period of oscillation for a pendulum?
Equation for simple harmonic oscillators | Physics | Khan Academy
Equations of Simple Harmonic Motion including Frequency and Period
Simple Harmonic Motion
Derivation of Equation in Simple Harmonic Motion
Deriving The Period Of A Pendulum Using SHM
simple harmonic motion (SHM) class 11 important formulas
Deriving the Simple Harmonic Motion equations from first principles | A Level Physics
Simple Harmonic Motion is Simple!
Комментарии
 0:12:58
0:12:58
 0:06:46
0:06:46
 0:08:14
0:08:14
 0:00:58
0:00:58
 0:04:49
0:04:49
 0:02:37
0:02:37
 0:13:30
0:13:30
 0:08:10
0:08:10
 0:17:35
0:17:35
 0:09:11
0:09:11
 0:09:30
0:09:30
 0:13:23
0:13:23
 0:11:06
0:11:06
 0:05:48
0:05:48
 0:14:11
0:14:11
 0:09:45
0:09:45
 0:14:14
0:14:14
 0:20:01
0:20:01
 0:00:25
0:00:25
 0:06:24
0:06:24
 0:02:20
0:02:20
 0:00:10
0:00:10
 0:20:53
0:20:53
 0:00:54
0:00:54