Oxford Calculus: Fourier Series Derivation

Check your working using the Maple Calculator App – available for free on Google Play and the App Store.

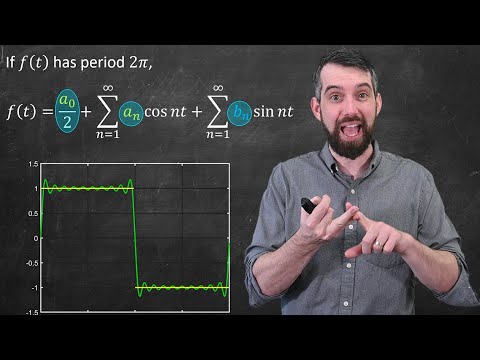
We start by deriving the orthogonality relations for sine and cosine, which are essential for the derivations of the Fourier Series coefficients. The integral relations rely on the trigonometric ‘product-to-sum formulae’ which enable the product of two sine or cosine terms to be separated and thus integrated directly. The delta function is also introduced to help to simplify the notation.

We then assume that a Fourier Series of the required form exists, with as yet unknown coefficients a0, an and bn. These are derived by first integrating the entire equation from -L to L to get a0; then multiplying by cosine and integrating to get the an coefficients for each n; and finally multiplying by sine and integrating to get the bn coefficients for each n. The integrals are evaluated using the previously derived orthogonality relations.

Finally, the interchanging of the summation and integral signs is addressed with a very brief discussion of uniform convergence and what this means in the context of a series.

Don’t forget to check out the other videos in the ‘Oxford Calculus’ series – all links below.










You can also follow Tom on Facebook, Twitter and Instagram @tomrocksmaths.

Get your Tom Rocks Maths merchandise here:

[larzcaetano]

Finally!!! Please, make a derivation of Laplace Transform!

[nychan]

I appreciate you really did the integrations and didn't just state the results.

[griffinarcher]

So grateful for these videos. I watched some other lectures from big universities and they don't really explain anything, just write terms on the board and expect it to be understood. You're a great teacher and I feel that I finally understand this topic; I always have a lot of questions that go unanswered in other videos so I appreciate the way you explain your steps thoroughly. Great video!

[leeris]

I am a computer science student, currently delving around machine learning and it brought me here. I once again am reminded of how beautiful mathematics can be!

[ed.puckett]

Thank you for talking about the interchange of summation and integration and the property of uniform convergence!

[MynulShanto]

Perhaps this is the easiest video on Fourier series on YouTube. Doing integration ( specially showing the value of delta (mn) ) makes this video unique. That's why I easily grasped the concept how to identify the co-effcient. Thanks a lot. My professor just messed up the topic and made it hard to learn.

[leophoenixmusic]

Great video!
Adding to the list of people suggesting this: would love to see how to derive the Fourier transform from Fourier series. 😊

[JosephJoestar_]

Studying physics rn, this helped much! Thx!!!

[KenStarkey]

I loved the derivation. I could only imagine how Fourier felt when he derived or invented this. Seems so clear but we know some steps.
Also reversing the sum with the integral you said we know it converges to f(x). I thought that was what you were trying to prove.

[KaiKunstmann]

Each of the 3 positions contains each decimal digit 1/10 of the time (if you count leading zeros). So each digit appears 3×1000/10 = 300 times.
More generally: In a list of all possible n-positional integers (allowing for leading zeros) each base-b digit appears exactly n·b^(n-1) times, e.g. b=10 and n=3 is 3·10^(3-1) = 3·10² = 300.

[M.athematech]

For orthogonality, for m=n, you only covered m=n>0, the case m=n=0 has to be treated as a special case or else you are again dividing by 0.

[physicsadhyaapak]

Please make a video on Fourier, Laplace Transforms and Special Functions such as Bessel functions, Hermite, Legendre Functions.

[family-accountemail]

Just want to say chalk on a board presentation can be very good! Children for a while now never see chalkborads in schools ( here in UK ) and I have been laughed at for being so old as to remember chalk! My dad take about learning to write on a slate?

[nickbishop]

Probably pretty low-brow for you, but can you do one on induction proofs?

[NikitaPetrovich-zyhp]

Why did u decide to integrate this eqation on 23:12 ?

[dmitriiefimov]

Oh my, just came a minute shy to the end :) Tom, is there a way to describe a FT in terms of SU(2) Lie group? And I didn't watch the video yet - just in case the answer is out there - I'll do in a moment!

[Academy-wr]

Hi. Im a 11th grade student. I didnt understand the part where: integrating the sin function, then inputting x=L gives zero (while deriving last RHS term for a0). I get why it happens for a cos function, we integrate it and it becomes sin, and every integer multiple of pi for sin is but for sin when we integrate, it become cos function... which is not 0 at every integral multiple of pi right? If you could clarify this doubt asap it'd be of help

[dennisyang]

Hi Tom can you make a video testing the new AI chatGPT's maths skills by asking it a bunch of maths questions. I've used it a couple of times and it seems to be quite knowledgeable to a degree.

[evazhang]

integration of cos would not be 0 at 30:43, would you please double check when you get a chance please?

[michaelmapple]

16:00 Odd number times even number is even. Got a bit confused for a while