Fourier Series: Part 1

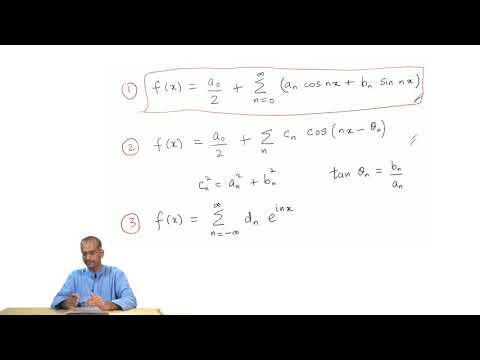
This video will show how to approximate a function with a Fourier series, which is an infinite sum of sines and cosines. We will discuss how these sines and cosines form a basis for the space of functions.

These lectures follow Chapter 2 from:
"Data-Driven Science and Engineering: Machine Learning, Dynamical Systems, and Control" by Brunton and Kutz

This video was produced at the University of Washington

[byynee]

Fourier series as inner products and projections onto orthogonal basis. I'm in awe! Can't thank you enough Prof. Steve Brunton!

[minghanzhu]

I learned Fourier Transforms 5 years ago and today is the first day I fully understand it from the vector space point of view. Thank you so much.

[kamalabouzhar]

It makes a lot of sense to think about the coefficient A_k and B_k as projections on the orthogonal basis formed by cos and sine. Never thought about it this way ;) it always good to learn and relearn the same concept from different perspectives.

[iwakeupsad]

Hi Steve! I've been a sound designer working and building with Fourier EQ's and Compressors for years and years BUT never has someone explained it to me like this. I can't thank you enough. I feel like I get my job and my art now.... and it feels great.

[BoZhaoengineering]

Thinking of sine and cosine as basis, function space is span ( sine, cosine). This really make sense when I was trying to gain a solid understanding of Fourier ‘ s method. Thank you very much for your instruction video.

[rhke]

I have read dozens of books and viewed dozens of videos on this topic. This series is the only one that marries intuition with process and procedures and explain why. Congrats. I highly recommend to everyone.

[sandlertossone]

After all the calculus, physics, signals and systems courses I have taken, the only thing I understood is how to do them and not what they actually mean. I was worried about not getting answers from the books, online, or my professors because I want to teach in the future. This is a game changer and was like inventing the light bulb to me. I can't thank you enough for not only explaining it in detail, but doing it in a simple and understandable way.

[djmips]

I'm not trying to impress anyone, but after a lot of thinking over the years (I'm a game programmer) I came to this idea on my own but I was never sure if I was correct. This is the first time I've seen a presentation that clearly confirms what I had conjectured. I'm learning a lot of details now. I will continue on with this series as I am quite curious to know more and confident that I can understand your presentation format.

[mohamededbey]

I've just found out about this awesome channel. You sir are wonderful.
Please don't stop the good work 😉

[erockromulan]

I just got the book and I'm starting Chapter 2 with a major head scratch. Then I watch this video and it's like taking a blindfold off. Thank you, Steve!!

[jeremystookey]

The best Fourier Analysis videos taught by a floating torso on YouTube.
Thank you Steve. You've made this topic much easier to understand.

[farhanmadilfaraz]

You made me fall in love with mathematics again. Great work, Thank You so much.

[whenmathsmeetcoding]

What a great interpretation of Fourier series with vector spaces, I am proud to say I do follow quite a few channels and your's work is best among them.

[amribrahim]

That's literally the best explanation I've ever heard about Fourier series. Thank you!

[pairadeau]

If there is such a thing as pedagogical wizardry you're witnessing it. This channel is superbly good.

[gauravbhokare]

This is the most simple, elegant and beautiful explanation (IF YOU KNOW LINEAR ALGEBRA) I've ever seen. Thank you Prof. Brunton.

[mugiwara-no-luffy]

The analogue for projecting f onto orthogonal vector basis to explain projecting f onto orthogonal functions was really helpful and made everything click for me. Thanks!

[majdabouakil]

Suddenly these formulas appear to be very simple and I'll probably never forget them. Thank you very much.

[jairjuliocc]

I really like maths and machine learning and after long time trying, i finally understand fourier series with this video .Thank you so much!!. Greetings from Peru

[isaacharris]

The intuition you provide regarding the inner products is incredible. So helpful in understanding this difficult topic. Wish you were my Prof. when I learned Fourier Series in class!