filmov
tv
5 = 3 + 4? The Staircase Paradox. Spot The Mistake 'Disproving' The Pythagorean Theorem

Показать описание
The Pythagorean Theorem says the square of the hypotenuse is equal to the sum of the squares of the sides. But this video says the hypotenuse is simply the sum of the sides. How is that possible? Can you figure out the mistake in the logic?
Connect on social media. I update each site when I have a new video or blog post, so you can follow me on whichever method is most convenient for you.
My Books
Connect on social media. I update each site when I have a new video or blog post, so you can follow me on whichever method is most convenient for you.
My Books
Κωνσταντίνος Κουφός - Μετρώ Αντίστροφα (5,4,3,2,1) | Official Music Video [HD]...
5 = 3 + 4? The Staircase Paradox. Spot The Mistake 'Disproving' The Pythagorean Theorem
5 3/4 as a Decimal (Five and Three-Quarters)
5 Divided by 3/4 (Five Divided by Three-Fourths)
5 times 3/4 (Five times Three-Fourths)
5 3/4 a fraccion impropia, convertir fraccion mixta a fraccion impropia
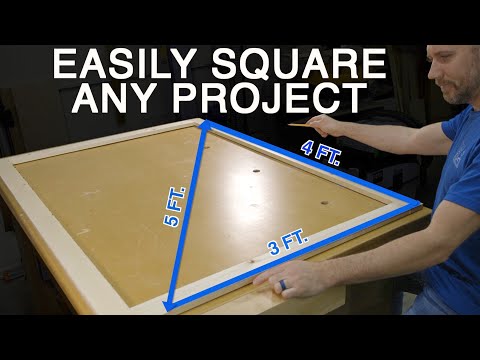
How To Use The 3-4-5 Rule To Make A Square - DIY At Bunnings
3-4-5 Triangle Method For Finding Square
Scary teacher level 1,2,3,4,5,6,7,8,9,10,11,12,13,14 complete
5 mas 3/4 , suma de un numero entero mas una fraccion 5+3/4
Praxis: Rechter Winkel mit der 3-4-5 Methode
3-4-5 Method, How to get a perfect right angle when building structures.
1, 2, 3, 4, 5, Once I Caught a Fish Alive! | CoComelon Nursery Rhymes & Kids Songs
ASTOUNDING: 1 + 2 + 3 + 4 + 5 + ... = -1/12
Adding Fractions- 3/4 + 5/6
Learn Number Counting 1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20 | Numbers By RV AppStudios
How To Make A Perfect Right Angle [3-4-5 Method]
Gavin Harrison: Grooving With 3's 4's and 5's - Drum Lesson (Drumeo)
Jacob Collier's 2 / 3 / 4 / 5 / 6 polyrhythm solved!
Subtracting a Fraction from a whole number
Wofür nutzt man die 3-4-5-Methode? | Quick Tips ☀️ Pina
How to Measure 4 Litres, with a 5 Litre and 3 Litre Container - Step by Step Instructions - Tutorial
✅ Cómo Sacar una ESCUADRA PERFECTA Método 3-4-5 ▶︎ TEOREMA PITÁGORAS
CBeebies: Something Special - 1, 2, 3, 4, 5 Once I Caught a Fish Alive - Nursery Rhyme
Комментарии
 0:03:16
0:03:16
 0:01:48
0:01:48
 0:01:21
0:01:21
 0:01:21
0:01:21
 0:01:09
0:01:09
 0:00:22
0:00:22
 0:00:22
0:00:22
 0:05:49
0:05:49
 0:47:21
0:47:21
 0:00:43
0:00:43
 0:03:50
0:03:50
 0:04:24
0:04:24
 0:03:35
0:03:35
 0:07:50
0:07:50
 0:01:50
0:01:50
 0:12:30
0:12:30
 0:03:50
0:03:50
 0:02:44
0:02:44
 0:00:36
0:00:36
 0:02:42
0:02:42
 0:01:48
0:01:48
 0:02:09
0:02:09
 0:06:15
0:06:15
 0:00:44
0:00:44