Mathematical Physics Fourier Series

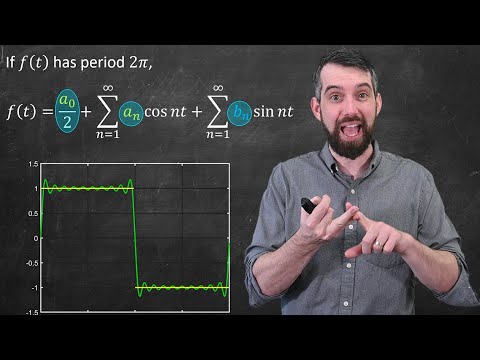
Mathematical Physics Fourier Series - Helpful for BSc / MSc / BTech Engineering Mathematics students - It is a powerful mathematical physics tool that decomposes periodic functions into sums of sine and cosine terms. This basic technique is fundamental in mathematical physics, especially for solving differential equations in fields like heat conduction, wave motion, and electromagnetism. Basics of Fourier Series help simplify complex physical problems in mathematical physics by breaking them down into manageable components. In mathematical physics, the Fourier series is often applied to problems in fluid dynamics, signal processing, and quantum mechanics. Using the Fourier series and its properties, physicists can solve complex boundary value problems in mathematical physics. A deep understanding of the mathematical methods of physics is essential for mastering mathematical physics. The mathematical methods of physics include techniques like the Fourier series in physics, which allow for the practical analysis of physical systems. In mathematical physics, these methods, including the Fourier series, derive solutions to partial differential equations, such as the heat or wave equation. The Fourier series is precious when dealing with periodic boundary conditions, common in mathematical physics problems. Understanding the mathematical methods of physics allows students to approach and solve problems in theoretical and applied physics with greater confidence and skill. Mastering the concepts of the Fourier series and other mathematical methods of physics is vital for analyzing wave propagation, quantum fields, and many other phenomena. These tools form the foundation of mathematical physics and are indispensable in academic study and real-world applications.

00:00 Introduction
02:17 Orthogonality Property
09:29 Equations for Orthogonal Condition

[ProfSivakumarRajagopalan]

Playlist for Fourier Series

Playlist for Laplace Transform

Playlist for Series Solution of Differential Equations

Playlist for Vector Spaces and Groups

Playlist for Group Theory (by Prof SVMS)

Playlist for BSc Electricity and Magnetism

Playlist for MSc Classical Mechanics

Playlist for MSc Classical Electrodynamics

[hariprabhu]

I admire your passion for teaching sir.. 🙏

[saynora]

Thank you sir, your lecture is so informative and helpful to build our concepts clearly

[jagatacharjee]

Prof, please upload in a regular basis, meaning on a regular interval of time

[vrangarajan]

Thank you air, for a wonderful lecture. Isn't zero also a member of the orthogonal set ? for n equal to zero --> Sin nx --> 0. Thanks

[Quasar]

Sir, how does the condition for verifying the orthagonality translate to the functions being orthagonal to eachother (which is by my basic understanding defined as the tangents of the functions at any point being perpendicular to each other). What is the insight behind multiplying two functions and taking the integral of the resultant over its period?.