Volume of Revolution - Comparing the Washer and Shell Method

Показать описание

This video compares how to determine volume of revolution using washers and shells.

Рекомендации по теме

Комментарии

You are the real MVP, studying for my calc exam . Thank you!!

glo

I found this review very helpful. I never really understood when was the best time to execute the shell method or the disk method and this really cleared things up for me.

MichaelQuotesTheBible

By far the best explanation of the difference between washer/disk and shell I've ever seen.

KB-vdwq

great video to demonstrate the comparisons of shell vs washer...trying to prepare for a cal2 midterm

charlesairiohuodion

you're the best! thanks for this video, my teacher did not explain this very well and you did it perfectly!

kevinchau

He's missing a squared in the washer method for the rotation around y-axis.

arnoldkwok

"When you use disk/washer method you will cut the solid into infinite no. of disks and integrate all of them to find total volume." i.e. you will summarize the area for an infinite no. of disks/circles. the area of a circle is pi(r^2)

When you use the shell method you summarize the volumes of an infinite number of shells. And the volume of a shell is 2Pi*r

kaspera

Great video! But at 8:07, shouldn't it be ((y-2)^2)^2?

yankeefan

Well done. This was exactly the explanation I needed. Thank you.

Mikeyman

Thank You! I have a better understanding now :-)

joanneho

This was a very good video! Much learned!

civice

Using the shell method, h(y) is not squared.

Mathispoweru

Can you do a video rotating about x=a or y=a?

ken

Thank you really broke it down nicely.

palowjanem

Why is the shell method 2pi while the washer is only pi?

_FootHand

I know this is crazy, but you asked. Think about the protection of a condom's surface. It protects what goes in/out of the two "surface areas" (or "functions" if your dirty enough to call it that) One side holds a higher value.

The "disk method" portrays a function that flows from an intrinsic function projecting outward
The "washer method" portrays a function the flows from an extrinsic function projecting inward

And vice versa if you flip the coordinates of x and y...z is where it gets "fun"

mikaelgarcia

at 8:15 shouldn't it be (y-2)^4 because it gets squared again?

Gaurav-rveh

Volume of Revolution - Comparing the Washer and Shell Method

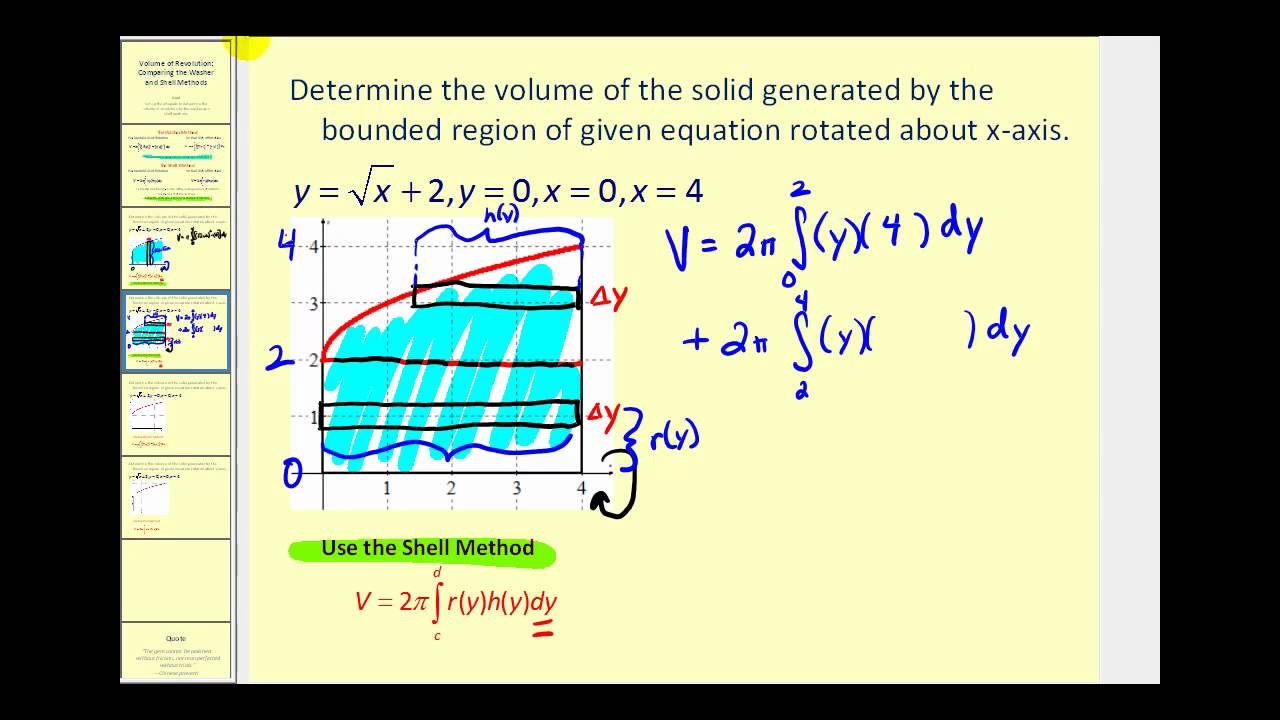
Volume of Revolution - Comparing the Washer and Shell Method

Comparing Methods of Finding Volume

Shell Method - Volume of Revolution

Volume of Revolution (Cylindrical Shells)

Disk/Washer vs. Cylindrical Shell...when to use which?

Overview and Comparison Disk and Shell Methods

Disc/Washer Method vs. Shell Method (rotated about different lines)

Intro to Solids of Revolution (1 of 3: Establishing the formula)

Calc 2, Lec 13B, Proof Using Comparison Test for Improper Integrals, Volumes of Solids of Revolution

Calculating Volume by Cylindrical Shells

Introduction to Volumes by Cylindrical Shells: Visual Comparison with Slicing

Calculus 1 Lecture 5.2: Volume of Solids By Disks and Washers Method

Calculus Volumes of Revolution Examples

Solid of revolution between two functions (leading up to the washer method) | Khan Academy

Volume of Revolution - The Disk Method

Volume of revolution - washers

Volumes: Examples around x-axis & y-axis

Solids of Revolution - Subtracting Volumes

Volume of Solid of Revolution Disk Method and Washer Method Calculus 1 AB (Read Description)

Volumes of Revolution - Cylindrical Shells (Two Examples)

Ex 3: Volume of Revolution Using the Disk Method (Exponential Function)

Volumes of Revolution - Disk/Washers Example 3

Volume of Revolution - The Shell Method about the y-axis

Ex: Volume of Revolution - Disk Method (Quadratic Function)