filmov
tv
Calc 2, Lec 13B, Proof Using Comparison Test for Improper Integrals, Volumes of Solids of Revolution

Показать описание
Calculus 2, Lecture 13B.
(0:00) Prove that the improper integral of (2 + sin(t))/(t^2 + 1) from 1 to infinity converges using the comparison test.
(8:14) Volumes of solids of revolution. Example: Rotate the region bounded by y = x^2 between x = 0 and x = 2 about the x-axis.
(18:04) Rotate the region bounded by y = x^2 and y = sqrt(x) between x = 0 and x = 2 about the horizontal line y = -2.
(27:51) Check answer with Mathematica.
(0:00) Prove that the improper integral of (2 + sin(t))/(t^2 + 1) from 1 to infinity converges using the comparison test.
(8:14) Volumes of solids of revolution. Example: Rotate the region bounded by y = x^2 between x = 0 and x = 2 about the x-axis.
(18:04) Rotate the region bounded by y = x^2 and y = sqrt(x) between x = 0 and x = 2 about the horizontal line y = -2.
(27:51) Check answer with Mathematica.
 0:29:30
0:29:30
 0:00:48
0:00:48
 0:33:11
0:33:11
 0:00:29
0:00:29
 0:35:15
0:35:15
 0:00:36
0:00:36
 0:00:14
0:00:14
 0:08:07
0:08:07
 0:06:20
0:06:20
 0:00:15
0:00:15
 0:15:25
0:15:25
 0:09:40
0:09:40
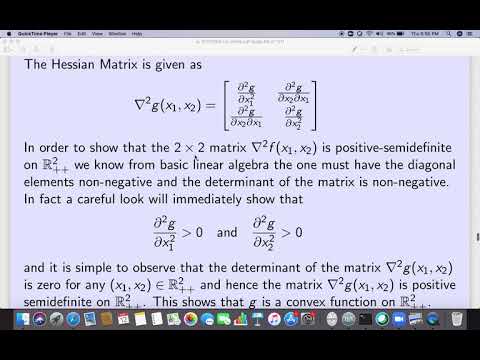 0:22:17
0:22:17
 0:25:39
0:25:39
 0:18:18
0:18:18
 0:09:13
0:09:13
 0:08:30
0:08:30
 0:18:04
0:18:04
 0:46:43
0:46:43
 1:17:59
1:17:59
 0:33:49
0:33:49
 0:06:29
0:06:29
 0:20:45
0:20:45
 0:39:12
0:39:12