📚 Solving Quadratic Equations with the Square Root Property and Completing Square! 🧮

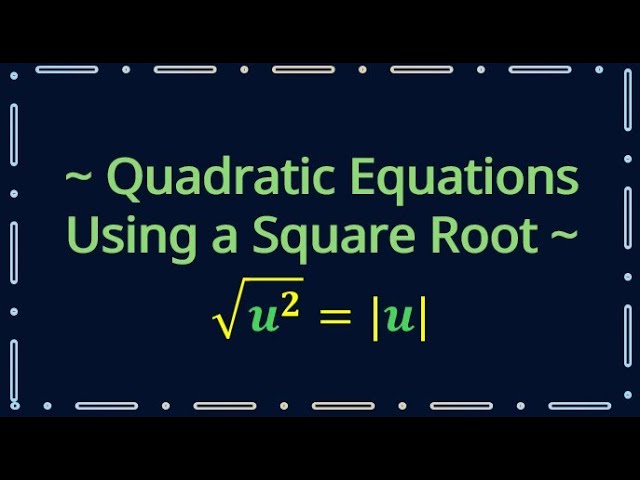
In this lesson, we dive into solving quadratic equations using the Square Root Property. . Watch as we unravel common misconceptions and demonstrate step-by-step solutions to a variety of quadratic equations.

🚀 What You’ll Learn:
✅ The concept of absolute value in square root properties.
✅ How to apply the square root property to solve quadratic equations.
✅ Examples ranging from simple isolations to the method of completing the square.
✅ How to handle quadratic equations with irrational solutions.

🔑 Key Highlights:
.
✅ Solving Quadratic Equations:
     ✅ Isolating perfect squares.
     ✅ Using absolute value equations.
     ✅ Applying the method of completing the square.
✅ Practical examples for equations with positive, negative, and fractional solutions.
✅ Understanding factorization and non-factorable quadratics.
✅ Handling equations with fractions and irrational roots.
✅ Perfect square trinomials and completing the square for non-factorable equations.

Whether you're a student mastering quadratic equations or revisiting algebra, this tutorial will strengthen your understanding and problem-solving skills. ✨

👉 Subscribe for more math tutorials: [Your Channel Link]

#MathTutorials #QuadraticEquations #SquareRootProperty #AlgebraHelp #CompletingTheSquare #MathForBeginners #AlgebraMadeEasy #STEMEducation #LearnMath #MathLessons #MathTips #EquationSolver #HighSchoolMath #CollegeMath #MathConcepts #StudyWithMe #MathMadeSimple #EducationalVideos #OnlineLearning #MathExplained #samsoleymani #mathetica

[samsoleymanimath]

For Example 4, the left-hand side should be (x-3)^2. The equation turns into |x-3|=5. Using the same approach the solutions are x=-2 or x=8.