filmov
tv
Are the real numbers... REAL?

Показать описание
The first video in a series which will build up to defining the p-adic numbers. In this episode, we break down our intuitive understanding of the real numbers, and build it back up again using the formality of Cauchy sequences.
#some2 #SoME2
Introduction (0:00)
Non-definitions (0:50)
Playing with sequences (3:35)
The definition of Cauchy sequences (5:31)
Important side note! (8:35)
Quotient your problems away! (9:11)
The definition of the real numbers (14:00)
0.999... = 1 (14:57)
Denouemont (16:35)
#some2 #SoME2
Introduction (0:00)
Non-definitions (0:50)
Playing with sequences (3:35)
The definition of Cauchy sequences (5:31)
Important side note! (8:35)
Quotient your problems away! (9:11)
The definition of the real numbers (14:00)
0.999... = 1 (14:57)
Denouemont (16:35)
Are the real numbers... REAL?
How real are the real numbers, really?
What are the Types of Numbers? Real vs. Imaginary, Rational vs. Irrational
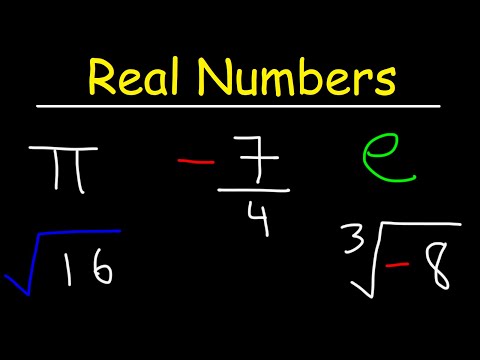
Real Numbers
WHAT IS A REAL NUMBER? WHOLE NUMBER? NATURAL NUMBER?
What are Real Numbers? | Don't Memorise
An Alternative Proof That The Real Numbers Are Uncountable
What are Real Numbers ? Number System
Real Numbers FULL CHAPTER | Class 10th Mathematics | Chapter 1 | INTRODUCTION VIDEO | @prayagstudy
Imaginary Numbers Are Real [Part 1: Introduction]
Classification of Numbers (Natural, Whole, Integers, Rational, Irrational, Real) - Nerdstudy
Rational, Irrational and Real Numbers
The Real Number System
Are Imaginary Numbers Real?
Properties of Real Numbers
Pre-Algebra 33 - Real Numbers
S01.9 Proof That a Set of Real Numbers is Uncountable
Categories of the Real Numbers | Don't Memorise
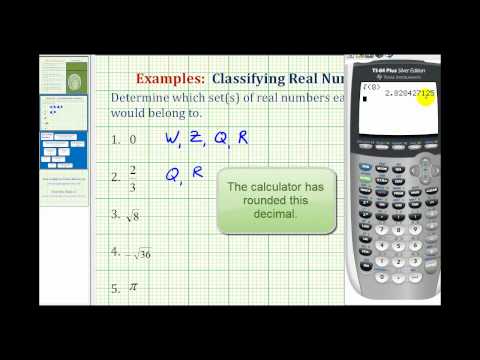
Identifying Sets of Real Numbers
RA1.1. Real Analysis: Introduction
Set of Real Numbers | Subsets of Real Numbers | Set Symbols in Math [Animated] - Pre-Algebra
'Master Real Numbers Like Never Before | Symbiosis of Concepts Explained!'
Amazing Tricks to Understand Real Numbers | Real Numbers | Letstute
What Are The Real Numbers?
Комментарии
 0:16:56
0:16:56
 0:07:52
0:07:52
 0:09:00
0:09:00
 0:24:46
0:24:46
 0:03:08
0:03:08
 0:03:37
0:03:37
 0:07:20
0:07:20
 0:05:44
0:05:44
 0:04:01
0:04:01
 0:05:47
0:05:47
 0:07:58
0:07:58
 0:04:36
0:04:36
 0:06:22
0:06:22
 0:05:54
0:05:54
 0:10:00
0:10:00
 0:08:23
0:08:23
 0:04:02
0:04:02
 0:03:35
0:03:35
 0:06:50
0:06:50
 0:10:41
0:10:41
 0:03:42
0:03:42
 0:25:31
0:25:31
 0:10:35
0:10:35
 0:04:57
0:04:57