filmov
tv
Are Imaginary Numbers Real?

Показать описание
The Imaginary number i was much debated long ago, yet is widely accepted now. But the question is: are imaginary numbers real? I mean, they're "imaginary" right? They don't actually exist....do they?
►BECOME A CHANNEL MEMBER
Disclaimer: This video is for entertainment purposes only and should not be considered academic. Though all information is provided in good faith, no warranty of any kind, expressed or implied, is made with regards to the accuracy, validity, reliability, consistency, adequacy, or completeness of this information.
#math #brithemathguy #imaginarynumbers
Are Imaginary Numbers Real?
Imaginary Numbers Are Real [Part 1: Introduction]
Do Complex Numbers Exist?
The Real World Uses of Imaginary Numbers
Imaginary Numbers Are Not Imaginary | Jeff O'Connell | TEDxOhloneCollege
How Imaginary Numbers Were Invented
What are the Types of Numbers? Real vs. Imaginary, Rational vs. Irrational
Imaginary Numbers Are Just Regular Numbers
Necessity of complex numbers
Imaginary Numbers - Basic Introduction
10 - What are Imaginary Numbers?
Imaginary Numbers Explained Bob Ross Style
Where do Imaginary Numbers come from?
The true history of complex numbers.
Imaginary Numbers are Not 'Imaginary'! In 5 Levels of Complexity
What's the physical meaning of imaginary numbers?
Imaginary Numbers Are Real [Part 2: A Little History]
Complex Numbers - Introduction to Imaginary Numbers | Don't Memorise
What is a Complex Number
Why do Electrical Engineers use imaginary numbers in circuit analysis?
Imaginary numbers are real
Real Numbers
Imaginary Numbers Are Real [Part 7: Complex Multiplication]
How Imaginary Numbers Make Real Physics Easier to Understand
Комментарии
 0:05:54
0:05:54
 0:05:47
0:05:47
 0:11:26
0:11:26
 0:16:49
0:16:49
 0:10:04
0:10:04
 0:23:29
0:23:29
 0:09:00
0:09:00
 0:09:02
0:09:02
 0:07:39
0:07:39
 0:14:12
0:14:12
 0:38:00
0:38:00
 0:11:23
0:11:23
 0:01:00
0:01:00
 0:05:43
0:05:43
 0:13:59
0:13:59
 0:06:03
0:06:03
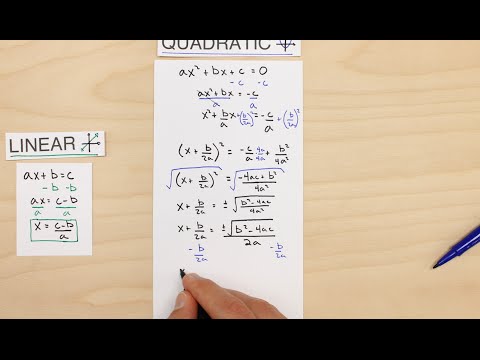 0:05:16
0:05:16
 0:04:50
0:04:50
 0:01:00
0:01:00
 0:13:08
0:13:08
 0:02:02
0:02:02
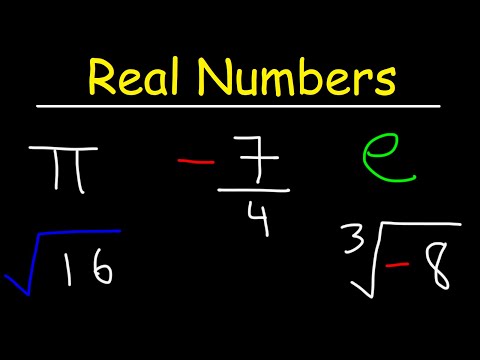 0:24:46
0:24:46
 0:04:25
0:04:25
 0:16:17
0:16:17