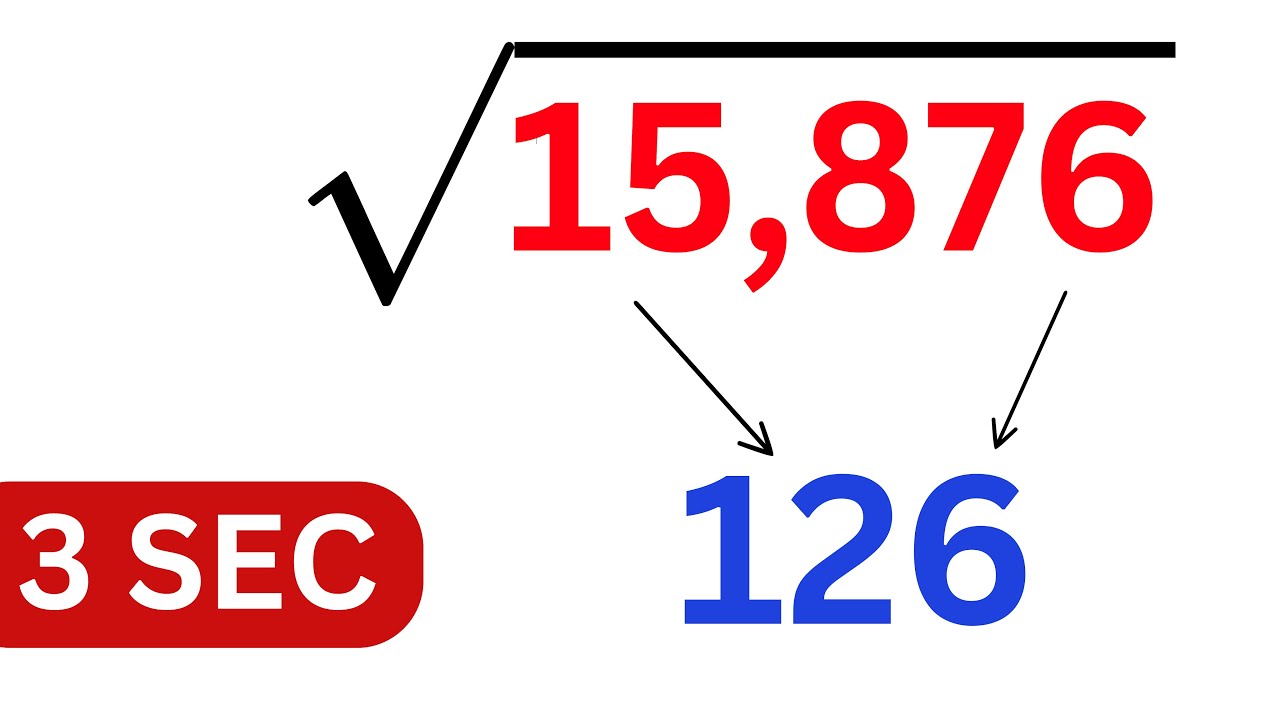
Square Root in 3 seconds - Crazy Math Trick

Square Root in 3 seconds - Crazy Math Trick | Math Olympiad | Harvard University Entrance Exam Interview | This question frightened 300K+ examinees! | Hard Geometry Exam Question | Only 1% of Students Got this Math Question Correct

If you're reading this, drop a comment using the word "Root". Have an amazing day, you all are awesome!

[SnowyPlayer]

Since this trick only applies to perfect squares, could you tell me how do I find out whether the number is a perfect square or not without the need to taking the square root of it, please?

[greninjamariokartpokemonfan]

58²=3, 364

(a+b)²=a²+2ab+b²
(50+8)(50+8)
2, 500+2(400)+64
2, 500+800+64
3, 300+64=3, 364

(a-b)²=a²-2ab+b²
(60-2)(60-2)
3, 600-2(120)+4
3, 600-240+4
3, 360+4=3, 364

[vibhashrivastava]

Vedic maths method from india.
Good to see the world learning this method ❤

[denysmace]

It's a nice trick, but one small quibble. You started off saying you only need to learn the squares of 0 through 9, and you end by casually throwing in the square of 12.

[aaditmordani]

@Brain Station how did you get 12 x 13 in 4:15

[Steven-vl]

misleading ... the trick allows you to quickly recognize a perfect square. it would take a normal person more like 30 seconds to apply the trick

[krishnatripathi]

How would we know that which number is a perfect square

For example:-
As we know that 144 is square of 12 which is also determined by your trick but with your trick 134 is also look like square of 12

[rmadhavan]

can you give some extra problems after each vid?? it would help al lot

[SnapShorts]

this would 100% take longer than 3 seconds for starters

[Evan-zt]

Can you make more videos that teaches tricks like this

[scmtuk]

There is no way most people would be able to pick the number(s) who's square ends with the same digit as the square we're trying to find the root of, then remove the last two digits of the answer, then find the number who's square is less than the remaining part of the number without the last two digits, then multiply that number by the next number, then choose the greater of the two possible last digits, if the original number is greater than the number we got by multiplying the number who's square is less than the part of the number we got by removing the last two in 3 seconds.

Also, if the difficulty of a task is unrelated to the time it takes to do a task, then another way of finding the square root of a number, is the use of prime factorization.

For example, for 3364, we will find the prime factorization:
3364 | 2
1682 | 2
841 | 29
29 | 29
1
Now we simply write the product of the numbers in the second column, like so:
2 x 2 x 29 x 29

To simplify this, we can then rewrite this expression in the form of exponents:
2² x 29²

Then we can simply take the square root of this product, to find the answer is 2 x 29 = 58.

[Sħmabdul]

this is helpful you are a good youtuber

[Maths_Wonderland]

Which app do you use to edit your videos?

[Sweetcow]

Tysm this helps me save so much time in solving problems

[DrMattFen]

If I only had 3 to 5 seconds i would have guessed wrong.. Might be easier to just "simply" _memorise_ them all..

[benjamin-richephelem]

I think you should kindly give reasons why you choose the lower number in some cases and bigger number in other cases please?

[_Paps]

whats the thing/rule that allows this trick to actually work?

[jeffw]

Another quick way to find the correct answer between two choices is to cast out nines. For example, if the square root of 729 is either 23 or 27, we can first check 23 as a root by adding the digits together. 2+3 is 5, which gives us a remainder of 5. 23x23 gives us two remainders of 5, and we multiply them to get 25. 2+5=7. The nines remainder of 729 must also be 7, or we have the wrong root.
7+2+9=18, 1+8=9, and we can cast out the 9, giving us a remainder of zero. Seven does not equal zero, so 23 is not the correct root, and that leaves 27 as the only possible answer.

[Ohio_Renz]

Square root of 3364 is 58. Because find the square of 4. Choose either 2 or 8. Cancel 2 digits. Square Root of 33 is Irriational Number. But remove the decimals. Then the answer is 58

[alphaadarsh]

3:46 in this section why didn't you multiple 5×4