Expressing sin(x) and cos(x) in terms of tan(x)

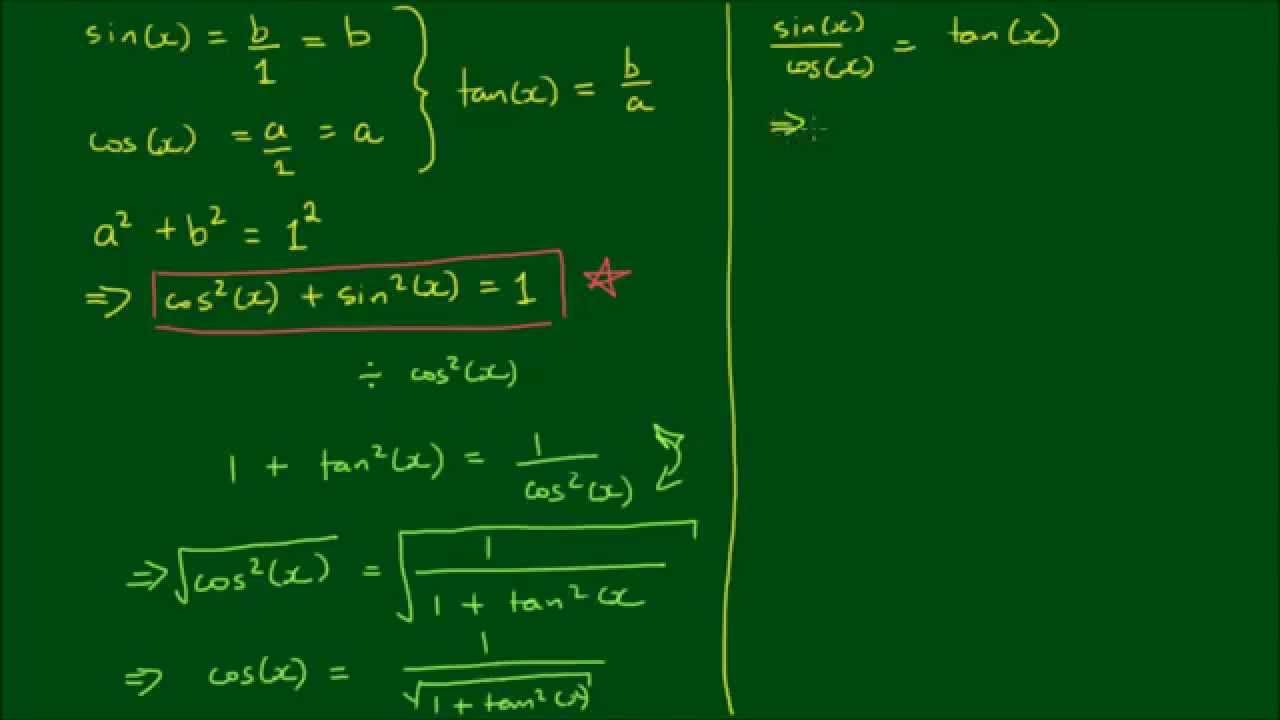
In this video, I show how with a right angled triangle with hypotenuse 1, sides (a) and (b), and using Pythagoras' Theorem, that:

cos(x) = 1 / sqrt( 1 + tan^2(x)) and
sin(x) = tan(x) / sqrt( 1 + tan^2(x))

From the Pythagorean Identity: cos^2(x) + sin^2(x) = 1. If we divide this by cos^2x, we get...

1 + tan^2(x) = 1/cos^2(x)

And if we flip this over, we get...

cos^2(x) = 1/[1 + tan^2(x)]

And taking the square root of both sides...

cos(x) = 1/√[1 + tan^2(x)]

For sin(x), we note that sin(x)/cos(x) = tan(x), which we can rearrange to sin(x) = tan(x)cos(x). And thus...

sin(x) = tan(x)/√[1 + tan^2(x)]

These are useful trigonometric identities that can help us when performing integrals of trig functions.

Thanks for watching. Please give me a "thumbs up" if you have found this video helpful.

Please ask me a maths question by commenting below and I will try to help you in future videos.

[MasterWuMathematics]

the integral of cos(a/x) is one of those integrals that cannot be expressed as an elementary function, so it can't be "solved" in a sense. Let me do some more study on this topic and I'll make a video in the near future

[ejphilipsumatra]

2:27 I don't get the "if i flip this over" part, i need more explanation as i simply cannot just flip it over in my exam papers and expect my prof to understand😢

[IndianGamer-qzlf]

Thank You very much, sir. you have helped me a lot.

[emmanuelalbazi]

Thank you so much.
I love your channel.

[joaquingutierrez]

he forgot to add the plus or minus when he took the square roots

[emmanuelalbazi]

Can you solve the integral of cos(a/x)

[flirt]

How can i Express tanxsecx in terms of cosx

[R.d.onlysportid]

Thanks 😊 for the explanation it help me so much

[ZORO-dkkk]

Plz tell tan A in terms lf skn A can be expressed

[Triplechoco]

So what exactly are we trying to do? Just plug in Trigonometric identities until we get the terms of the Trig. Function we want? And then solve for the initial Trig. Funct, or are we supposed to solve for the Trig. Function we're to supposed to be expressing it for (tan(x))?