filmov
tv
Write an algebraic expression for cos(sin^-1 x), cosine of inverse sine x

Показать описание
an algebraic expression for cos(sin^-1 x)
Check out my site & social media
Thank you for watching!
Please subscribe and share my videos to help my channel grow!
blackpenredpen
Check out my site & social media
Thank you for watching!
Please subscribe and share my videos to help my channel grow!
blackpenredpen
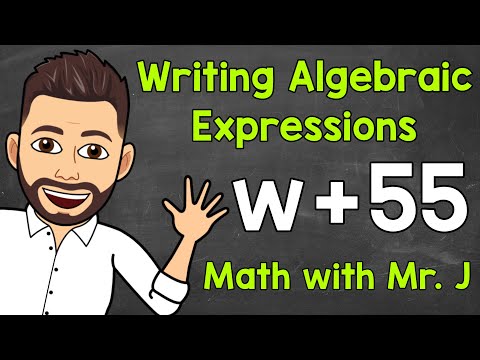
Writing Algebraic Expressions | Writing Expressions with Variables | Math with Mr. J
Algebraic Expressions (Basics)
Writing Algebraic Expressions (One Operation) | Math with Mr. J
How to Write Algebraic Expressions
How to write algebraic expressions from word problems | 6th grade | Khan Academy
Forming Algebraic Expressions - Corbettmaths
Algebra Basics: Solving 2-Step Equations - Math Antics
Writing Expressions
Fast SAT Math Wins 🔌 Plug-In and ✅ Succeed #maths #satmathtips #function #algebra #viralshorts
Write an algebraic expression for each of the following: (a) 3 subtracted from a number y....
Basic Mathematics & Algebra : How to Write an Algebraic Expression
Writing Algebraic Expressions (Two Operations) | Math with Mr. J
Translating Words To Algebraic Expressions Explained!
How to Write Algebraic Expressions
Writing Trig Functions as Algebraic Expressions
Learn how to write an algebraic equation from a verbal expression
TRANSLATING WORDS INTO ALGEBRAIC EXPRESSIONS!
How to write basic algebraic expressions from word problems | 6th grade | Khan Academy
Write Algebraic Expressions from Statements: Form ax+b and a(x+b)
Introduction to Algebra Song | Variables & Algebraic Expressions
How to Write Algebraic Expressions in Words and Phrases
10 - Math grade(6) 2024 | unit 3 lesson 3 [ Writing Algebraic Expressions ]
How to write expressions with variables | Introduction to algebra | Algebra I | Khan Academy
GCSE Maths - How to Simplify Algebraic Expressions #27
Комментарии
 0:12:52
0:12:52
 0:09:56
0:09:56
 0:06:41
0:06:41
 0:06:13
0:06:13
 0:04:41
0:04:41
 0:10:43
0:10:43
 0:10:29
0:10:29
 0:11:32
0:11:32
 0:00:40
0:00:40
 0:01:09
0:01:09
 0:02:04
0:02:04
 0:07:57
0:07:57
 0:10:43
0:10:43
 0:03:33
0:03:33
 0:03:55
0:03:55
 0:01:01
0:01:01
 0:08:18
0:08:18
 0:03:08
0:03:08
 0:02:34
0:02:34
 0:03:24
0:03:24
 0:05:42
0:05:42
 0:35:54
0:35:54
 0:01:38
0:01:38
 0:06:27
0:06:27