filmov
tv
How Was Fermat's Last Theorem Proved for Regular Primes?

Показать описание
Proving Fermat' s Last Theorem (almost) in just 2 minutes !
What is Fermat's Last Theorem?
Fermat's Last Theorem - Numberphile
Elliptic Curves and Modular Forms | The Proof of Fermat’s Last Theorem
Fermat's Last Theorem solved
How Was Fermat's Last Theorem Proved for Regular Primes?
Mathematicians explains Fermat's Last Theorem | Edward Frenkel and Lex Fridman
Unveiling the genius behind Fermat's Last Theorem: Andrew Wiles
Thirty years of proof: an interview with Andrew Wiles on the anniversary of Fermat's Last Theor...
The Heart of Fermat's Last Theorem - Numberphile
The Bridges to Fermat's Last Theorem - Numberphile
What Is Fermat's Last Theorem?
'I loved every minute of it, however hard it had been'
Andrew Wiles: Fermat's Last theorem: abelian and non-abelian approaches
A brief history of Fermat's Last Theorem #math #numbertheory #fermat #fermatslasttheorem #euler
What was Fermat’s “Marvelous' Proof? | Infinite Series
When did you know it was Maths? - Andrew Wiles
Proving weird Fermat's Last Theorem in just 2 minutes !
The math behind Fermat's Last Theorem | Modular Forms
FERMAT'S LAST THEOREM
The Man Who Solved the World’s Most Famous Math Problem
What is Fermat's Last Theorem?
How Andrew Wiles proved Fermat's Last Theorem
Andrew Wiles proving Fermat's Last Theorem | Po-Shen Loh and Lex Fridman
Комментарии
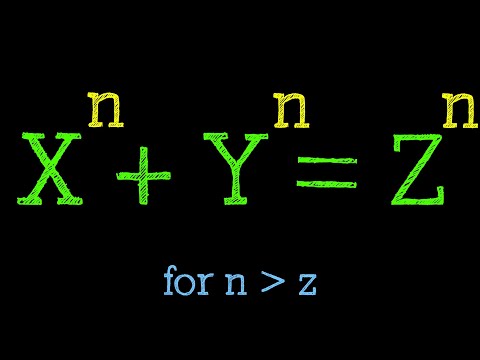 0:02:00
0:02:00
 0:01:44
0:01:44
 0:09:31
0:09:31
 0:10:14
0:10:14
 0:01:09
0:01:09
 0:17:05
0:17:05
 0:15:14
0:15:14
 0:02:34
0:02:34
 0:02:02
0:02:02
 0:09:28
0:09:28
 0:27:53
0:27:53
 0:00:55
0:00:55
 0:03:31
0:03:31
 0:53:12
0:53:12
 0:00:48
0:00:48
 0:14:40
0:14:40
 0:00:49
0:00:49
 0:02:00
0:02:00
 0:14:37
0:14:37
 0:00:18
0:00:18
 0:11:14
0:11:14
 0:07:06
0:07:06
 0:01:00
0:01:00
 0:04:26
0:04:26