filmov
tv
The math behind Fermat's Last Theorem | Modular Forms

Показать описание
The fascinating piece of math hidden behind the proof of Fermat's last Theorem for most people.
===Chapters===
0:00 Introduction
1:14 Lattices
4:05 Modular Forms
7:26 The Modular Group
10:46 Fourier Series
13:14 How many are there
13:50 Outro
===Made with===
===Music===
Music by Vincent Rubinetti
Download the music on Bandcamp:
Stream the music on Spotify:
===Tags===
#modularforms #fermat #modularity
===Chapters===
0:00 Introduction
1:14 Lattices
4:05 Modular Forms
7:26 The Modular Group
10:46 Fourier Series
13:14 How many are there
13:50 Outro
===Made with===
===Music===
Music by Vincent Rubinetti
Download the music on Bandcamp:
Stream the music on Spotify:
===Tags===
#modularforms #fermat #modularity
Proving Fermat' s Last Theorem (almost) in just 2 minutes !
Fermat's Last Theorem - Numberphile
The math behind Fermat's Last Theorem | Modular Forms
What is Fermat's Last Theorem?
Elliptic Curves and Modular Forms | The Proof of Fermat’s Last Theorem
Mathematicians explains Fermat's Last Theorem | Edward Frenkel and Lex Fridman
The Bridges to Fermat's Last Theorem - Numberphile
A brief history of Fermat's Last Theorem #math #numbertheory #fermat #fermatslasttheorem #euler
Fermat's Last Theorem solved
How Was Fermat's Last Theorem Proved for Regular Primes?
Fermat's Last Theorem
The Heart of Fermat's Last Theorem - Numberphile
Andrew Wiles: The Dreamer Behind Fermat's Last Theorem
HISTORY OF MATH - Pierre de Fermat 's Contributions #letmarch2023 #mathmajorship
Fermat's Last Theorem: A Mathematical Enigma #maths
The Biggest Project in Modern Mathematics
Pingu reacting to proof of Fermat's Last Theorem
Fermat's Last Theorem Final Solution-Explained
Pierre Fermat when asked whether he really had a proof of his last theorem.
Ramanujan, 1729 and Fermat's Last Theorem
Fermat's Last Theorem - Analyze
How did Fermat Inspire Newton? #shorts
Who proved Fermat's Last Theorem?
The theorem that has puzzled world's greatest minds for three centuries - Fermat's Last Th...
Комментарии
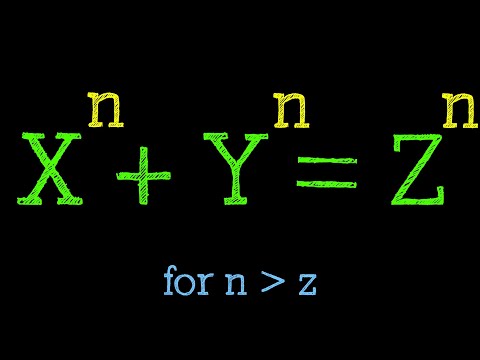 0:02:00
0:02:00
 0:09:31
0:09:31
 0:14:37
0:14:37
 0:01:44
0:01:44
 0:10:14
0:10:14
 0:15:14
0:15:14
 0:27:53
0:27:53
 0:00:48
0:00:48
 0:01:09
0:01:09
 0:17:05
0:17:05
 0:00:48
0:00:48
 0:09:28
0:09:28
 0:00:11
0:00:11
 0:01:01
0:01:01
 0:00:07
0:00:07
 0:13:19
0:13:19
 0:00:10
0:00:10
 0:00:31
0:00:31
 0:00:23
0:00:23
 0:16:48
0:16:48
 0:00:51
0:00:51
 0:00:20
0:00:20
 0:00:15
0:00:15
 0:00:58
0:00:58