filmov
tv
What was Fermat’s “Marvelous' Proof? | Infinite Series

Показать описание
If Fermat had a little more room in his margin, what proof would he have written there?
Tweet at us! @pbsinfinite
Email us! pbsinfiniteseries [at] gmail [dot] com
Resources:
Contemporary Abstract Algebra by Joseph Gallian
Standard Definitions in Ring Theory by Keith Conrad
Rings and First Examples (online course by Prof. Matthew Salomone)
Fermat's Enigma by Simon Singh
Who was first to differentiate between prime and irreducible elements? (StackExchange)
Previous Episodes:
What Does It Mean to be a Number?
What are Numbers Made of?
Gabe's references from the comments:
Blog post about the Peano axioms and construction of natural numbers by Robert Low:
Recommended by a viewer for connections to formulation of numbers in computer science:
In 1637, Pierre de Fermat claimed to have the proof to his famous conjecture, but, as the story goes, it was too large to write in the margin of his book. Yet even after Andrew Wiles’s proof more than 300 years later, we’re still left wondering: what proof did Fermat have in mind?
The mystery surrounding Fermat’s last theorem may have to do with the way we understand prime numbers. You all know what prime numbers are. An integer greater than 1 is called prime if it has exactly two factors: 1 and itself. In other words, p is prime if whenever you write p as a product of two integers, then one of those integers turns out to be 1. In fact, this definition works for negative integers, too. We simply incorporate -1. But the prime numbers satisfy another definition that maybe you haven’t thought about: An integer p is prime if, whenever p divides a product of two integers, then p divides at least one of those two integers.
Written and Hosted by Tai-Danae Bradley
Produced by Rusty Ward
Graphics by Ray Lux
Assistant Editing and Sound Design by Mike Petrow and Linda Huang
Special thanks to Roman Pinchuk for supporting us on our Converse level on Patreon.
Along with thanks to Matthew O'Connor, Yana Chernobilsky, and John Hoffman who are supporting us on Patreon at the Identity level!
And thanks to Mauricio Pacheco who are supporting us at the Lemma level!
Комментарии
 0:14:40
0:14:40
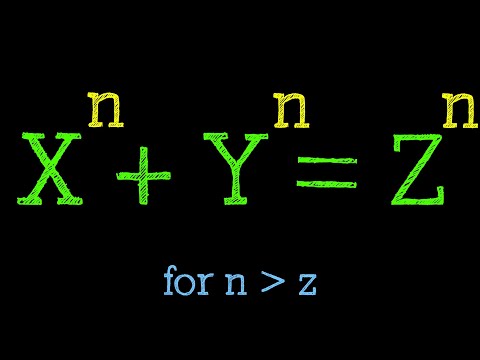 0:02:00
0:02:00
 0:01:44
0:01:44
 0:09:31
0:09:31
 0:54:26
0:54:26
 0:01:09
0:01:09
 0:00:48
0:00:48
 0:07:06
0:07:06
 0:01:01
0:01:01
 0:10:14
0:10:14
 0:05:00
0:05:00
 0:15:14
0:15:14
 0:03:21
0:03:21
 0:17:05
0:17:05
 0:11:14
0:11:14
 0:09:28
0:09:28
 0:02:00
0:02:00
 0:04:26
0:04:26
 0:02:02
0:02:02
 0:01:02
0:01:02
 0:16:07
0:16:07
 0:00:31
0:00:31
 0:02:42
0:02:42
 0:53:12
0:53:12