filmov
tv
Math Dude 016 • Video Extra! • The Fibonacci Sequence...and Rabbits!
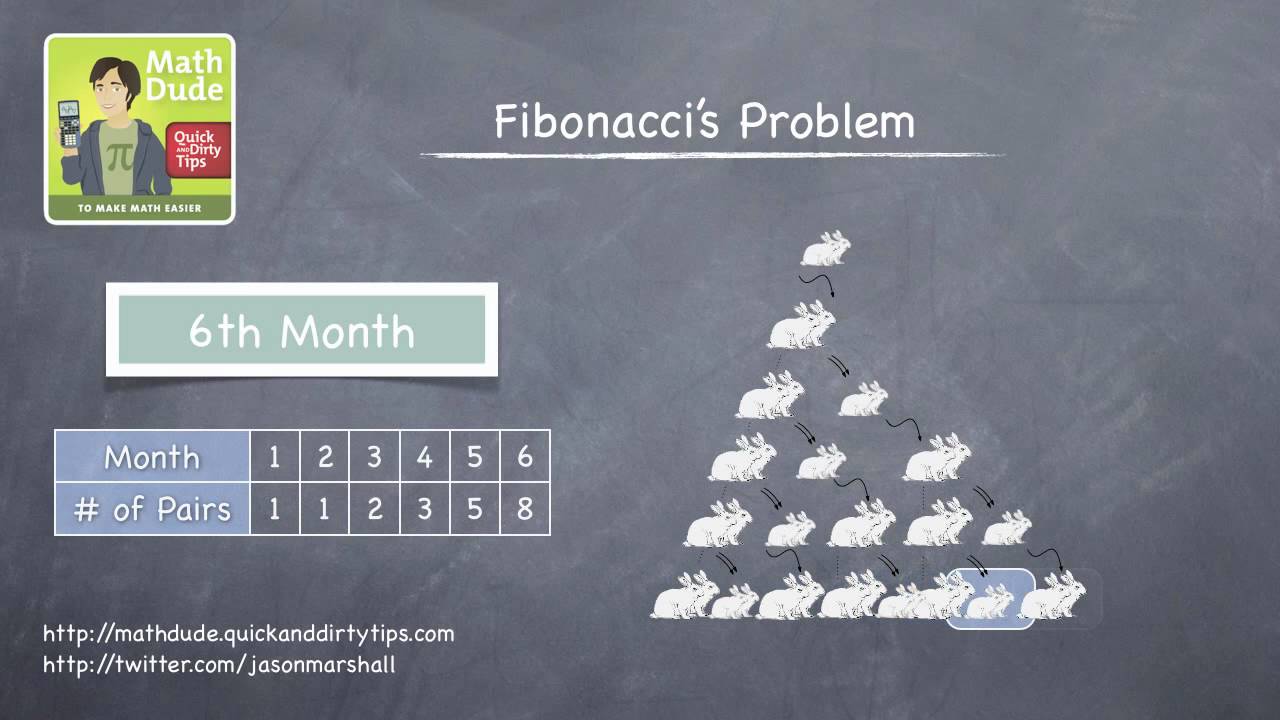
Показать описание
Find out how to solve Fibonacci's problem about rabbit populations and derive the Fibonacci sequence in this appendix to Episode 16 of the Math Dude's Quick and Dirty Tips podcast.
Math Dude 016 • Video Extra! • The Fibonacci Sequence...and Rabbits!
Math Dude 017 • Video Extra! • What is the Golden Ratio?
The Fibonacci Sequence and Rabbits with Math Dude, Jason Marshall
Learning Math
Human Calculator Solves World’s Longest Math Problem #shorts
Math Dude 018 • Video Extra! • The Golden Spiral and the Rule of Thirds
Math Dude 015 • Video Extra! • Can Populations Grow Exponentially?
Baldi's Basic Math POV Version (Animation Meme) #memeanimation #baldisbasics @dude.dans_
my 13 year old iPad 2 (2011) still runs one app pretty well. YouTube kids app!
Amazing Math Hacks
WHY I HATE MATH 😭 #Shorts
Math trick for multiplying?
Animation vs. Math
Math Dude 010 • Video Extra! • Why Can't the Denominator of a Fraction Be Zero?
What Is a Geometric Sequence? with Math Dude, Jason Marshall
Did you know this Fraction Tricks #math #mathematics #mathstricks #maths #mathhacks
Math Prof answers 6÷2(1+2) = ? once and for all ***Viral Math Problem***
The Most Wholesome Math Equation
What's the Exact Value of the Golden Ratio? with Math Dude, Jason Marshall
Math Dude 020 • Video Extra! • How to Do Long Division
The only SAT Math DESMOS Guide you'll ever need
Calculator Math Guy (Green Screen) MEME with sound
The Hardest Math Test
Solving Equations | Easy Methods to find X | Easy Algebra | Maths Shortcuts
Комментарии
 0:03:15
0:03:15
 0:02:26
0:02:26
 0:03:15
0:03:15
 0:02:37
0:02:37
 0:00:34
0:00:34
 0:01:49
0:01:49
 0:02:06
0:02:06
 0:00:09
0:00:09
 0:01:48
0:01:48
 0:00:16
0:00:16
 0:00:24
0:00:24
 0:00:16
0:00:16
 0:14:03
0:14:03
 0:02:32
0:02:32
 0:02:06
0:02:06
 0:01:00
0:01:00
 0:06:04
0:06:04
 0:00:16
0:00:16
 0:02:26
0:02:26
 0:02:51
0:02:51
 0:17:04
0:17:04
 0:00:15
0:00:15
 0:00:28
0:00:28
 0:00:10
0:00:10