filmov
tv
Divergence Theorem

Показать описание
In this video, I present the divergence theorem, which is a natural generalization of the fundamental theorem of calculus in 3 dimensions. It says that the surface integral of a vector field F is the triple integral of the divergence of F, and it provides a neat way of calculating surface integrals. Enjoy!
Gauss Divergence Theorem. Get the DEEPEST Intuition.
The Divergence Theorem
Gauss's Divergence Theorem
The Divergence Theorem // Geometric Intuition & Statement // Vector Calculus
The Divergence Theorem, a visual explanation
Divergence Theorem
A unified view of Vector Calculus (Stoke's Theorem, Divergence Theorem & Green's Theor...
Divergence and curl: The language of Maxwell's equations, fluid flow, and more
Electrostatics potential energy II B.Sc NEP 2020 , LU 2025
16.9: The Divergence Theorem
3D divergence theorem intuition | Divergence theorem | Multivariable Calculus | Khan Academy
Divergence theorem example 1 | Divergence theorem | Multivariable Calculus | Khan Academy
Calculus 3: The Divergence Theorem (Video #35) | Math with Professor V
The Divergence Theorem - Part 1
Multivariable Calculus: Divergence Theorem - Examples (16.9)
Divergence Theorem
The Divergence Theorem
Gauss Divergence Theorem: Basics, Proof, Physical Significance and Applications
Divergence Theorem Example and Verification Explained
Divergence Theorem #calculus #physics
Divergence theorem proof (part 1) | Divergence theorem | Multivariable Calculus | Khan Academy
Surface And Flux Integrals, Parametric Surf., Divergence/Stoke's Theorem: Calculus 3 Lecture 15...
Example: Verifying the Divergence Theorem
Understanding the Divergence Theorem #shorts
Комментарии
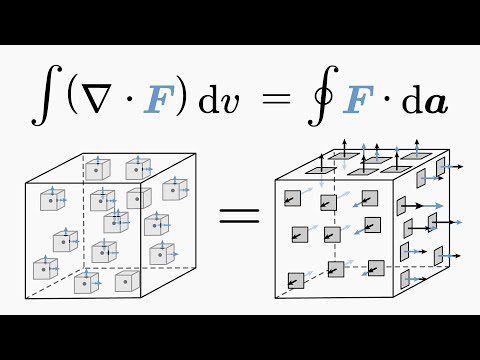 0:07:53
0:07:53
 0:06:31
0:06:31
 0:26:53
0:26:53
 0:07:35
0:07:35
 0:12:51
0:12:51
 0:10:42
0:10:42
 0:08:18
0:08:18
 0:15:42
0:15:42
 0:00:00
0:00:00
 0:24:10
0:24:10
 0:09:14
0:09:14
 0:10:45
0:10:45
 0:55:08
0:55:08
 0:06:11
0:06:11
 0:08:55
0:08:55
 0:11:55
0:11:55
 0:10:04
0:10:04
 0:12:49
0:12:49
 0:20:59
0:20:59
 0:00:50
0:00:50
 0:08:29
0:08:29
 3:31:35
3:31:35
 0:20:09
0:20:09
 0:00:40
0:00:40