The Divergence Theorem // Geometric Intuition & Statement // Vector Calculus

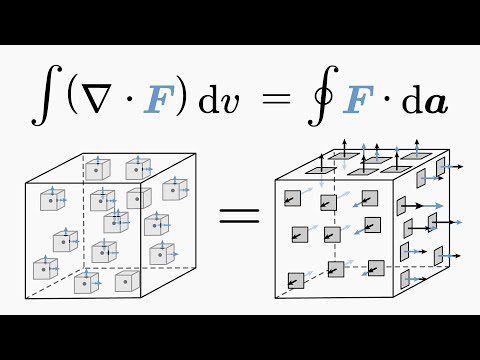
In this video we get to the last major theorem in our playlist on vector calculus: The Divergence Theorem. We've actually already seen the two-dimensional analog when we studied the Divergence or Flux form of Green's Theorem. Now we upgrade to the three-dimensional situation where we have a closed, smooth surface and a vector field. The question is, to what degree is there outwards flux of the vector field across this surface? The divergence theorem allows this global property to be compared to a triple integral over the enclosed volume of the divergence of the vector field; that is, adding up a local property of the divergence. Much like Stokes' Theorem before it, the divergence theorem is another example where integrating a differential operator along an entire region gives us information (in this case outward flux) on the boundary of that region.

0:00 Divergence of a Field
2:49 Recalling Green's Theorem
4:40 Divergence Theorem
6:20 Conditions

MY VECTOR CALCULUS PLAYLIST:

OTHER COURSE PLAYLISTS:

OTHER PLAYLISTS:
► Learning Math Series
►Cool Math Series:

BECOME A MEMBER:

MATH BOOKS & MERCH I LOVE:

SOCIALS:

[timondalton]

I find it rare to both understand an equation intuitively and how to calculate it after watching a video. You are raising the bar of education everywhere.

[DarinBrownSJDCMath]

Thanks for making all these public this weekend!

[tomatrix]

It’s amazing how these are just translations of 2d concepts into 3d. Great presentation!

[aidanbaxter]

your diagrams are great. understanding this so intuitively after your 7 minute video is incredible. what an awesome theorem!

[sarvasvkakkar]

A Really Big Thanks to you Sir for giving an amazing source to have a crystal clear understanding of concepts in multivariable calculus!

[structuralanalysis]

This is a brilliant content for visualization. Thank you so much for uploading these in youtube. God bless you. Keep up the good work.

[dhruvxx]

it was so easy, but even tho our prof is great i didn't got the concept, vector calculus is best with all these animations, really thanks for all these

[latestjobsupdates]

@2:41 correction : it takes a vector function and spits out a scaler function.

[nippletonuniversity]

Now this is the stuff I should be covering. I approve

[Matthias]

I love your videos. They inspire me to seek out explanations for all kinds of math. Thank you for being such a great teacher.

[zexisun]

Thank you so much, my classes are lacking of these geometric interpretations, now I am a lot clear about the topic

[nasimhossain]

I love every second of your explanation <3

[carmelpule]

Congratulations for your videos,

I am now a very old man and when I was younger about 65 years ago, I tried to clarify in my mind the different activities that the few derivatives and associated integrals, contribute to the following set of particular "activities/ functions" they create I could see all this as an Engineering function in my own mind, but never drew my concepts on papers. They seem to have the same building blocks.
1. Cauchy Riemann relations
2. The Grad operator.
3. The curl operator.
4. the Divergence operator.
5 . Green's Curl theorems of circulation
6. Green's Divergent theorem of flux
7. Stoke's Curl theorem involving circulation
8. Divergence theorem involving divergences through volumes,
I always thought that students should see the close links there are in how these derivatives are combined to produce their " engineered" activity.

dU/dx dU/dy dU/dz
dV/dx dV/dy dV/dz
dZ/dx dZ/dy dZ/dz and reduced to two dimensions
dU/dx dU/dy
dV/dx dV/dy

[JB-jiyq]

Very interesting, all so liked what was behind you as well.

[sethbeckett]

Yo thank you for this video (and the Stoke's Theorem one), super duper helpful!!

[swayamkumarpatro]

Thank you for this lecture series sir. I have my end semester exams now on Integration in Vector Fields and Multivariable Calculus. You have helped a lot!!!

[ahmadawlagi]

shouldn't the left-hand side of the divergence theorem have d sigma instead of ds?
4:40

[zethayn]

You are amazing sir, thank you very much!

[sdsa]

thanks! Having studied both flux forms of the 2D green theorem, I was wondering why there was something missing in the 3D Kelvin-Stokes Theorem! it only has a 3D curly form, but finally, now found the 3D divergence form (its in the name duh!)

[davehumphreys]

Its been many years now, but I seem to recall that there are two 'things' about vector fields that, if known, tell us all there is to know about the field. One is where are the sources and sinks, if they exist, located within the field, and the other is where in the field are the spots that might cause a rotation of the field, located. Given the equation of the vector field, if you then run the divergence on it, using the dot product of nabla with the field equation, you will end up with a formula that contains 3 functions of x, y and z [ assuming Cartesian co-ordinates], that are then added algebraically. If you then enter any values of x, y and z into this formula the result with be a scaler number that is either +ve, -ve or zero. If its +ve, its telling you that at that xyz location, the field lines are diverging away from the point and that there is a source of the field there. If its -ve, its telling you that, at that location, the field lines are converging toward the point and that there is a sink of the field there. I find this idea easy to visualize by thinking about a static charge distribution and considering the electric field. If my divergence equation gives me a positive answer, then its telling me that at that xyz location there is a +ve charge ie a source of the field. This idea also explains why the divergence of the magnetic field is zero, since the field lines form closed loops and have no source or sink.
The curl of a vector field is, in my opinion, very similar to the angular momentum vector. The curl vectors are just lines you can draw to represent a rotation of the field. They also have no sources or sinks, which, again, explains why the divergence of the curl is zero.