filmov
tv
The Divergence Theorem, a visual explanation
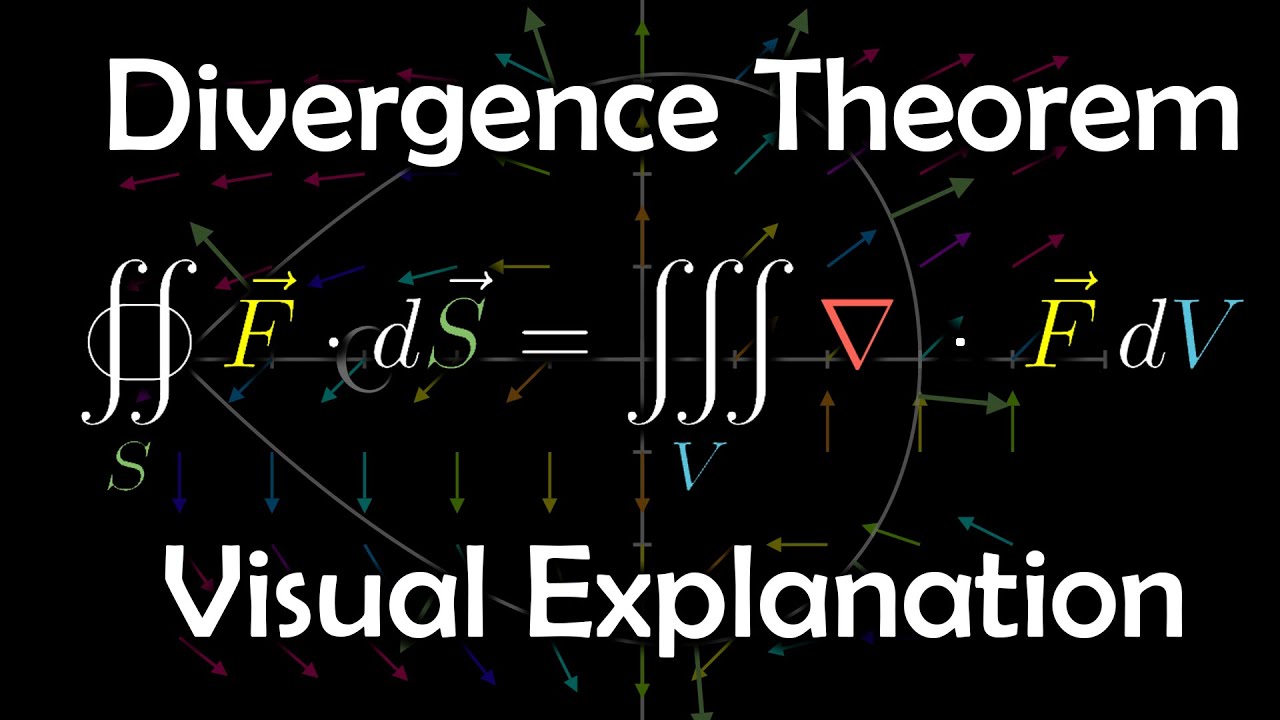
Показать описание
This video talks about the divergence theorem, one of the fundamental theorems of multivariable calculus. The divergence theorem relates a flux integral to a triple integral.
Follow Me!
Follow Me!
The Divergence Theorem, a visual explanation
The Divergence Theorem // Geometric Intuition & Statement // Vector Calculus
The Divergence Theorem
3D divergence theorem intuition | Divergence theorem | Multivariable Calculus | Khan Academy
Gauss Divergence Theorem. Get the DEEPEST Intuition.
Divergence and curl: The language of Maxwell's equations, fluid flow, and more
Multivariable Calculus: Divergence Theorem - Examples (16.9)
Intuition behind the Divergence Theorem (why it's true)
Calculus 3: The Divergence Theorem (Video #35) | Math with Professor V
Example of the divergence theorem
Multivariable calculus 4.7.5: Two-dimensional version of the divergence theorem
The Divergence Theorem - Examples I
The Divergence Theorem
Green's Theorem, explained visually
16.9: The Divergence Theorem
A unified view of Vector Calculus (Stoke's Theorem, Divergence Theorem & Green's Theor...
Example: Verifying the Divergence Theorem
Gauss's Divergence Theorem
Divergence Theorem #calculus #physics
Divergence Theorem
Physics - Advanced E&M: Ch 1 Math Concepts (21 of 55) The Divergence Theorem Example
The Divergence Theorem #6
Divergence Theorem
Multivariable Calculus | Verifying the Divergence Theorem
Комментарии
 0:12:51
0:12:51
 0:07:35
0:07:35
 0:06:31
0:06:31
 0:09:14
0:09:14
 0:07:53
0:07:53
 0:15:42
0:15:42
 0:08:55
0:08:55
 0:09:51
0:09:51
 0:55:08
0:55:08
 0:09:11
0:09:11
 0:08:24
0:08:24
 0:10:11
0:10:11
 0:10:04
0:10:04
 0:06:32
0:06:32
 0:24:10
0:24:10
 0:08:18
0:08:18
 0:20:09
0:20:09
 0:26:53
0:26:53
 0:00:50
0:00:50
 0:11:55
0:11:55
 0:06:53
0:06:53
 0:03:14
0:03:14
 0:30:57
0:30:57
 0:20:41
0:20:41