Gauss's Divergence Theorem

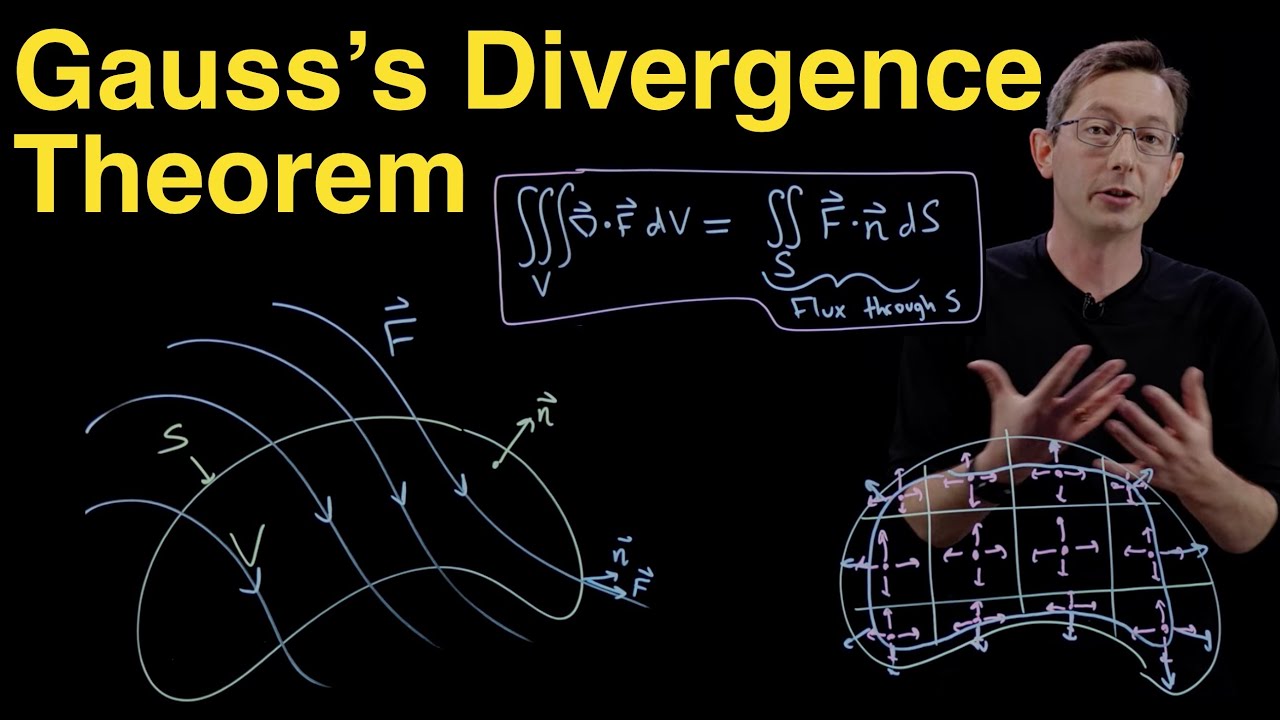
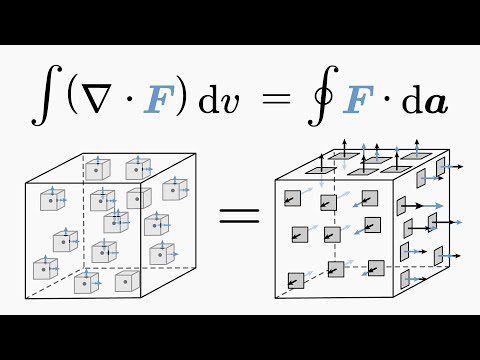
Gauss's Divergence theorem is one of the most powerful tools in all of mathematical physics. It is the primary building block of how we derive conservation laws from physics and translate them into partial differential equations.

@eigensteve on Twitter

This video was produced at the University of Washington

%%% CHAPTERS %%%
0:00 Introduction & Overview
8:55 Why Gauss's Theorem is True
14:38 Gauss's Theorem for PDEs: Mass Conservation
24:11 Recap

[theverner]

I am so much amazed how excited you are teaching this theorem. Wished to have teachers like you at uni too.

[francescogiuliano]

> I see a new video has been posted
>> I put "like"
>>> I watch the video

[StratosFair]

These lectures are fantastic, thank you for taking the time to produce and share them for free.

[zoteha]

Every math teacher feels it is his duty to say that he is not a fancy artist or so when he draws some kind of diagram

[joonasmakinen]

At 20:25 Steve oversimplified by moving the time derivative inside the volume integral just like that. It can only be done if the volume being integrated wont change over time, invalid assumption in fluid dynamics. Taking this into account leads us to another beautiful theorem called Reynolds Transport Theorem (RTT), which interestingly naturally leads to the right-hand-side on Steve’s board (if F is a velocity field).

[johnalley]

Outstanding lecture, professor. Defining first in words, providing an intuition and then releasing the math!

Shock and Awe.

Anyone can deliver the symbols. Gifted educators deliver intuition and genuine understanding.

[HarrydeBont]

Watching this video, I remembered being totally fascinated (for the first time in my life) by theoretical electrical theory. Thanks for the passion you bring presenting this math.

[hendriklohad]

It is thanksgiving eve and I am learning some quality vector calc from these lectures. They are so greatly made!! Every detail is explained and is wrapped so elegantly together. A joy to watch.

[wp]

Great video. Excellent for showing the intuition of the volume built as a union of smaller volumes, for divergence theorem. Just few comments:

- Divergence Thm has some assumptions. Broadly speaking, everything inside of the statement of the theorem must be meaningful, as an example if you write a divergence of F, that function F must be regular enough (differentiable) for the divergence to exist; REMEMBER that PDEs of Physics translate into "regular enough local regions" all the general Principles of Physics holding for all the physical systems, differentiable or not;
- Mass equation example:
> when you put time derivative inside the volume integral, you're doing right only if that volume does not change in time (i.e. you're implicitly considering a fixed control volume, otherwise that manipulation is WRONG). Anyway the conclusion you reach is right, but your derivation only holds for a steady volume for integration. Integral laws and then differential laws can be easily translated from the very statement of the Physical Principles if you firs consider Lagrangian volumes (i.e. those volumes moving with the continuum), and then transformed to fixed control volumes (some math required)
> maybe I missed that, but the physical meaning of F is not explicit here. In order to have the right physical dimension, it must have the dimension of a velocity. Indeed, it is the velocity field of the continuum under investigation in most of cases (few times it's a bit more tricky, i.e. in diffusion problems that vector field contains both a local average - averaged on the species velocity, maybe - velocity contribution and a drift velocity, likely due to gradient in the specie concentration, see Fick's law for diffusion).

Keep going. I'm very curious how this series evolves.

[lhliu]

Very excited to watch every update on this series！

[yashwanthcalidas]

Before this I had no idea fluid mechanics can be so intuitive and interesting. Great work sir, Thank you so much for your effort.

[iniyanmdr]

This is a treasure worth 1M views. I learnt this in my college days. Understood 15 years later.

[Martin-iwll]

Great lecture again, you are treasure for mankind!
I find the most interesting with the mass continuity equation is the physical interpretation that we can derive for div (F) by rewriting the continuity equation in terms of material derivatives

[AceBlockey]

Such great explanations and a highly quality channel. Great for building a strong intuition of concepts rarely explained in a straightforward manner.

[majorfallacy]

just came here to say that while i'm currently not watching most of your videos as you upload them, i'm still very thankful because i'm 99% certain that i'll need them again at some point in the future

[ChristAliveForevermore]

Great explanation! Gauss truly was a super genius for figuring this out.

[michelleelizabeth]

Life saving videos for students. Awesome. Thank you so much.

[bendavis]

What an outstanding explanation! I'm so surprised that my Calc textbook left out the Mass Continuity Equation when going over the Divergence Theorem. It's really motivating to hear how powerful this equation is in applied math and physics. I love hearing the real-world applications.

[CBMM_]

I'm so grateful for living at this time, so I can learn this theorem in 25 minutes.

[c.l.]

I'm so lucky to have discoverd your channel while self-learning multi-variable calc! Abosolutely recommend to anyone (even non-math majors who hasn't touched calculus in 4 years).