filmov
tv
Subspaces
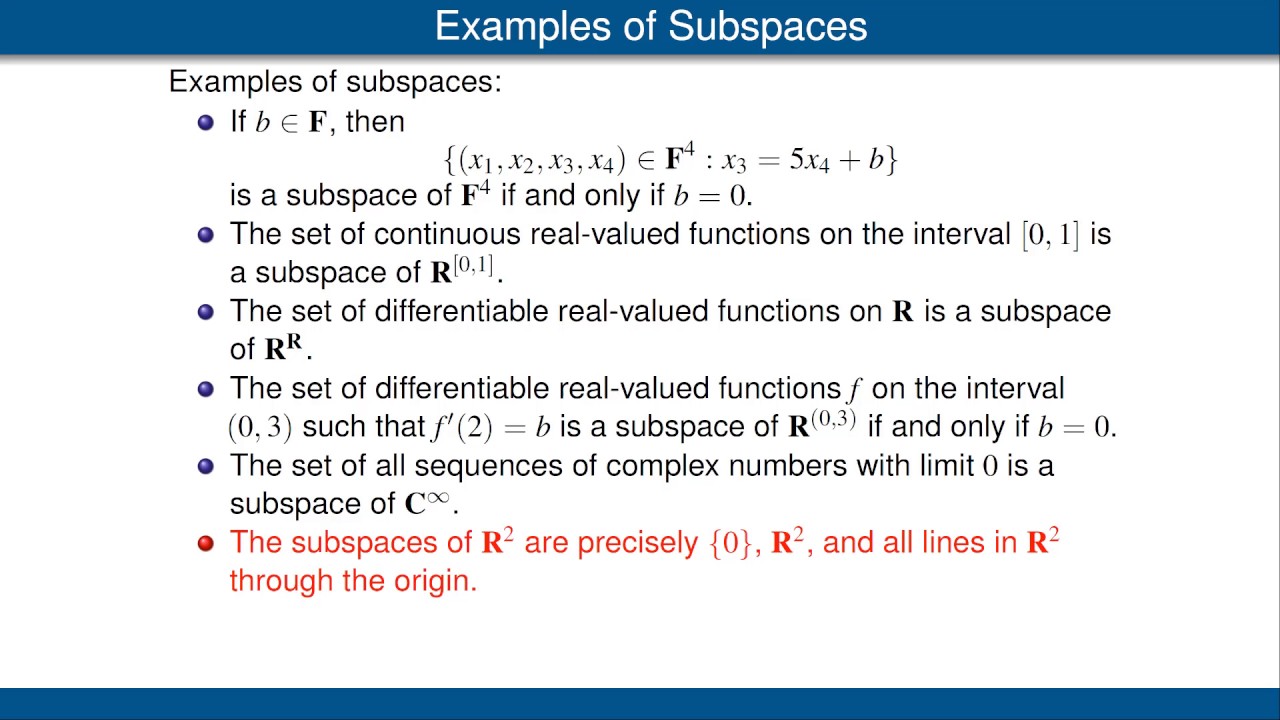
Показать описание
Subspaces of a vector space. Sums and direct sums.
Subspaces and Span
Linear subspaces | Vectors and spaces | Linear Algebra | Khan Academy
SUBSPACES - LINEAR ALGEBRA
Vector Subspaces
Subspaces are the Natural Subsets of Linear Algebra | Definition + First Examples
Linear Algebra 7 | Examples for Subspaces
Subspaces of Three Dimensional Space
Subspaces
4.1 Vector Spaces and Subspaces
Basis of a subspace | Vectors and spaces | Linear Algebra | Khan Academy
How to Prove a Set is a Subspace of a Vector Space
Linear Algebra Example Problems - Subspace Example #1
Understanding Vector Spaces
Linear Algebra - Lecture 28 - Subspaces
Linear combinations, span, and basis vectors | Chapter 2, Essence of linear algebra
Linear Algebra - Lecture 27 - Subspaces of R^n
Linear Algebra 6 | Linear Subspaces
What is a Subspace?
Linear Algebra 4.1.2 Subspace of a Vector Space
Advanced Linear Algebra 1: Vector Spaces & Subspaces
Subspaces of R^n
Linear Algebra - 13 - Checking a subspace EXAMPLE
4.2 - Subspaces (Part 1)
Vector Subspaces and Examples
Комментарии
 0:05:50
0:05:50
 0:23:29
0:23:29
 0:13:04
0:13:04
 0:08:46
0:08:46
 0:06:26
0:06:26
 0:10:56
0:10:56
 0:14:45
0:14:45
 0:10:12
0:10:12
 1:14:57
1:14:57
 0:19:00
0:19:00
 0:05:58
0:05:58
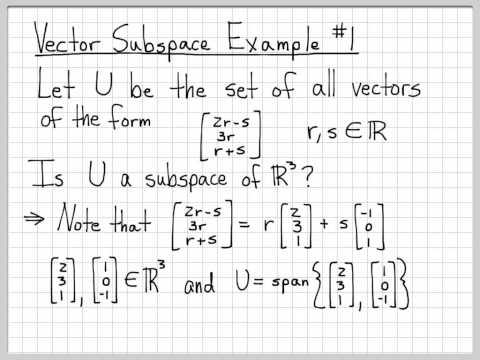 0:04:48
0:04:48
 0:08:41
0:08:41
 0:10:58
0:10:58
 0:09:59
0:09:59
 0:24:55
0:24:55
 0:09:34
0:09:34
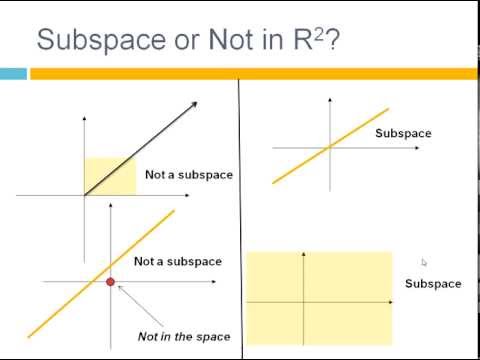 0:07:13
0:07:13
 0:17:58
0:17:58
 0:41:25
0:41:25
 0:09:33
0:09:33
 0:07:12
0:07:12
 0:27:24
0:27:24
 0:18:05
0:18:05