filmov
tv
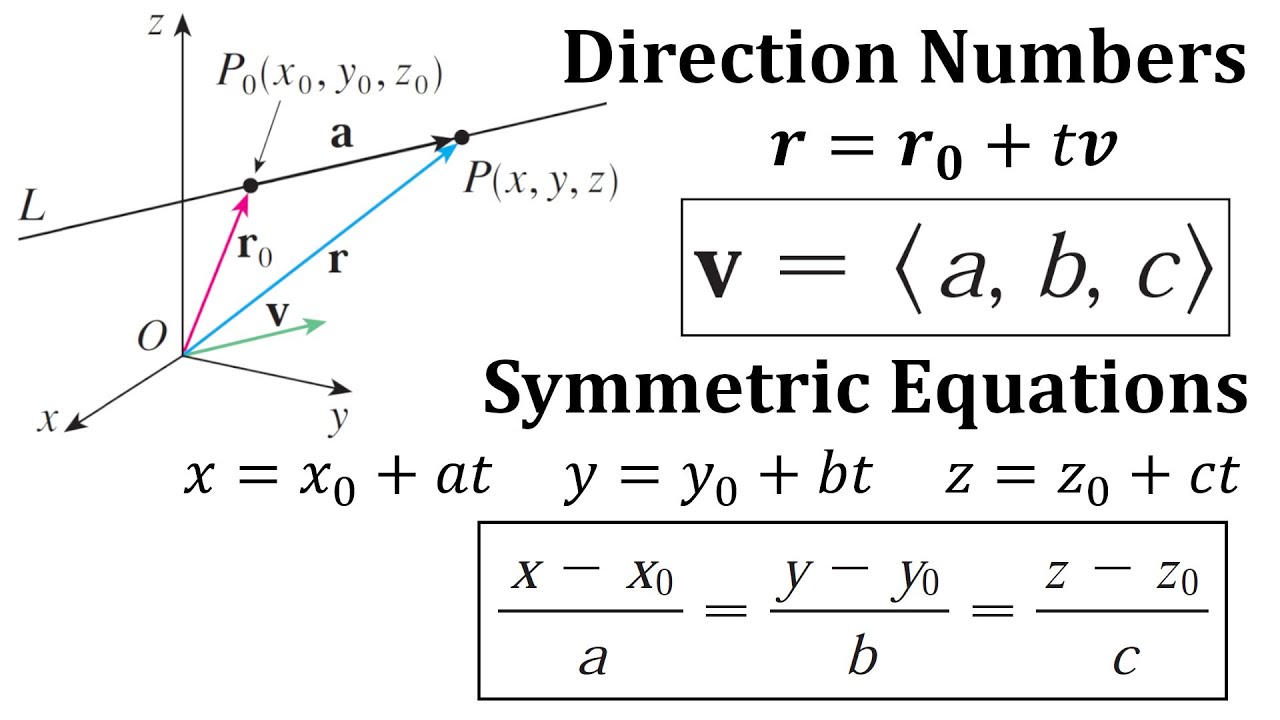
Direction Numbers and Symmetric Equations of a Line
Показать описание
In this video I go over what direction numbers and symmetric equations of a line are. Direction numbers are the components of the parallel vector in a vector equation of a line. We can use any three numbers that are proportional to the direction numbers and still get the same line. This is because the vector and parametric equations of a line are not unique.
The symmetric equations involve taking out the parameter t from each of the three parametric equations and then equating them to get equations that are symmetric, as the name implies. If one of the direction numbers is 0, then the symmetric equations can be simplified by having that coordinate equal to the corresponding point on the line.
The timestamps of key parts of the video are listed below:
- Direction Numbers: 0:00
- Symmetric Equations of a Line: 3:40
- Symmetric Equations if a Direction Number is Zero: 7:00
- Example 2: 9:21
- Solution to 2(a): 9:46
- Solution to 2(b): 15:34
- Note on Example 2: 20:30
This video was taken from my earlier video listed below:
Related videos:
------------------------------------------------------
The symmetric equations involve taking out the parameter t from each of the three parametric equations and then equating them to get equations that are symmetric, as the name implies. If one of the direction numbers is 0, then the symmetric equations can be simplified by having that coordinate equal to the corresponding point on the line.
The timestamps of key parts of the video are listed below:
- Direction Numbers: 0:00
- Symmetric Equations of a Line: 3:40
- Symmetric Equations if a Direction Number is Zero: 7:00
- Example 2: 9:21
- Solution to 2(a): 9:46
- Solution to 2(b): 15:34
- Note on Example 2: 20:30
This video was taken from my earlier video listed below:
Related videos:
------------------------------------------------------
0:22:17
Direction Numbers and Symmetric Equations of a Line
0:11:37
How To Find The Vector Equation of a Line and Symmetric & Parametric Equations
0:24:50
🔶09 - Vector, Parametric and Symmetric Equations of a line in a 3D-plane: Ex1 and Ex2
0:05:18
Given Two Points on a Line Find the Parametric and Symmetric Equations
0:08:44
Parametric and symmetric equations of the line (KristaKingMath)
0:04:58
How to Find Parametric and Symmetric Equations for a Line Given Another Parallel Line
0:05:48
Vectors - Vector, Parametric and Symmetric Equations of Lines
0:05:43
Write Vector and Symmetric Equation of Lines Q6
0:02:29
Write Symmetric Equation Q11
0:05:18
How to Write Vector Line Equation in Symmetric Form with zero direction number
0:02:38
Parametric and Symmetric Equations given a Point and a Parallel Vector
0:05:17
Vector Equation, Parametric Equations and Symmetric Equation Passing Through Two Points (3D)
0:10:49
Symmetric equations for the line of intersection of two planes (KristaKingMath)
0:37:08
An introduction to Parametric and symmetric equations
0:47:57
Calculus 3: Equations of Lines and Planes (Video #5) | Math with Professor V
0:03:25
How to Write Symmetric Equation in Vector Form
0:03:03
Symmetric Equation Q10
0:05:03
Determine Vector, Parametric and Symmetric Equation of Line from Two Points
0:26:03
Vectors 8.3 Vector, Parametric and Symmetric equations of lines in R3
0:11:39
Writing VECTOR, PARAMETRIC & SYMMETRIC Equations For Lines (1)
0:09:39
Finding a Point Symmetric with Respect to a Line, 2 METHODS
0:11:38
Vector, Parametric, Symmetric Equations - Lines in 3D
0:04:12
Symmetric Equation Given Direction Angles Q13
0:11:52
Calculus III: Equations of Lines and Planes (Level 2) | Vector, Parametric, and Symmetric Equations
Комментарии