filmov
tv
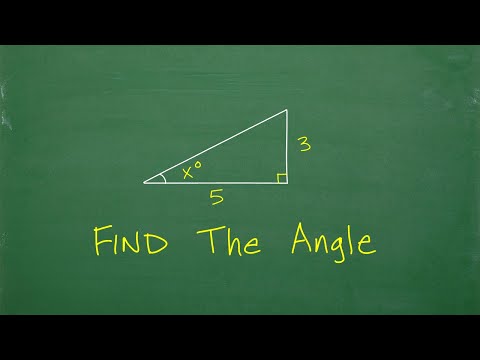
Can You Find Angle X? | Geometry Challenge!

Показать описание
Can You Find Angle X? | Geometry Challenge!
Can you Find Angle X? Step-by-step Explanation
Can You Find Angle X? | Quick & Simple Explanation
Can you find Angle X in this given shape? | Step-by-Step Tutorial
Challenge Question: Can you find the Angle X?| Step-by-Step Explanation
Can you find the angle X? | Two isosceles triangles | [Step-by-step explanation]
Can you find Angle X in the Cyclic Quadrilateral? | Important Geometry skills explained
Find the angle X | How to Solve this Tricky Geometry problem Quickly
Lines and Angles - Exercise 6.1 (Que. 1 to 4) | Class 9 Maths Chapter 6 | CBSE 2024-25
Hard Problem | Find Angle x | PRMO RMO INMO IMO Math Olympiads SSC
Find the Value of Angle X in this Triangle | Fast & Easy Tutorial
Justify your answer | Can you find the angle X? | #math #maths | #geometry
How do you find the angle? Let’s see…
No Calculators! | Can you find the angle X? | (Pythagorean Theorem) | #math #maths #geometry
Missing Angles in Triangles | How to Find the Missing Angle of a Triangle Step by Step
How to Find Angle x in a 5-Pointed Regular Star Using Three Easy Methods
Can you find the angle X? | Think outside the box | #math #maths #geometry
Math Olympiad | Find angle X in the triangle | Important Geometry skills explained step by step
Can You Find the Angle X in Triangle ABC? | Fast & Easy Tutorial
Finding the Missing Angle of a Triangle: A Step-by-Step Guide | Triangles | Math with Mr. J
Have you tried this technique? | Find the angle X | (Two isosceles triangles in a right triangle!!!)
Calculate angle X in a 5-sided irregular Polygon | Learn how to Solve this Geometry problem Quickly
Can you find the angle X? | (Trigonometry Training) | #math #maths #geometry #trigonometry
Can you find the angle X? | Two Methods | (Step-by-step explanation) | #math #maths #geometry
Комментарии
 0:08:44
0:08:44
 0:04:54
0:04:54
 0:05:12
0:05:12
 0:04:01
0:04:01
 0:08:05
0:08:05
 0:04:02
0:04:02
 0:04:59
0:04:59
 0:06:42
0:06:42
 0:20:06
0:20:06
 0:05:13
0:05:13
 0:04:53
0:04:53
 0:09:28
0:09:28
 0:15:46
0:15:46
 0:08:46
0:08:46
 0:03:40
0:03:40
 0:08:45
0:08:45
 0:08:52
0:08:52
 0:10:03
0:10:03
 0:08:02
0:08:02
 0:05:14
0:05:14
 0:08:04
0:08:04
 0:03:17
0:03:17
 0:10:52
0:10:52
 0:11:12
0:11:12