filmov
tv
An Infinite Sum of Reciprocals of Odd Squares
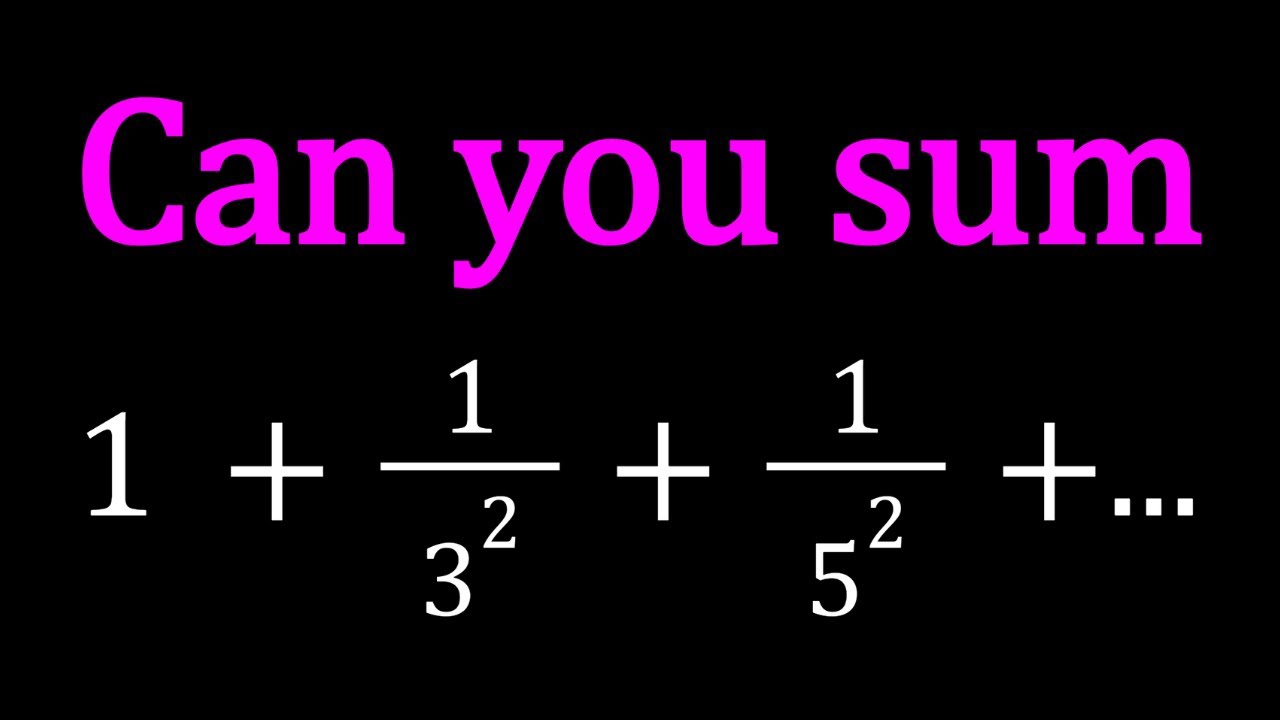
Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (aplusbi)
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
When you purchase something from here, I will make a small percentage of commission that helps me continue making videos for you.
If you are preparing for Math Competitions and Math Olympiads, then this is the page for you!
CHECK IT OUT!!! ❤️ ❤️ ❤️
If you need to post a picture of your solution or idea:
#radicals #radicalequations #algebra #calculus #differentialequations #polynomials #prealgebra #polynomialequations #numbertheory #diophantineequations #comparingnumbers #trigonometry #trigonometricequations #complexnumbers #math #mathcompetition #olympiad #matholympiad #mathematics #sybermath #aplusbi #shortsofsyber #iit #iitjee #iitjeepreparation #iitjeemaths #exponentialequations #exponents #exponential #exponent #systemsofequations #systems
#functionalequations #functions #function #maths #counting #sequencesandseries
#algebra #numbertheory #geometry #calculus #counting #mathcontests #mathcompetitions
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
When you purchase something from here, I will make a small percentage of commission that helps me continue making videos for you.
If you are preparing for Math Competitions and Math Olympiads, then this is the page for you!
CHECK IT OUT!!! ❤️ ❤️ ❤️
If you need to post a picture of your solution or idea:
#radicals #radicalequations #algebra #calculus #differentialequations #polynomials #prealgebra #polynomialequations #numbertheory #diophantineequations #comparingnumbers #trigonometry #trigonometricequations #complexnumbers #math #mathcompetition #olympiad #matholympiad #mathematics #sybermath #aplusbi #shortsofsyber #iit #iitjee #iitjeepreparation #iitjeemaths #exponentialequations #exponents #exponential #exponent #systemsofequations #systems
#functionalequations #functions #function #maths #counting #sequencesandseries
#algebra #numbertheory #geometry #calculus #counting #mathcontests #mathcompetitions
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
The Reciprocal Prime Series (this proof should be taught in calculus!)
An Infinite Sum of Reciprocals of Odd Squares
An Infinite Sum With Reciprocals
The sum of the reciprocals of all primes diverges | #some2
700 years of secrets of the Sum of Sums (paradoxical harmonic series)
An Infinite Sum With Reciprocals
Why is pi here? And why is it squared? A geometric answer to the Basel problem
If I did this in 1734 I'd be World Famous
Infinite Sum of Squared Reciprocals Proof
EASIEST proof that the sum of the reciprocals of the primes diverges!
Sum the series of reciprocal squares of the integers - Euler method
Infinite series: summing reciprocals of triangular numbers (visual proof)
When mathematicians get bored (ep1)
An Interesting Sum With Reciprocals of Factorials
Summing The Reciprocals of Sums
The Reciprocals of Primes - Numberphile
A Nice Property of Sums of Reciprocals
This is SERIESLY NUTS! Deriving the Sum of Reciprocals of the Odd Numbers Squared using INTEGRALS!
Sum of Reciprocals of Terms of a Sequence
The sum of the reciprocals of the squares
GMAT Sum of Reciprocals Questions -- the Quick and Easy Way
How to find Sum of Reciprocals of given numbers and their product
An Interesting Sum With Reciprocals
The Sum of All Prime Twins
Комментарии
 0:11:44
0:11:44
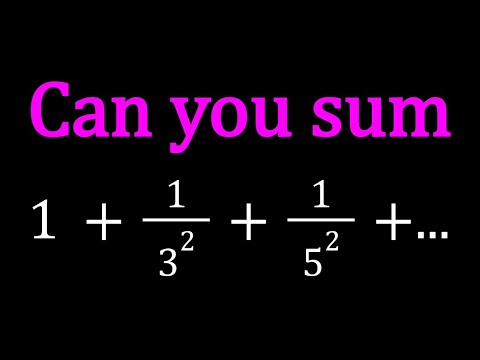 0:08:08
0:08:08
 0:10:05
0:10:05
 0:07:34
0:07:34
 0:46:34
0:46:34
 0:12:02
0:12:02
 0:17:08
0:17:08
 0:03:57
0:03:57
 0:08:52
0:08:52
 0:09:37
0:09:37
 0:14:06
0:14:06
 0:03:13
0:03:13
 0:00:37
0:00:37
 0:10:11
0:10:11
 0:09:47
0:09:47
 0:15:31
0:15:31
 0:07:29
0:07:29
 0:12:54
0:12:54
 0:07:50
0:07:50
 0:01:51
0:01:51
 0:01:44
0:01:44
 0:03:44
0:03:44
 0:09:15
0:09:15
 0:05:05
0:05:05