The Sum of All Prime Twins

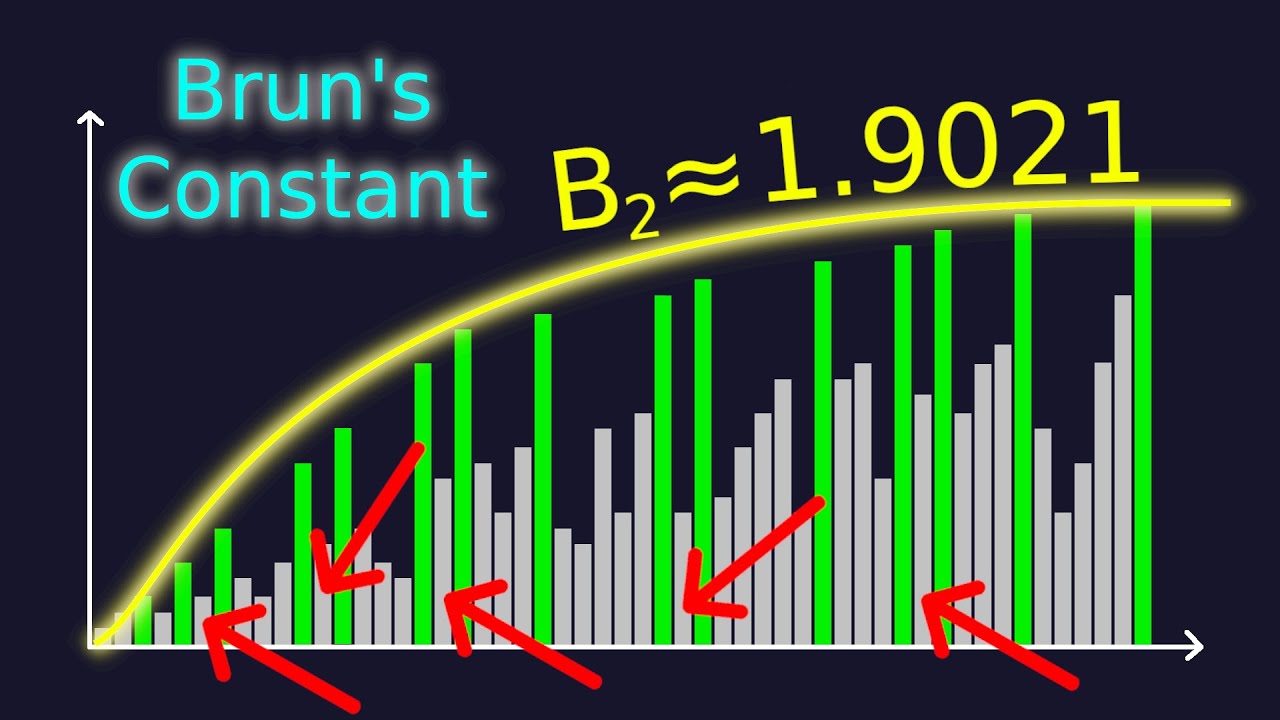
In number theory, Brun's theorem states that the sum of the reciprocals of the twin primes (pairs of prime numbers which differ by 2) converges to a finite value known as Brun's constant. Brun's theorem was proved by Viggo Brun in 1919, and it has historical importance in the introduction of sieve methods.

[notu]

This channel deserves more recognition, keep it up!

[cara-seyun]

It would be pretty freaky if Brun’s Constant is a rational number

[diniaadil]

In the twin prime series, do we duplicate primes that share two twin prime pairs ?
Ie, should the sum be 1/3 +1/5 +1/7 +... or 1/3+1/5+1/5+1/7+... ?

[whhitryjekugitihg]

this reminds me of the Goldbach conjecture!

[GerardCalvo]

Does B4 < B2 imply that the quadruplets of primes are less densely packed than twin primes amongst all natural numbers? E.g., does it mean that there are fewer quadruplets of primes than twin primes (which is obviously true, although both could be infinite)? Or, as another example, assume B'4 to be the (infinite?) sum of the reciprocals of consecutive primes that are separated by 4, or cousin primes: B'4 = Then, B'4 converges to approx 1.09. Thus B4 < B'4 < B2. Does this imply that there are fewer cousin primes than twin primes, and fewer quadruplets than cousin primes? Could anyone prove this statement or the opposite?

[codygamin]

This is great so i am commenting for the algorithm

[xCorvusx]

Isn't B_2 being irrational equivalent to there being infinitely many Twin prime pairs?
If there were finitely many, B_2 would be rational.

[williamzame]

This is wrong. If p is a prime > 3 then it is either p = 3n+1 for some n or p =3n+2 for some n. If p =3n+1 then p+2 =3n+3 is divisible by 3, so not prime. If p = 3n+2 then p+4 = 3n+6 is divisible by 3. So there are no prime triplets much less prime quadruplets.

[lol......................]

Im going to leave a comment for youtube algorithm

[activetutorial]

I can't fanum a 50 second video about steamed hams got more views than this.

[ShaunakDesaiPiano]

2:18 - here’s my take on why S₂(x) converges, using a fact from this guy’s video 6 Minutes of Fascinating Math:

For conciseness, let Hₙ be the harmonic series with all terms with denominator ending in the digit n removed.

The fact I’m referring to is that while the harmonic series diverges, H₉ converges. Of course, 9 is nothing special, as he himself said. Now consider the fact that S₂(x) is a series with absolutely no denominators ending in 0. By the previous fact, H₀. Obviously, S₂(x), on top of removing terms with denominator ending in 0, removes all composite denominators, aka removes more terms. Therefore, S₂ is less than H₀ and thus converges.

Does that make sense as an argument?