Derivative of ln(x) from First Principles

How to differentiate ln(x) from first principles

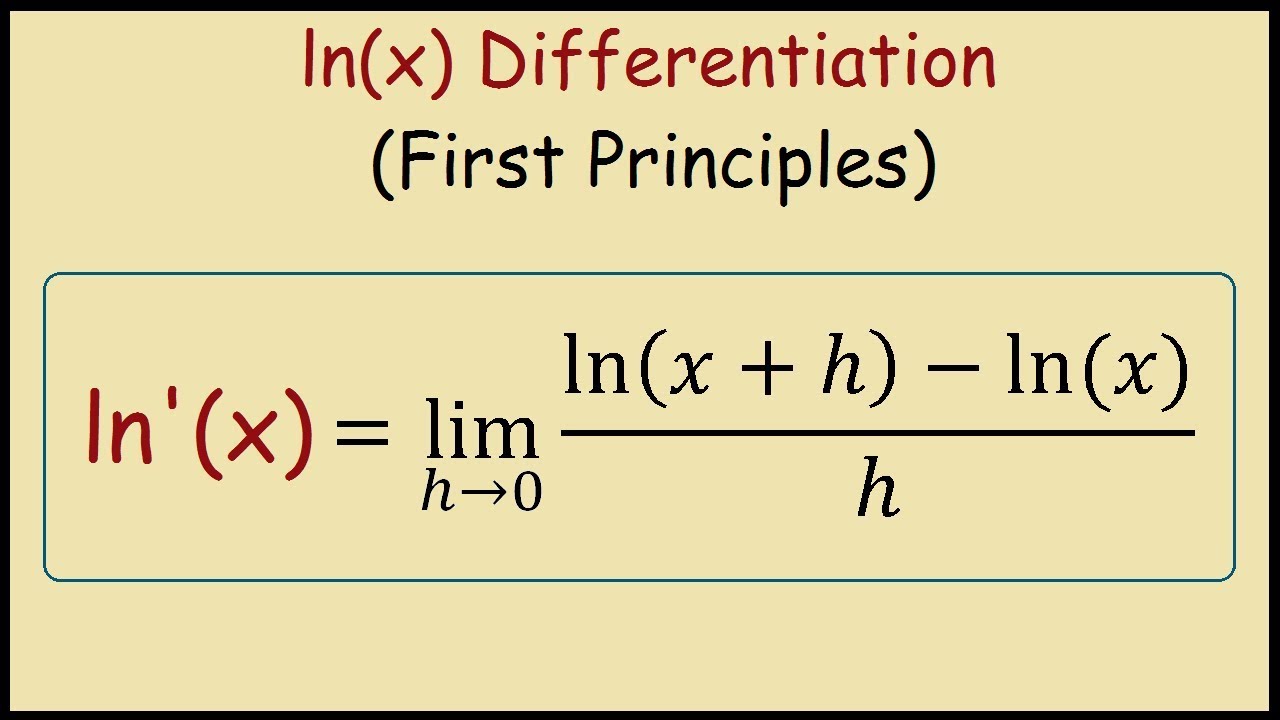
Begin the derivative of the natural log function by using the first principle definition and substituting f(x) = ln(x)

A few techniques are used throughout the process namely log laws, substitution and the limit identity for the exponential function.

Music by Adrian von Ziegler

[Pastozafaire]

Brilliant! Love the background music as well....

[sophiedillon]

THIS IS SO HELPFUL THANK YOU!!
However, why do we use substitution? I didn't understand that step!!

[JamesJon]

Good lord! That was brilliant and complicated. I hope I'm never expected to whip something like that out of thin air.

[tianlilee]

That substitution seemed a little dubious because it converts h, a constant scalar to be a function of x. Without the substitution, we can still carry the factor 1/h as a power, that will give us ln( exp( 1/x)), which still gives us what we want, nonetheless, I love the use of the exponential identity

[archieforsyth]

Is this not circular reasoning, as the limit of sin(x)/x to 0 is? The way I know to get the limit as v tends to 0 for the (1 + v)^(1/v) is by using the rule that limit of e^ln(f(v)) = e^lim(ln(f(v))) and then using L’hopital’s rule. The L’hopital’s part uses the result that the derivative of ln(x) = (1/x). Unless there is another way of getting the result for e is this not circular reasoning?

[amirhaiqal]

Thank you, this was hard to find though. Thank you so much

[David-kdlw]

Hi i dont get the use substitution part. Can someone please help?

[Seastric]

eXPERLY DONE, EXACTLY WHAT I LOOKING FOR