filmov
tv
Chinese Math Olympiad Question | Find the shortest distance from a circle to the origin

Показать описание
Given a equation of a circle, can you find the shortest distance from this circle to the origin? There are different methods to solve this problem. For example, we can use calculus or trigonometric substitution to solve this question. In this video, we'll use a geometric method to solve it, which is easer. This is a question of Mathematical Olympiad.
Dr. Wang's Channel:
Recommended playlist:
[Math Olympiad]
[Geometry]
Dr. Wang's Channel:
Recommended playlist:
[Math Olympiad]
[Geometry]
Chinese Math Olympiad Question | Find the shortest distance from a circle to the origin
china vs US math fight
China juniors Math Olympiad Question | China Math Olympiad 1993 #olympiad #china #imo
Solve this Chinese Math Olympiad Question Sample
Chinese IMO team
This is the Gao Kao (Chinese Math Exam) 😱
Viral question from China
China Math Olympiad Question Solved
Germany| can you solve?? | Nice Algebra Problem | Math Olympiad Question
China Math Olympiad Problem #shorts #maths #mathematics #radical #olympiad #algebra
China Math Olympiad Question Solved
Chinese Math Olympiad Question #matholympiadproblem #exponentialproblem #algebraicexpression
A Chinese Math Olympiad Question | You should know this trick!
Find limit | Chinese math competition question
Math Olympiad Question | You should know this trick!!
China Middle School Math Olympiad Question | Simplify the expression
Chinese Middle School Math Olympiad Question. How to solve a system of 2 equations for 3 variables?
HOW CHINESE STUDENTS SO FAST IN SOLVING MATH OVER AMERICAN STUDENTS
Chinese Middle School Math Olympiad. Find x^4+x^(-4) from quadratic equation | Olympiad Mathematics
China | Math Olympiad | A Nice Algebra Problem 👇👇
A Nice Olympiad Exponential Multiplication Problem #short #olympiad #mathematics #maths #exponents
Chinese Middle School Math Olympiad question | Solve an inequality | Olympiad Math
China Math Olympiad Question | You should know this trick
CHINA Mathematical Olympiad-1998 | SCHOOL OF OLYMPIAD | Quadratic | (L-1)
Комментарии
 0:04:29
0:04:29
 0:00:10
0:00:10
 0:05:27
0:05:27
 0:00:16
0:00:16
 0:00:34
0:00:34
 0:00:34
0:00:34
 0:08:04
0:08:04
 0:00:31
0:00:31
 0:11:02
0:11:02
 0:00:07
0:00:07
 0:00:31
0:00:31
 0:01:01
0:01:01
 0:03:20
0:03:20
 0:04:44
0:04:44
 0:00:33
0:00:33
 0:05:58
0:05:58
 0:04:10
0:04:10
 0:00:23
0:00:23
 0:04:20
0:04:20
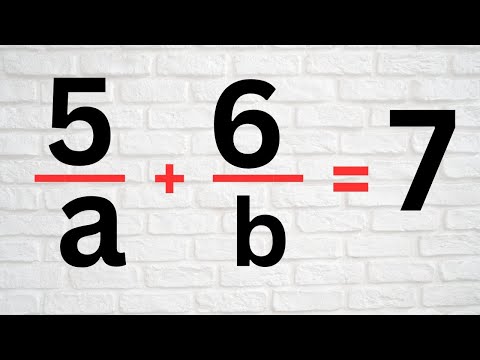 0:16:05
0:16:05
 0:00:52
0:00:52
 0:08:31
0:08:31
 0:02:41
0:02:41
 0:03:32
0:03:32