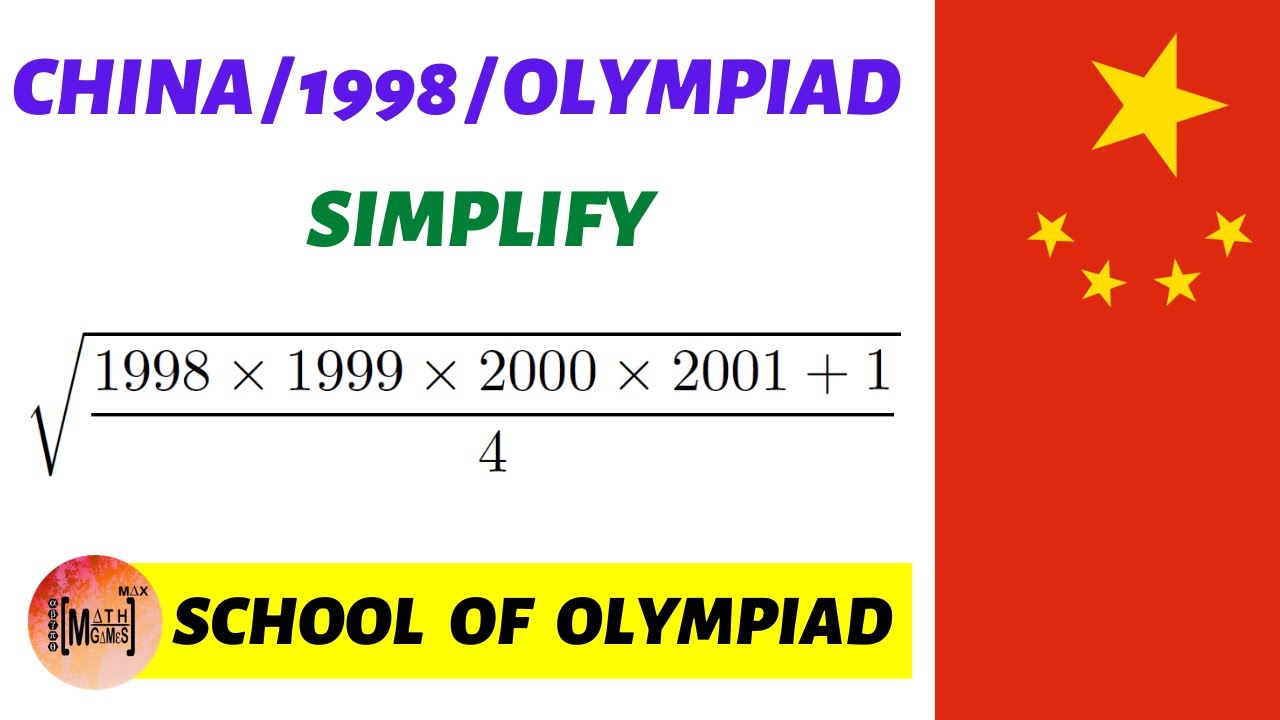
CHINA Mathematical Olympiad-1998 | SCHOOL OF OLYMPIAD | Quadratic | (L-1)

We have to simplify an expression and calculate it without actually calculating.

School of OLYMPIAD is a brand new series by MAX MATH GAMES where we will be solving many questions were asked in Junior Maths Olympiads.

This one is from CHINA 1998 Junior Maths Olympiad (China Mathematical Competitions for Secondary Schools except for CHNMOL)

Maths is a universal subject, all the nation have a common ground. International Maths Olympiad where we all unite.

⚡⚡⚡ THIS SERIES WILL BE - NO DRAMA, NO CLICKBAIT, ONLY MATH.
This is 🌎🌍🌏 SCHOOL OF OLYMPIADS / OLYMPIAD 101 / MATHS 101

✤ Do follow us on:

🎯 Aim for Maths Olympiad Gold 🥇

✔️ Maths Olympiad Class 8
✔️ Maths Olympiad Class 9
✔️ Maths Olympiad Class 10
✔️ Maths Olympiad Class 11
✔️ Maths Olympiad Class 12

🎯 Here’s a quick rundown of what you’re about to learn ⬇️
⚬ IOQM 2020-21
⚬ IOQM 2021
⚬ Maths Olympiad Exam 2020 (Olympiad Maths)
⚬ Maths Olympiad 2020 - NSEJS 2020, NSEB , NSEA & NSEC
⚬ Maths Olympiad Class 8
⚬ Maths Olympiad Class 9
⚬ National Maths Olympiad / NSO
⚬ INMO Preparation
⚬ RMO Olympiad
⚬ PRMO Class 8 (NSO Olympiad)
⚬ HBCSE Olympiad

⭐ Sounds Amazing?
WE WILL BE BRINGING TO QUESTIONS FROM ALL AROUND THE GLOBE.
⚬ AHSME - American High School Mathematics Examination
⚬ AIME - American Invitational Mathematics Examination
⚬ APMO - Asia Pacific Mathematics Olympiad
⚬ ASUMO - Olympics Mathematical Competitions of All the Soviet Union
⚬ BMO- British Mathematical Olympiad
⚬ CanMO - Canadian Mathematical Olympiads
⚬ CHNMOL- China Mathematical Competition for Secondary Schools
⚬ CHNMOL(P) - China Mathematical Competition for Primary Schools
⚬ CHINA - China Mathematical Competitions for Secondary Schools except for CHNMOL
⚬ CMO -Canada Mathematical Olympiad
⚬ HUNGARY -Hungary Mathematical Competition
⚬ IMO - International Mathematical Olympiad
⚬ INMO - Indian National Mathematical Olympiads
⚬ IREMO - Ireland Mathematical Olympiad
⚬ KIEV - Kiev Mathematical Olympiad
⚬ MOSCOW - Moscow Mathematical Olympiad
⚬ POLAND - Poland Mathematical Olympiad
⚬ PUTNAM - Putnam Mathematical Competition
⚬ RUSMO - All-Russia Olympics Mathematical Competitions
⚬ SSSMO - Singapore Secondary Schools Mathematical Olympiads
⚬ SMO - Singapore Mathematical Olympiads
⚬ SSSMO(J) - Singapore Secondary Schools Mathematical Olympiads
for Junior Section
⚬ SWE - Sweden Mathematical Olympiads
⚬ USAMO - United States of American Mathematical Olympiad
⚬ USSR - Union of Soviet Socialist Republics

#MathsOlympiad #olympiad #olympiadexam2020 #olympiadexam2021 #StayHomeWithMe #WithMe #LearningWontStop #mathsandphysicsfun #UnitedbyMathematics

👉🏼 If you're looking to get a more effective Olympiad 2021 Preparation playlist like this one, make sure to subscribe to our channel right here:

Thanks for watching this session of SCHOOL OF OLYMPIAD . Hope you have enjoyed and learned more about Maths Olympiad Exams 2020 and How to Prepare for Maths Olympiad Exam 2020 for Maths Olympiad 2020 (Olympiad Exams 2020).

😇 PRO TIP : Always remember, the best way to learn mathematics, developing logical abilities and enhancing your brain power is to try to solve these questions yourself. And hence, after seeing the question, pause the video and give this question a try, and then resume the video when you wish to check out the solution.

🚀 MAX MATH GAMES Playlist links:

✅✅Do Subscribe and Press the 🔔 Bell Icon (All) to get notifications for all new videos:

[lololol]

At the end, suppose the test forbids the use of calculators, how are we supposed to calculate 1998² ? Any tricks?

[serhiypidkuyko]

it's a simple question:
let x = 1999
then the expression = (1/2)√[(x-1)x(x+1)(x+2)+1] = (1/2)(x^2+x-1) = (1/2)(1999^2+1998)
since (x-1)x(x+1)(x+2)+1 = (x^2+x)(x^2+x-2)+1=(x^2+x)^2 - 2(x^2+x) + 1 = (x^2+x-1)^2

[matheusurbano]

Consider solving some OBM questions. This one is the brazilian mathematics olympiad