The clever way curvature is described in math

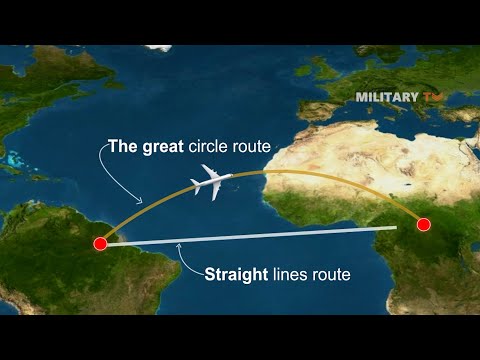
How do mathematicians describe curvature of surfaces? There are two measures: Gaussian and mean curvatures, and both are useful in differential geometry, the study of surfaces and higher-dimensional manifolds (or lower-dimensional curves).

I deliberately didn't say principal curvatures, which are the eigenvalues of the shape operator. The eigenvalues are guaranteed to be real, and the eigenvectors must also be orthogonal, because the shape operator is real and symmetric. However, getting to the point where we can prove the shape operator is real and symmetric is a bit tricky (can be proved rather easily with computations, but I'm not sure how to do it "intuitively"); and getting from real symmetric matrices to real eigenvalues and orthogonal eigenvectors is another thing that I still don't know how to think about intuitively.

Files for download:

Sources:

- Visual Differential Geometry and Forms by Tristan Needham
For this whole series, I have not consulted this book, but it should be a nice resource anyway for the geometric intuitions.

- Soap film images:

Other than commenting on the video, you are very welcome to fill in a Google form linked below, which helps me make better videos by catering for your math levels:

If you want to know more interesting Mathematics, stay tuned for the next video!

SUBSCRIBE and see you in the next video!

If you are wondering how I made all these videos, even though it is stylistically similar to 3Blue1Brown, I don't use his animation engine Manim, but I use PowerPoint, GeoGebra, and (sometimes) Mathematica to produce the videos.

Social media:

For my contact email, check my About page on a PC.

See you next time!

[mathemaniac]

First video of a trilogy about minimal surfaces - surfaces that minimise surface area given boundary conditions. Please LIKE, SUBSCRIBE, COMMENT as usual. The next video is how soap films take the shape they do (rather than why).

There are different conventions for the Gauss map: do you take the outward normal or inward normal? And what does outward or inward mean if the surface is not compact? There are also different conventions for the shape operator / second fundamental form, where you might see something like S(phi_u) = -n_u instead.

Regardless, all those conventions differ by negative signs, so it doesn't matter too much. (Except for the people who define mean curvatures in such a way that the mean curvature of a sphere is negative. Or people who define shape opeartor such that on the unit sphere it is not the identity map. What are you thinking?)

[darkshoxx]

Great video and easy to follow explanations. Quick Tangent 😉: I went through all of this at university in our second year, and we only ever got two visual demonstrations/examples about this, and I was surprised it didn't come up here. It was about how Gaussian curvature is a measure of internal geometry and therefore independent of external transformations. Meaning if you roll a piece of paper into a cylinder, they will both have the same Gaussian curvature (namely zero). And for the same reason, a slice of pizza that is curved by gripping the crust and bending it will remain straight towards the tip and not bend downwards, because that shape would have positive Gaussian curvature, but pizza has a Gaussian curvature of zero, so that can't happen. In other words, you can't bend a flat surface in two directions at the same time.

[TTuCbKoTpIc]

5:07 for those who like me didn't understand the reason why the normal vector can only be assosiated with a point of the circle and not the whole sphere, just imagine moving it across the cylinder along v. As it stays perpedicular to the surface, it can only be directed toward a point on the circle

[moonshine]

Love this. I just finished studying these things this semester and you nailed it! I'm surprised you managed to not mention eigenvalues, but I think it's good, they weren't really necessary to the video.

[copywright]

TO EVERYBODY WHO READS THIS. Please upvote this video, and maybe let it play in the background multiple times, it's legitimately some of the best content on youtube, and it's not getting the views it deserves.

Since I've begun to work on videos of a similar genre and style, I know how much time and effort goes into not just creating, but VERIFYING everything, along with making sure its intelligible. It's wayyyy too easy to use math jargon and confuse the heck out of everyone watching. That he manages to break things down so well along with having stellar production value is not something the internet should take for granted.

Tl;dr I'm glazing mathemaniac but it's well deserved

[krzysiekczajkowski]

Please do more on differential geometry. Great video!

[adriencances]

Astonishingly clear and well written, with great animations, keep up this amazing work man!

[chalkchalkson]

In case you are somehow looking for subjects to cover in this series, I think it would be really cool to discuss how this framework generalises to Riemann manifolds and metric tensors. Not only because it's really neat to relate curvature and different notions of distance (like "how different are our longitudes and latitudes" vs "how many meters are we apart"), but also because it's always fun when geoemtry and fancy physics intersect :)

[prashantkumarsingh]

Thank you for this wonderful explanation.

[Iovemath]

8:56 Gaussian Curvature in a sense is the measurement of how the surface can reshape to a flat plane without distortion. A paper can easily curve into a cylinder by just roll it up. So the curvature of a cylinder is 0.

[ayte]

Surprised how few comments this video has, so I'm leaving my own. Great content!

[as-qhqq]

In the sphere map, the normal vector isn't on the surface of the sphere but at the origin...the sphere is the locus of the tip of all possible unit normals.

[Malicide]

Just curious based on your chosen topic: are you a fan of the works of Pavel Grinfeld (aka the mathisbeautiful channel guy)? Reading his texts really helped me wrap my mind around tensors, curvature, and minimal surfaces, not unlike your videos. And thanks for the channel, great stuff as always!

[EarlJohn]

"It's bendy..."
"It has the same shape as my girlfriend... (hubba hubba)"
I'm sure there are others that are nearly as descriptive. 😅😅😅

[jomilariola]

Great Video, Never expected Curvature being measured via linear algebra, but makes sense in retrospect
Q: btw whats the background music? (ex: 1:50), it feels familiar…

[kovanovsky]

Great video! Since I majored in physics and studied GR, I'm wondering if you're gonna cover Riemann tensor, Ricci tensor, and Ricci scalar. Would be great to see how they are connected to these two types of curvature (or how perhaps they are the same as these two) 😃

[ringoffire]

I just learned all about this yesterday, what a crazy coincidence. Too bad I didn’t see this vid until now

[alexboche]

Nice Video! Can you clarify how/if this applies to higher dimensional manifolds?

[Mayo-rv]

يجب السرد التاريخي
ويجب تعريف الحاجه لهذه الرياضيات
الجميله
والأسباب الحقيقيه لوجودها

[apteropith]

i've been trying to work out something about the curvature of hyperboloids

clearly, a hyperboloid embedded in ordinary 3D space has a varying positive gaussian curvature
but, a hyperboloid generated by rotations in a minkowski space is a surface of uniform _negative_ curvature

this makes some sense given that it's effectively an anti-sphere, but how one would _calculate_ that curvature as negative has never been clear to me

but i'd previously been given oversimplified accounts of how gaussian curvature is calculated, so now i'm guessing that in this matrix-formulation the metric tensor has to be inserted somewhere somewhen (i've never liked the matrix formulations for minkowski spaces, because that "somewhere" and "somewhen" is deeply unintuitive to me)