filmov
tv
Logarithm Expressions in 3 Minutes - Quick Maths
Показать описание
A quick video on logarithm expressions and definitions, followed with some exercises! I hope you guys find this video helpful for your exams, and I will be uploading a few more videos on log some time soon. Good luck!
Transcript:
Hi everyone! In today’s video, I will be going over the definition of logarithms and some sample questions. To put simply, log tells us how many times we have to multiply the same number to get another number. So to get 64, how many 4’s do we have to multiply together? We need to multiply it 3 times, so the log of this particular example would be 3. You can think of it as finding the exponent.
To start, we will go over the definition of log, which is if y equals to log, base b, of x, (y = logbx) then x = by. This is what we call the logarithmic form, and this is what we call the exponential form. So if I give you 8 = 23, then its log form would be 3 = log2 8. I usually visualize the number the log equals to as the exponent, and the base of the log as the number under the exponent. So as a quick exercise, I will give you a few expressions, and I would like you to convert them either from log form to exponential form, or vice versa. Pause the video to try them out!
Alright, now let’s go over solving for some x’s in those expressions. For example, let’s say I want you to find what does log, base 5, of 25 (log5 25) equal to. According to the definition, we know that the base of the log is the number under the exponent, and the exponent is what we are trying to find. So 5 to the power of what equals to 25? It would simply just be 2. And how about this, what is x in this question (log3 x = 4)? Again, we use the definition, and find that the base of the log is 3, to the power of 4, which is the number on the log forms equals to. We find that x is 81. And now, let’s do more practice questions! Pause the video if you need.
Thank you for watching and I hope that this video helped you out! My next few videos will be on solving log equations and properties, good luck with exams!
#mathhelp #mathtutorial #log
Transcript:
Hi everyone! In today’s video, I will be going over the definition of logarithms and some sample questions. To put simply, log tells us how many times we have to multiply the same number to get another number. So to get 64, how many 4’s do we have to multiply together? We need to multiply it 3 times, so the log of this particular example would be 3. You can think of it as finding the exponent.
To start, we will go over the definition of log, which is if y equals to log, base b, of x, (y = logbx) then x = by. This is what we call the logarithmic form, and this is what we call the exponential form. So if I give you 8 = 23, then its log form would be 3 = log2 8. I usually visualize the number the log equals to as the exponent, and the base of the log as the number under the exponent. So as a quick exercise, I will give you a few expressions, and I would like you to convert them either from log form to exponential form, or vice versa. Pause the video to try them out!
Alright, now let’s go over solving for some x’s in those expressions. For example, let’s say I want you to find what does log, base 5, of 25 (log5 25) equal to. According to the definition, we know that the base of the log is the number under the exponent, and the exponent is what we are trying to find. So 5 to the power of what equals to 25? It would simply just be 2. And how about this, what is x in this question (log3 x = 4)? Again, we use the definition, and find that the base of the log is 3, to the power of 4, which is the number on the log forms equals to. We find that x is 81. And now, let’s do more practice questions! Pause the video if you need.
Thank you for watching and I hope that this video helped you out! My next few videos will be on solving log equations and properties, good luck with exams!
#mathhelp #mathtutorial #log
0:02:58
0:01:45
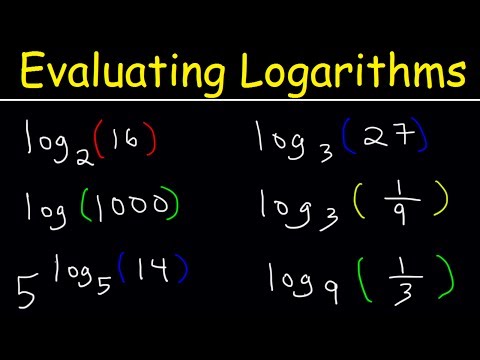0:10:20
0:04:31
0:02:46
0:03:20
0:01:23
0:02:44
0:11:09
0:03:11
0:01:21
0:01:50
0:04:54
0:10:37
0:03:04
0:12:48
0:01:26
0:01:32
0:21:12
0:02:56
0:03:46
0:04:37
0:03:40
0:02:48