filmov
tv
Evaluate this Log Expression In 1 Minute | NO Calculators Allowed

Показать описание
Evaluate this Log Expression In 1 Minute | NO Calculators Allowed
Evaluate this Logarithmic Expression in 1 Minute | Fast & Easy Explanation | No Calculators
Logarithms - The Easy Way!
Evaluate the Logarithmic Expression
Evaluating Basic Logarithms Without a Calculator
Pre-Calculus - Learn How To Evaluate a Logarithmic Expression
Can YOU evaluate this LOG Expression?
Evaluating a Logarithmic Expression in Three Ways
PSPP Practical - 3
Precalculus: Evaluating the Base b Logarithmic Expression
Precalculus: Evaluating the Base b Logarithmic Expression
Can YOU evaluate this LOG expression (No Calcs allowed)
Precalculus: Evaluating the Base b Logarithmic Expression
Solving Logarithmic Equations
Evaluating A Logarithmic Expression
Evaluating A Logarithmic Expression
Pre-Calculus - Simplifying the Logarithmic Expression Without a Calculator
Properties of Logarithms
Evaluate the Logarithmic Expression @KasyannoEZMath
Q3 Multiple Choice Evaluate Log Expression
Fancier logarithm expressions | Logarithms | Algebra II | Khan Academy
Evaluate Log Expression with Radicals Q7
Natural Logarithms
Algebra 2 - How to Evaluate a Logarithmic Expression Using Rules of Logarithms
Комментарии
 0:04:54
0:04:54
 0:04:24
0:04:24
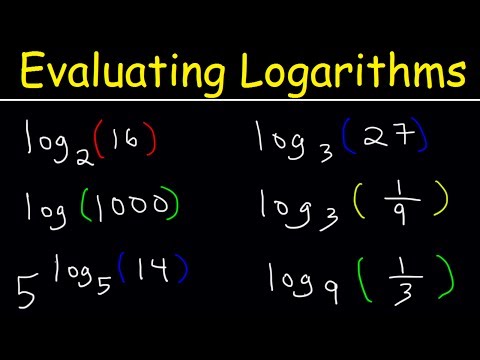 0:10:20
0:10:20
 0:00:58
0:00:58
 0:03:27
0:03:27
 0:01:04
0:01:04
 0:02:51
0:02:51
 0:09:31
0:09:31
 0:09:25
0:09:25
 0:00:42
0:00:42
 0:00:46
0:00:46
 0:04:09
0:04:09
 0:00:39
0:00:39
 0:25:27
0:25:27
 0:07:47
0:07:47
 0:09:36
0:09:36
 0:00:53
0:00:53
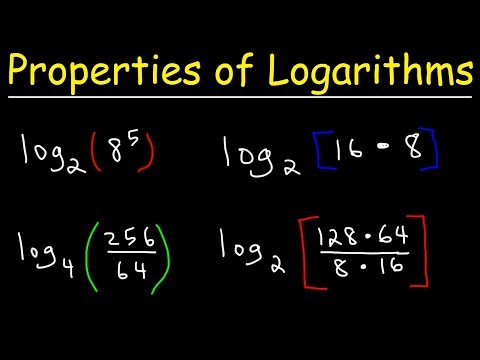 0:05:40
0:05:40
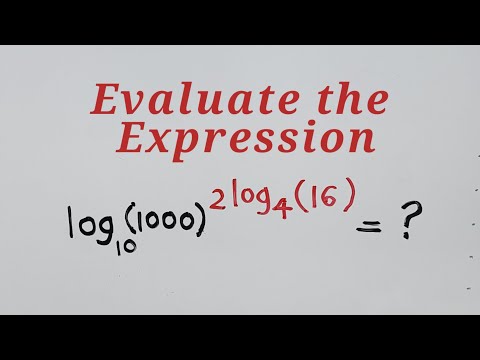 0:02:41
0:02:41
 0:00:44
0:00:44
 0:04:20
0:04:20
 0:04:31
0:04:31
 0:02:49
0:02:49
 0:01:15
0:01:15