filmov
tv
Finding the range for projectile motion from a cliff
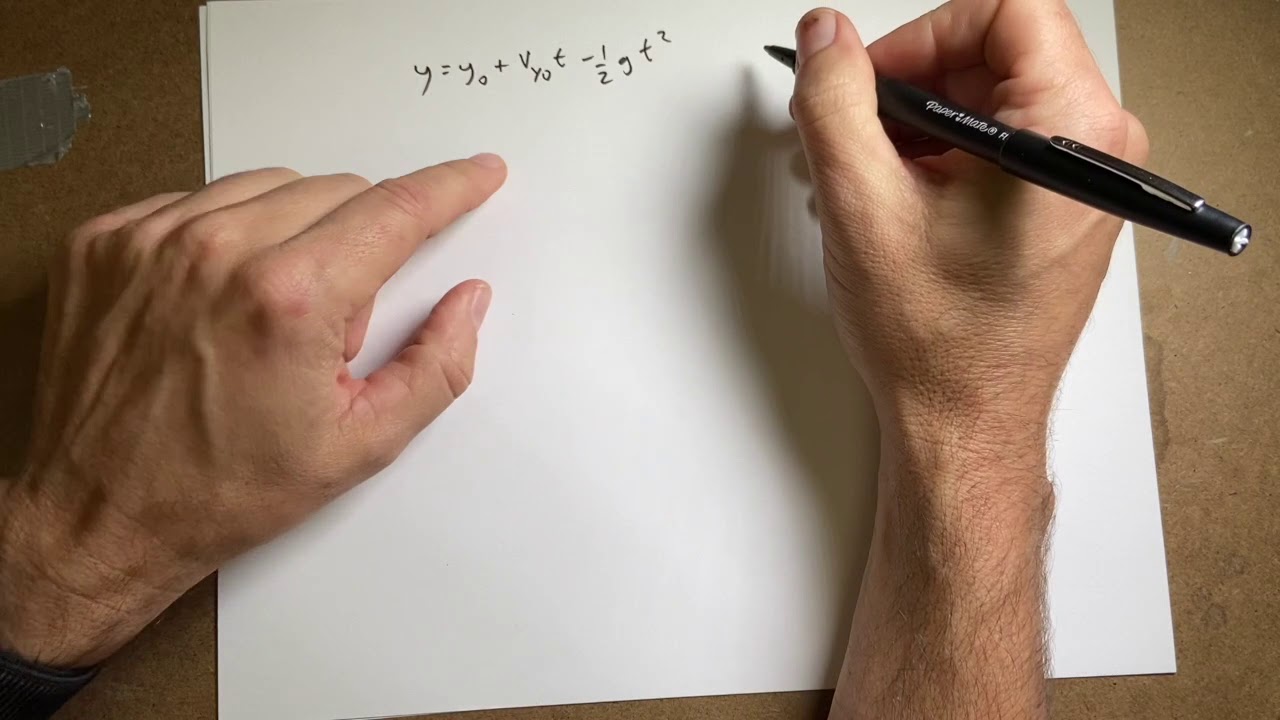
Показать описание
This is pretty much the hardest projectile motion problem you will see. If you can solve this, you should be OK.
Here a ball is on a cliff 12 meters high and launched with a velocity of 5 m/s at a 30 degree angle. How far from the base of the cliff will the ball land?
Here a ball is on a cliff 12 meters high and launched with a velocity of 5 m/s at a 30 degree angle. How far from the base of the cliff will the ball land?
Projectile Motion - Maximum Range Angle - Physics & Calculus
Range of a Projectile - quick derivation of the formula
Range of a Projectile in Projectile Motion | Physics
Projectile Motion: Finding the Maximum Height and the Range
Finding the range for projectile motion from a cliff
Calculating Range of Projectile
No Calculus Needed?! How to Maximize Range Using Simple Geometry.
Projectile Motion - How to Find the Maximum Height and Range - Physics
As projectile motion
Introduction to Projectile Motion - Formulas and Equations
Projectile motion physics - Range general formula
Kinematics Part 3: Projectile Motion
maximum range of projectile
How To Solve Projectile Motion Problems In Physics
Projectile Motion - Find Distance
Finding the range and highest point of a projectile
VNV CLASSES - IIT JEE Maximum Range in Projectile from a Height
Further Physics: Derivation of Max Range for Projectile Motion | Kinematics
Find the Horizontal Range of a Projectile Motion
introduction to projectile motion
Calculating the angle of elevation and range of a projectile
Projectile Motion Calculating the Maximum Height Part 6
Projectile Motion: Finding time of flight, range, velocity and direction before striking the ground
Physics 3: Motion in 2-D Projectile Motion (27 of 31) Find Final Velocity=? (Example 1)
Комментарии
 0:05:35
0:05:35
 0:02:40
0:02:40
 0:05:27
0:05:27
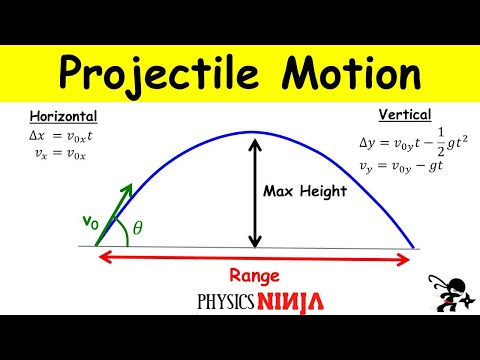 0:21:09
0:21:09
 0:19:57
0:19:57
 0:06:28
0:06:28
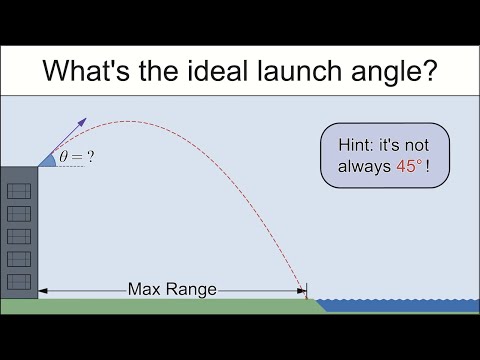 0:06:51
0:06:51
 0:23:33
0:23:33
 0:43:36
0:43:36
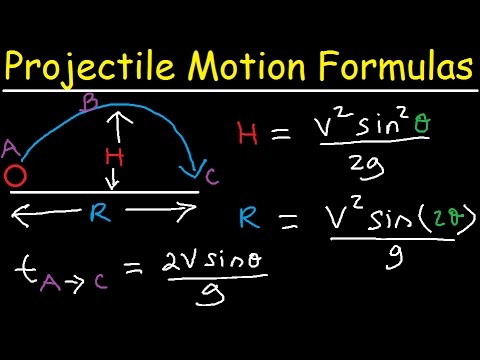 0:28:11
0:28:11
 0:14:49
0:14:49
 0:07:06
0:07:06
 0:02:56
0:02:56
 0:28:19
0:28:19
 0:04:15
0:04:15
 0:07:01
0:07:01
 0:06:59
0:06:59
 0:33:49
0:33:49
 0:07:10
0:07:10
 0:05:09
0:05:09
 0:05:37
0:05:37
 0:03:09
0:03:09
 0:11:03
0:11:03
 0:07:13
0:07:13