filmov
tv
Examples for optimization subject to inequality constraints, Kuhn-Tucker

Показать описание
Two examples for optimization subject to inequality constraints, Kuhn-Tucker necessary conditions, sufficient conditions, constraint qualification
Errata: At 17:32, (6/5, 8/5) is the only candidate point; (6/5, -8/5) does not satisfy the equation 4-2\lambda_1 y=0. At 46:55, (0,0) is not a candidate point, since it contradicts y larger than 2. At 50:56, it should be +26, not -26.
Errata: At 17:32, (6/5, 8/5) is the only candidate point; (6/5, -8/5) does not satisfy the equation 4-2\lambda_1 y=0. At 46:55, (0,0) is not a candidate point, since it contradicts y larger than 2. At 50:56, it should be +26, not -26.
Examples for optimization subject to inequality constraints, Kuhn-Tucker
More examples for optimization subject to inequality constraints, Kuhn-Tucker
Constrained optimization introduction
Linear Programming (Optimization) 2 Examples Minimize & Maximize
Lagrange multipliers, using tangency to solve constrained optimization
Constrained Optimization: Intuition behind the Lagrangian
lagrangians in economics: constrained optimization
Optimization Problems EXPLAINED with Examples
Sluggish Form? Optimizing Form Performance for Faster Loading in Microsoft Access, Part 2
Utility Maximization using Lagrange Method. utility optimization #lagrange #utility
Lagrange Multipliers | Geometric Meaning & Full Example
Optimization 2 Examples and Constraints
How to Solve ANY Optimization Problem | Calculus 1
Unconstrained Optimization - Examples I
Constrained Optimization
Constrained Optimization. Cost minimisation from given Cost function with Production Constraint
Static Optimization for Economists Part 1: The Method of Lagrange
Maximize Utilitiy Subject to Budget Constraint. Using Lagrange's Multiplier for Optimization
Spark performance optimization Part1 | How to do performance optimization in spark
Linear and Quadratic Optimization Models
Lecture 14 | Lagrange Dual Function | Convex Optimization by Dr. Ahmad Bazzi
Kuhn Tucker's Method with Numerical Example | Optimization Techniques
Query Optimization | SQL Query Optimization with Examples
Lagrange multiplier method of optimization
Комментарии
 0:53:53
0:53:53
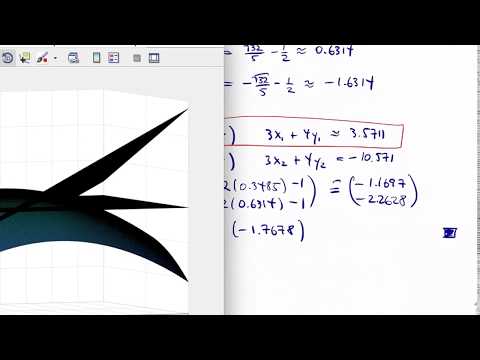 0:44:18
0:44:18
 0:06:29
0:06:29
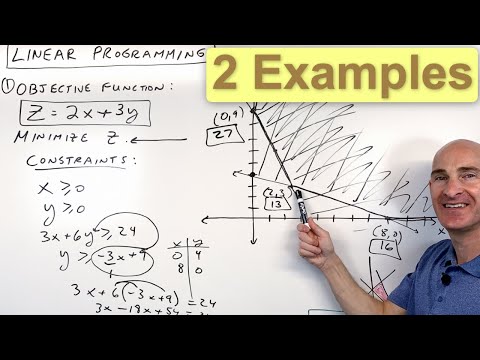 0:15:08
0:15:08
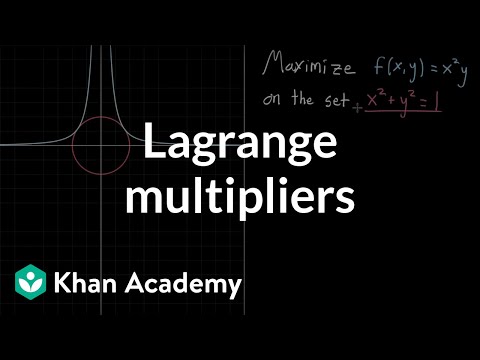 0:08:43
0:08:43
 0:10:49
0:10:49
 0:10:17
0:10:17
 0:10:11
0:10:11
 0:21:13
0:21:13
 0:08:37
0:08:37
 0:12:24
0:12:24
 0:57:32
0:57:32
 0:21:03
0:21:03
 0:07:20
0:07:20
 0:09:13
0:09:13
 0:10:41
0:10:41
 0:30:55
0:30:55
 0:11:37
0:11:37
 0:20:20
0:20:20
 0:24:51
0:24:51
 0:37:47
0:37:47
 0:42:04
0:42:04
 0:09:45
0:09:45
 0:11:49
0:11:49