filmov
tv
Maximize Utilitiy Subject to Budget Constraint. Using Lagrange's Multiplier for Optimization
Показать описание
This is fourth video on Constrained Optimization. In this video I have tried to solve a Utility Function With the given constraint.
The question was to maximize Utility Function with the given constraint.
The solution was like this
1. Set constraint equal to zero
2. Multiply it by 'lambda'
3. Form a Lagrange's Function
4. Partially differentiate Lagrange's Function with respect to the variables sand set them equal to zero
5. Solve for the variables to get optimum values
These videos are specially for Masters In Economics Aspirants who have difficulty in Mathematical Economics .
You can Join
On Facebook
Facebook page
On Telegram
The question was to maximize Utility Function with the given constraint.
The solution was like this
1. Set constraint equal to zero
2. Multiply it by 'lambda'
3. Form a Lagrange's Function
4. Partially differentiate Lagrange's Function with respect to the variables sand set them equal to zero
5. Solve for the variables to get optimum values
These videos are specially for Masters In Economics Aspirants who have difficulty in Mathematical Economics .
You can Join
On Facebook
Facebook page
On Telegram
0:11:37
Maximize Utilitiy Subject to Budget Constraint. Using Lagrange's Multiplier for Optimization
0:13:30
Maximize Utility function subject to budget constraint #utilityFunction #GATE #NET #Lagrange
0:05:31
Finding optimal quantities from the given utility function and budget Constraint
0:09:59
Marginal Analysis and Consumer Choice- Micro Topic 1.6
0:11:02
Micro: Unit 2.2 -- Utility Maximization
0:08:37
Utility Maximization using Lagrange Method. utility optimization #lagrange #utility
0:09:26
Utility Maximizing Bundle
0:06:46
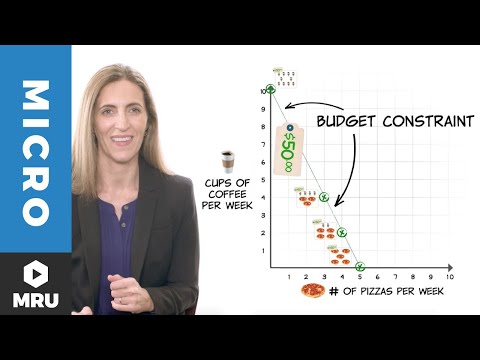
Budget Constraints
0:21:53
Budget-Friendly Studio Apartment Makeover Ideas for Modern, Minimalist Interior Inspiration
0:11:38
utility maximization in case of linear utility function. Max u= 2x+3y subject to budget 4x+6y=12
0:09:53
Utility Maximization. optimal bundle from the given utility function & budget constraint #lse #M...
0:07:36
Utility Maximization problem constrained optimization in economics #MRS #indifferencecurve
0:13:11
Utility Maximization problem Max U =Q1Q2, P1=1, P2=4 Budget =120 #utility #optimization #econmath
0:02:25
Utility Maximization: U = XY + 2Y + 2X
0:05:39
Utility Maximization with Kinked Budget Line
0:12:39
Utility Maximization: Algebra-based Solutions
0:06:18
Marginal utility of money from utility function subject to budget constraint u= X1X2 stX1+X2=6
0:31:18
Constrained Optimization -The Lagrangian Method of Maximizing Consumer Utility| Complete Derivation
0:06:49
Maximizing Consumer Utility with Three Goods
![(M3E6) [Microeconomics] Utility](https://i.ytimg.com/vi/Alu1DCMiTD4/hqdefault.jpg)
0:07:23
(M3E6) [Microeconomics] Utility Maximization Problem with Quasi-Linear Utility Functions
0:08:02
Principles of Microeconomics: How to Maximize Utility
0:06:29
Consumer Optimization
0:20:24
PMAP 8141 • Example: Maximizing utility
0:12:58
Maximizing Utility
Комментарии