filmov
tv
Quadratic formula for non-constant coefficients
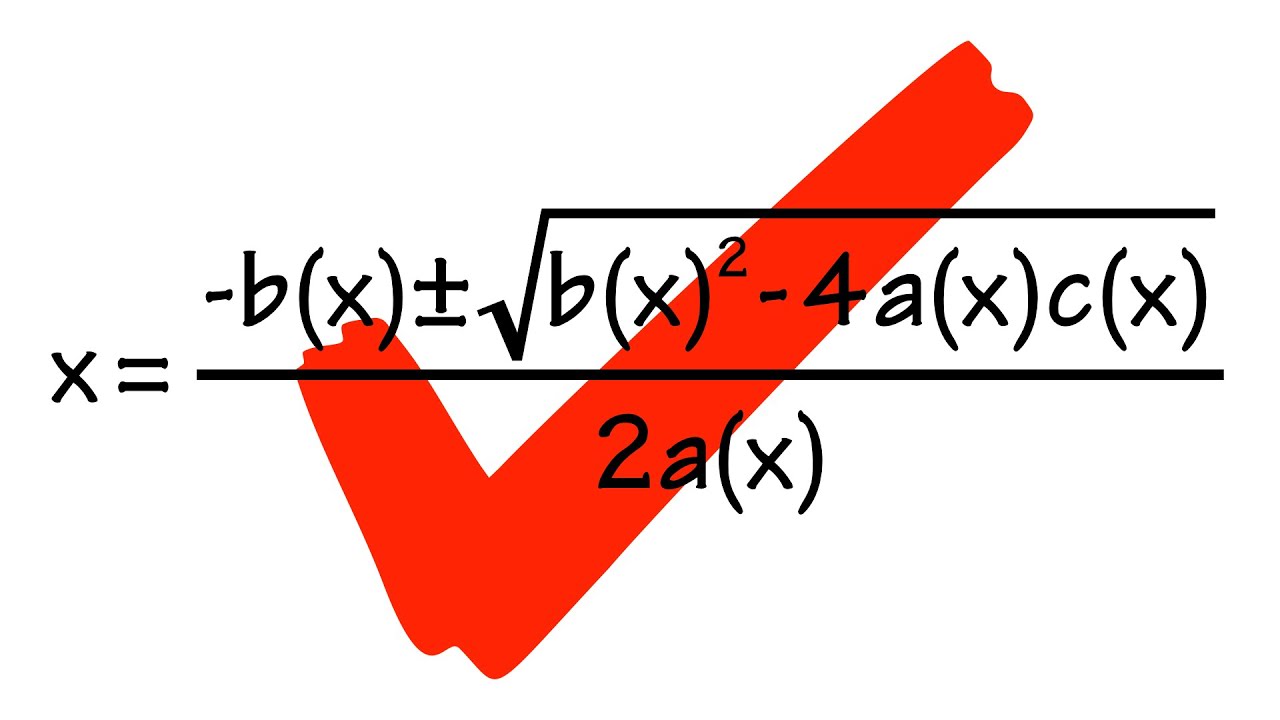
Показать описание
I was driving back home and wondered if the quadratic formula still works if a, b, c are not constants? In fact, what if a, b, and c are functions of x?
0:00 Quadratic formula for nonconstant coefficients
2:30 Creating x^2+(sin(x))x-1/2*cos(x)=0
----------------------------------------
Big thanks to my Patrons for the full-marathon support!
Ben D, Grant S, Erik S. Mark M, Phillippe S. Michael Z, Nicole D. Camille E.
Nolan C. Jan P.
----------------------------------------
#blackpenredpen #math #calculus #apcalculus
0:00 Quadratic formula for nonconstant coefficients
2:30 Creating x^2+(sin(x))x-1/2*cos(x)=0
----------------------------------------
Big thanks to my Patrons for the full-marathon support!
Ben D, Grant S, Erik S. Mark M, Phillippe S. Michael Z, Nicole D. Camille E.
Nolan C. Jan P.
----------------------------------------
#blackpenredpen #math #calculus #apcalculus
Quadratic formula for non-constant coefficients
How To Solve Quadratic Equations Using The Quadratic Formula
Solving Without Factoring or Quadratic Formula
How To Solve A Quadratic Equation With Only Two Terms. Constant Term Is Missing.
Undetermined Coefficients: Solving non-homogeneous ODEs
Quadratic Equations | Solve by factoring | Free Math Videos
Quadratic Equation Shortcut Trick | Solve Equation in 5 Seconds
ERTH/OCN312: Higher Order ODE's with Non-Constant Coefficients
Engineering Mathematics 18 | Particular Integrals, Wronskian and Euler - Cauchy Equations 2.0
Solving a Quadratic Equation Using the Quadratic Formula #Shorts #math #maths #mathematics #algebra
quadratic formula in 12 seconds!
Solve quadratic equation by factorisation
Solving Quadratic Equations by Factoring│Algebra
How To Find The Equation of a Quadratic Function From a Graph
Factoring Negative Quadratic Equations
How to Solve Quadratic Equation with Complex Coefficient
Nonhomogeneous DE with Constant Coefficients, Part 1
Solving Quadratic Equations using Quadratic Formula - Quadratic Equations
Quadratic Formula Derivation
Learn the ac method for factoring and solving a quadratic equation
Solving using the quadratic formula with complex solutions
Find the Value of K for which the Quadratic has Equal Roots - Quick and Simple Explanation
Factoring when a is greater than one
Quadratic Equations Grade 10
Комментарии
 0:09:10
0:09:10
 0:05:56
0:05:56
 0:00:58
0:00:58
 0:02:15
0:02:15
 0:12:44
0:12:44
 0:02:43
0:02:43
 0:00:30
0:00:30
 0:31:01
0:31:01
 1:37:33
1:37:33
 0:00:32
0:00:32
 0:00:19
0:00:19
 0:09:05
0:09:05
 0:22:30
0:22:30
 0:09:35
0:09:35
 0:09:42
0:09:42
 0:17:30
0:17:30
 0:06:50
0:06:50
 0:09:06
0:09:06
 0:00:48
0:00:48
 0:05:00
0:05:00
 0:05:28
0:05:28
 0:03:35
0:03:35
 0:03:50
0:03:50
 0:05:17
0:05:17