The 3 Jugs Riddle

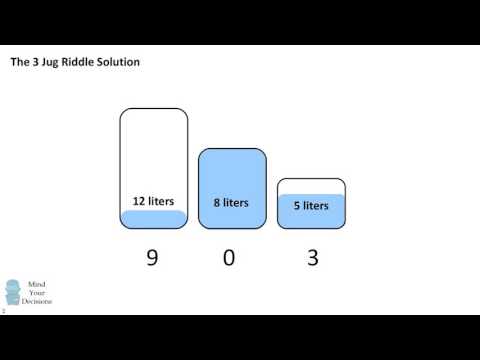
You have a full 12 liter jug and empty 5 and 8 liter jugs. Can you measure exactly 6 liters? This problem dates to 1484 and was posed in the context of a milkman making a home delivery to a customer. The story goes this riddle so delighted Simeon Denis Poisson as a young boy that it was one reason he pursued mathematics.

Connect on social media. I update each site when I have a new video or blog post, so you can follow me on whichever method is most convenient for you.

My Books

[simonshugar]

Tell the customer he's only getting 5 liters of milk until he gets a 6-liter jug

[spiderjump]

moral of the riddle the vendor should get a one liter ladle .

[pellaken]

0:24 leave a note that says "please ensure you have a properly sized jug for delivery" and leave.

[lvlarihuan]

By the time they finsh puring in and from, the milk gets rotten.

[TonboIV]

The trick is to figure out the last move first. The 5 litre can't hold 6 so you need to somehow get 6 into the 8 litre. The obvious way is to fill it up and pour out 2 litres, so you need to have another container with only space for 2 litres, and once you consider the differences between the container sizes, the way to do that is pretty obvious.

[TeraAFK]

Or you can just tilt the 12L jug and pour until the the surface makes a diagonal...?

[JDeWittDIY]

The solution you described is the 12-4-9-1-6 solution (the original container goes through the sequence of having 12, 4, 9, 1, 6 liters). There are other solutions as well. For example I found a 12-0-8-3-11-6 solution:

(Container order here is 12, 8, 5)
12, 0, 0 -- Start
0, 8, 4 -- Pour 12 liters from original into the other two containers (filling the 8).
8, 0, 4 -- Pour the 8 back into original.
8, 4, 0 -- Transfer the 4 liters from the 5 to the 8 container.
3, 4, 5 -- Pour 5 liters from the original to the 5, leaving 3 in the original.
3, 8, 1 -- Fill up the 8 container with the milk in the 5, leaving one liter in the 5.
11, 0, 1 -- Pour the 8 liters from the 8 container to the original, you now have 11 in the original
11, 1, 0 -- Transfer the 1 liter from the 5 to the 8 container.
6, 1, 5 -- Fill the 5 container from the original. The customer now has 6 liters (in two containers) and the milkman has 6 liters.

I'm now affectionately calling these the 4-9-1 solution and the 8-3-11 solution respectively. There might be other solutions, but I haven't found any that don't match these two basic patterns.

[cameronlambert]

There is a much simpler way to do it, you simply pour the 12L container into the 8L container until the 12L one is halfway empty, you can tell when it is exactly halfway because when you tilt the 12L container, it will make a perfect diagonal from the opposite corner of the jug. Much easier and more sanitary!

[Uejji]

This problem is an applied representation of the function 8x + 5y = 6, mapping Z -> Z

x represents the net number of times to fill or empty the 8 liter jug
y represents the net number of times to fill or empty the 5 liter jug

x, y only increment if filling a jug from empty to full from the 12 liter jug
x, y only decrement if empty a jug from full to empty to the 12 liter jug

Though in practice you'll probably be using one specifically to fill and the other specifically to empty.

Transferring water between the 8 and 5 liter jugs results in net zero change in water amount (assuming no spillage) and is not an arithmetic operation.

The particular solution presented in this video is (2, -2). The 8 liter jug is filled completely from empty to full from the 12 liter jug twice and the 5 liter jug is emptied from full to empty into the 12 liter jug twice.

The general solution set is (5n+2, -8n-2). Plug in any integer for n and see if you can construct a scenario.

For instance, n=-1 -> (5(-1)+2, -8(-1)-2) = (-3, 6)
-Fill 5 (0, 1), transfer to 8. (7:5:0)
-Fill 5 again (0, 2), transfer to 8. There's not enough room, so empty 8 when it is full (-1, 2) and finish transferring. (10:2:0)
-Fill 5 again (-1, 3), transfer to 8. (5:7:0)
-Fill 5 again (-1, 4), transfer to 8. Empty 8 when full (-2, 4) and keep transferring. (8:4:0)
-Fill 5 again (-2, 5), transfer to 8. Empty 8 when full (-3, 5) and keep transferring. (11:1:0)
-Fill 5 again (-3, 6), transfer to 8. You are finished. (6:6:0)

Not as efficient as the presented solution, but correct just the same. Ideally you would choose the value n that minimizes ||5n+2| + |-8n-2||, which for this problem is n=0, resulting in (2, -2).

[stirrcrazy]

I’m proud to say this is the only problem on this channel I’ve been able to solve before you reveal the answer.

[magnusmohus]

My students loved this problem, but was distracted by the milk colour and the physics. The surface of liquid does not bend that way. I love your account and use it all the time for my advanced mathmatics group.

[ketochikvinidze]

The video showed more of how it's done and less of how to think to achieve those steps. What I did to solve the problem in 13 steps was to try to keep it from "looping" (repeating the exact same situation that already existed). So, if it was about to "loop", I tracked what action would've caused the loop and did something else that was possible.
this method is kind of random so the same result can be achieved with less than 13 steps.
This might not be a universal solution from problems like this, just what I thought of in this specific case.

[NotFlame]

SIMPLE.
GIVE 12L OF MILK.
ASK DOUBLE THE MONEY.
**Insert Lenny Face**

[numberzoog]

But the real question we all need to ask ourselves is "Why is the milk blue?"

[vaibhavpatil]

i aint buying blue milk i think its mixed with water

[TheTmshuman]

And that’s the origin of “don’t cry over spilled milk”

[Tecorsuh]

Before I watch this video, there are a couple of ways I can think to do this. The first and easiest is to pour half out of the 12 liter jug into the 8 liter. This is assuming the container is a fairly regular rectangular prism or cylinder, you can measure half with the liquid touching both a top edge and opposite bottom edge when tilted.
Like this: [/]

Otherwise the complicated process of pouring between containers:
12 to 8. 4 in 12, 8 in 8, 0 in 5
12 to 5. 0 in 12, 8 in 8, 4 in 5.
8 to 12. 8 in 12, 0 in 8, 4 in 5.
5 to 8. 8 in 12, 4 in 8, 0 in 5.
12 to 5. 3 in 12, 4 in 8, 5 in 5.
5 to 8. 3 in 12, 8 in 8, 1 in 5.
8 to 12. 11 in 12, 0 in 8, 1 in 5.
5 to 8. 11 in 12, 1 in 8, 0 in 5.
12 to 5. 6 in 12, 1 in 8, 5 in 5.
5 to 8. 6 in 12, 6 in 8, 0 in 5

[mattymoo]

Wow, the first riddle on your channel I was actually able to figure out!

[debries]

It's really satisfying to solve this and then see the video go through each step you took, more than it should be >.>

[justingolden]

Solution I came up with before watching: First pour 5 into the 5liter, then pour that into the 8, then pour 5 into the 5liter and fill the top of the 8. now there are 2liters in the 5 container. pour the remainder of the milk back into the 12 and repeat 2 more times to get 6 (requires another container to hold the milk)