filmov
tv
Complex numbers: Solving Equations (with example)
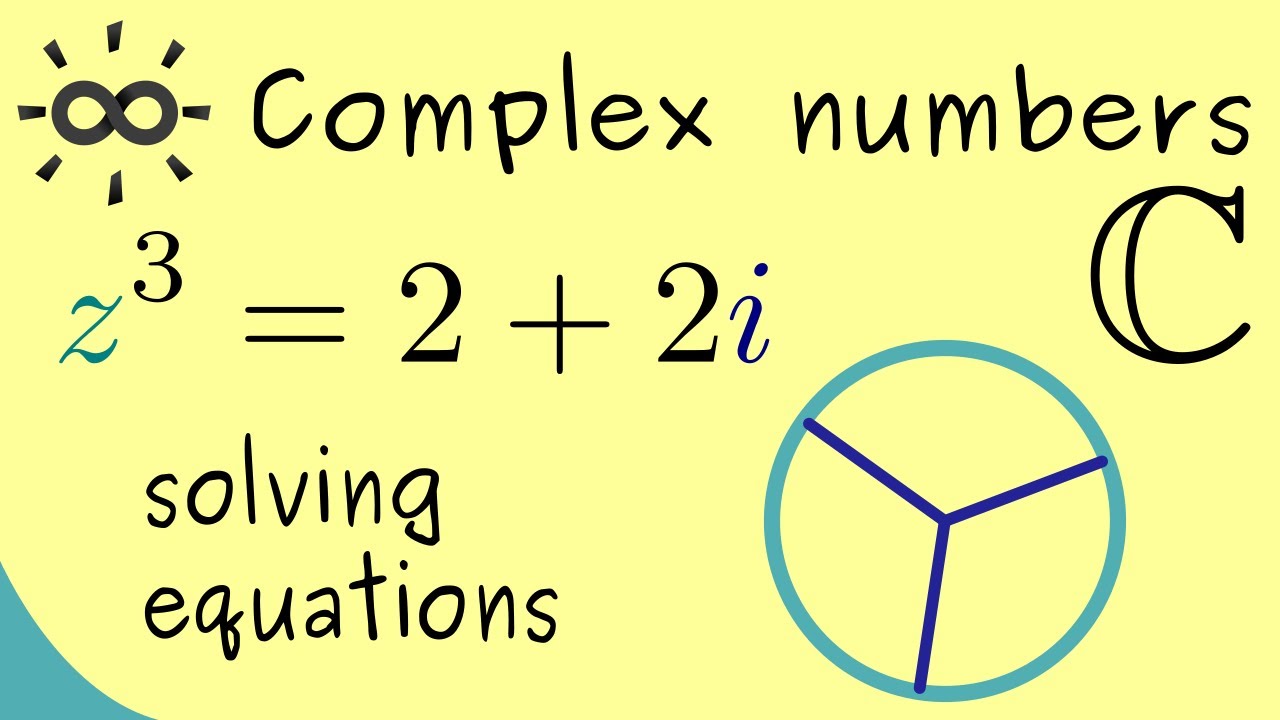
Показать описание
Thanks to all supporters! They are mentioned in the credits of the video :)
#StartLearningMathematics
This video is about solving simple equations with complex numbers, also known as extracting roots from complex numbers. There is a simple algorithm and a nice visualisation for this.
I hope that this helps students, pupils and others.
(This explanation fits to lectures for students in their first year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)
Solving Equations With Complex Numbers
Complex numbers: Solving Equations (with example)
Solving Equations with Complex Numbers 4 Examples
Solving equations with complex numbers
9. Complex Numbers: Solving Simultaneous Equations
Solving using the quadratic formula with complex solutions
Solving the Quintic Equation z^5 + 32 = 0 - Complex Analysis
03 - Equations involving Complex Numbers
Calculator Technique, Solving Simultaneous Equations On Casio Fx-991 Es Plus -
Complex Numbers - Practice Problems
Find Complex Roots of a Cubic Equation z^3 - 3z^2 + z + 5 = 0
Equations with Complex Solutions (2 of 2: Solving & factorising)
Pre-Calculus - Learn the basic operations of complex numbers
Simplifying Complex Numbers
Complex Numbers (How to find the nth root) : ExamSolutions Maths Video Tutorials
using calculator to solve complex numbers equations (matrix creamer's rule)
Complex Numbers (1 of 6: Solving Harder Complex Numbers Questions) [Student requested problem]
Algebra 2 - When Complex numbers are equal to each other, 5x + 1 + (3 + 2y)i = 2x - 2 + (y - 6)i
Precalculus 12.1a - Complex Quadratic
5 simple unsolvable equations
Factoring polynomials using complex numbers | Khan Academy
Solving Equations with Complex (Imaginary) Solutions | Math with Professor V
Example: Complex roots for a quadratic | Algebra II | Khan Academy
Simultaneous equations with complex numbers (Advanced Mathematics)
Комментарии
 0:02:07
0:02:07
 0:16:06
0:16:06
 0:29:37
0:29:37
 0:03:34
0:03:34
 0:04:07
0:04:07
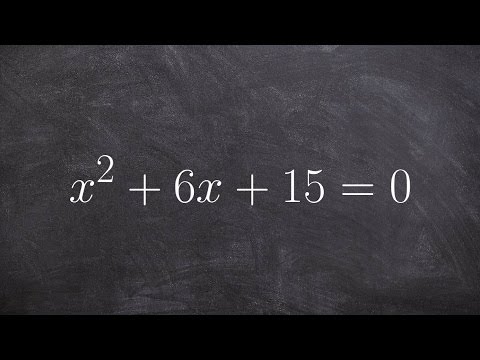 0:05:28
0:05:28
 0:04:17
0:04:17
 0:08:06
0:08:06
 0:01:01
0:01:01
 0:12:55
0:12:55
 0:05:54
0:05:54
 0:11:29
0:11:29
 0:10:46
0:10:46
 0:05:57
0:05:57
 0:14:21
0:14:21
 0:04:41
0:04:41
 0:10:36
0:10:36
 0:03:13
0:03:13
 0:09:01
0:09:01
 0:00:50
0:00:50
 0:03:10
0:03:10
 0:22:24
0:22:24
 0:10:15
0:10:15
 0:06:34
0:06:34