How to find the arc length of a semicircle using calculus.

We find the arc length of a circle of radius 1 by using the standard formula for arc length in rectangular coordinates. Bonus: one minute derivation of the arc length formula as a reminder!

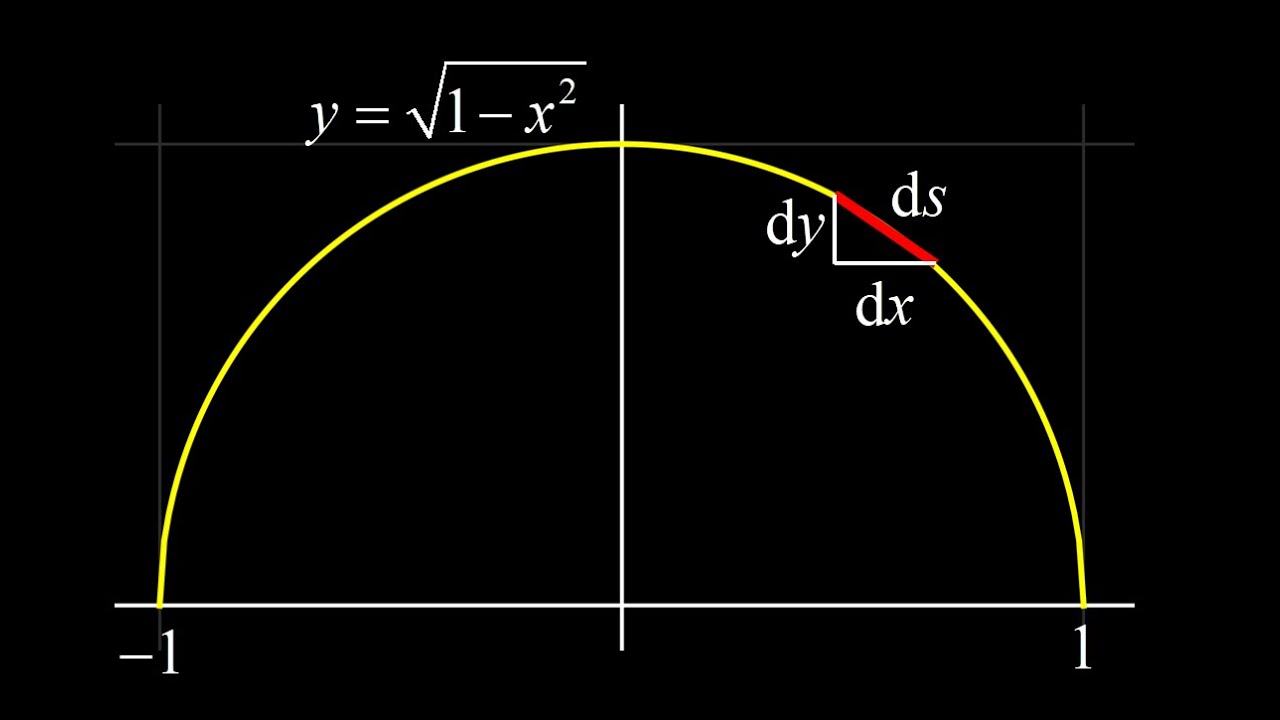
To compute the arc length of a semicircle, we need a formula for the semicircle as a function, so starting with the formula x^2+y^2=1 for a circle of radius 1 centered at the origin, we solve for y and get y=sqrt(1-x^2) for the upper half of the circle.

Next, we quickly derive the formula for the arc length in rectangular coordinates (in other words, in terms of x and y), so we get our usual formula for the arc length contribution ds as sqrt(1+(y')^2)dx.

Finally, we plug into our formula and manipulate the expression for the arc length increment into the form 1/sqrt(1-x^2). We recognize this expression as the derivative of the inverse sine function, so we quickly guess the antiderivative and evaluate across the limits of integration to get the answer we expected for the arc length of a semicircle using calculus: pi!

[oa]

this is brilliant, this should have way more views, , , beautifully explained

[fundopreto]

This is a great way to show the methods of calc II, but it is not actually a proof, since the values of sine (which are used in the end when you plug the 1 and 0 to the inverse sine function) depend on the fact that π is the ratio between the circumference and the diameter in the first place, so it is kind off circular reasoning. Great content to someone who is studying calculus nonetheless.