Olympiad Geometry Problem #8: Perpendiculars, Midpoints, Equal Angles

preview_player
Показать описание
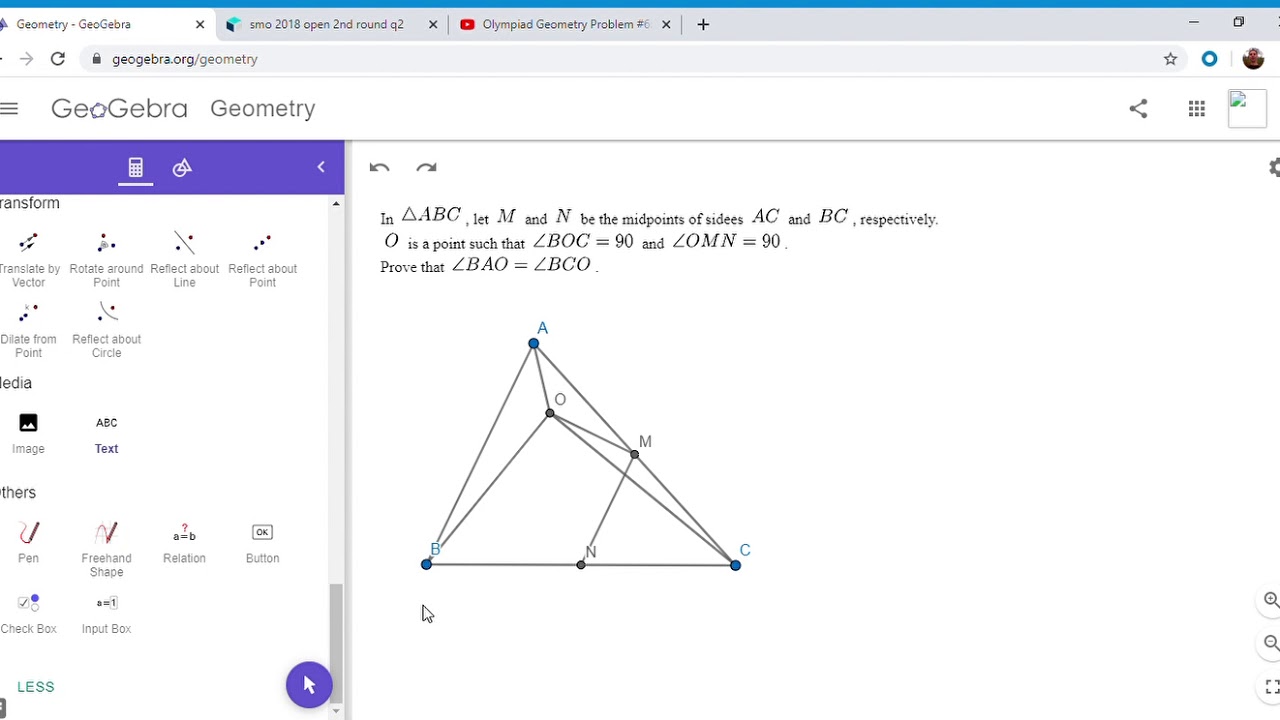
This is a simple but fun problem from SMO 2018. A couple of right angles are given, and you want to use that to show two angles are equal. Enjoy!
  1. Michael Greenberg
Рекомендации по теме
Olympiad Geometry Problem 0:08:03

Olympiad Geometry Problem #8: Perpendiculars, Midpoints, Equal Angles

Olympiad Geometry Problem 0:10:55

Olympiad Geometry Problem #6: Isosceles Triangle, Parallels, Perpendicular

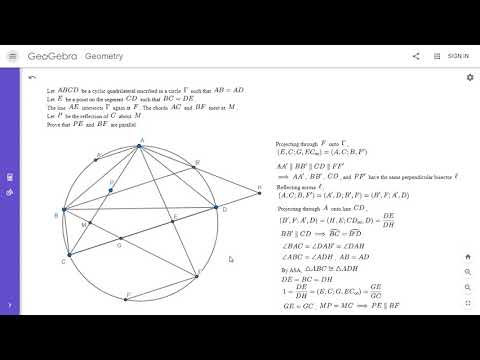
Olympiad Geometry Problem 0:07:54

Olympiad Geometry Problem #100: Circumcenter Perpendiculars Cyclic Quad

Olympiad Geometry Problem 0:12:29

Olympiad Geometry Problem #30: Incenter, Equal Segments, Perpendicular

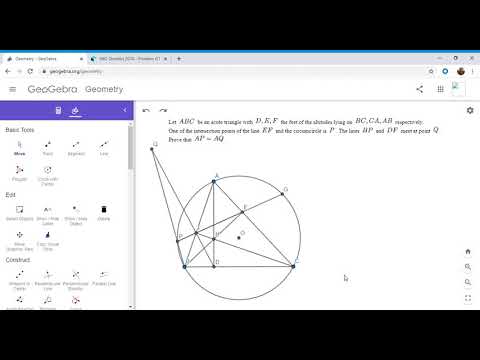
Olympiad Geometry Problem 0:11:05

Olympiad Geometry Problem #10: Isosceles Triangle, Midpoints, Perpendiculars

Olympiad Geometry Problem 0:13:05

Olympiad Geometry Problem #80: Incircle, Reflections, Perpendicular

What is the 0:03:09

What is the value x? | Perpendicular Angle Bisectors | 8th Grade Math Olympiad

Olympiad Geometry Problem 0:22:52

Olympiad Geometry Problem #89: Reflections, Tangents, Perpendicular

Q481 | Math 0:03:02

Q481 | Math Olympiad | Geometry | Isosceles Right Triangle | Angle Bisector Theorem

Olympiad Geometry Problem 0:10:33

Olympiad Geometry Problem #35: Angle Bisector, Perpendiculars, Tangency

What’s the area? 0:00:42

What’s the area?

Olympiad Geometry Problem 0:12:48

Olympiad Geometry Problem #99: Cyclic Quad, Equal Segment, Parallel

Geometry Problem | 0:00:44

Geometry Problem | Finding the Missing Angle | SAT Prep | Math Problem

Olympiad Geometry Problem 0:11:20

Olympiad Geometry Problem #3: Right Triangle, Perpendiculars, Equal Angles

Geometry everyone should 0:00:15

Geometry everyone should learn

Olympiad Geometry Problem 0:13:48

Olympiad Geometry Problem #91: Two Perpendiculars, Midpoint, Equal Segments

Lines and Angles 0:00:34

Lines and Angles Trick | Class 9 Maths Lines and Angles #fun #shorts #mathsiseasy #youtubeshorts

Tamasha Dekho 😂 0:00:13

Tamasha Dekho 😂 IITian Rocks Relatives Shock 😂😂😂 #JEEShorts #JEE #Shorts

Q474 | Math 0:03:10

Q474 | Math Olympiad | Geometry | 2024 AMC 10B Problem 11 | Triple Perpendicular Similarity Model

Missing Angles Geometry 0:00:37

Missing Angles Geometry Problem | Tricky Math Question | JusticeTheTutor #maths #math #shorts

HOW TO FIND 0:00:14

HOW TO FIND PERIMETER #perimeter #formulae #rectangle #circle #triangle #maths #shorts #ytshorts #yt

Olympiad Geometry Problem 0:12:18

Olympiad Geometry Problem #18: Altitudes, Circumcircle, Equal Segments

FULL FORM OF 0:00:41

FULL FORM OF MATHS😍#maths #MATHSFUN#shorts #viral

Last Words of 0:00:37

Last Words of Albert Einstein #shorts

Комментарии
Автор

Wow, that is really a nice solution :D
And here is mine :

Let OM intersect AB at E and let F be the reflection of C respect to BO, so we get F, O and C colliner and FA//OM. İt is enough to show that FAOB is cyclic. <OFA = <FOE = <EBO = <ABO and we get FAOB is cyclyc as desired.

kerimbendov
Автор

neat insight on the parallelogram construction! here's mine:
-> Construct F to be the foot of the altitude from C to AB. Notice that BFOC is cyclic.
-> Extend OM such that it intersects AB at X. By some angle chasing (on quadrilateral XMNB), we find that MX must be perpendicular to AB, implying that MX//CF. Since M is the midpoint of AC, X must be the midpoint of AF. Thus, O lies on the perpendicular bisector of AF.
-> From this, <BAO = <XAO = 90 - <AOX = 90 - <FOX = 90 - <OFC = 90 - <OBC = <BCO

justinvillafuerte
Автор

Let P be the point on the extension of OM such that MP=MO. Then AOCP is a parallelogram and NM is an altitude and a median in triangle ONP. Therefore NO=NP and P lies on the circumcircle of BOC and angle BPC = 90. AO=CP. Let`s take D such that DBPC is a rectangle. Then D lies on the circumcircle of BOC and BD=CP. Therefore BD=CP=AO and AO || CP || BD. It follows that AODB is a parallelogramm and angle BAO = angle BDO = angle BCO.

rustamnovikov