filmov
tv
6. The principle of equivalence.

Показать описание
MIT 8.962 General Relativity, Spring 2020
Instructor: Scott Hughes
Introduction to the principle of equivalence: freely falling frames to generalize the inertial frames of special relativity. Two important variants of the equivalence principle (EP): The weak EP (one cannot distinguish free fall under gravity from uniform acceleration over 'sufficiently small' regions); the Einstein EP (the laws of physics in freely falling frames are identical to those of special relativity over 'sufficiently small' regions).
License: Creative Commons BY-NC-SA
Instructor: Scott Hughes
Introduction to the principle of equivalence: freely falling frames to generalize the inertial frames of special relativity. Two important variants of the equivalence principle (EP): The weak EP (one cannot distinguish free fall under gravity from uniform acceleration over 'sufficiently small' regions); the Einstein EP (the laws of physics in freely falling frames are identical to those of special relativity over 'sufficiently small' regions).
License: Creative Commons BY-NC-SA
6. The principle of equivalence.
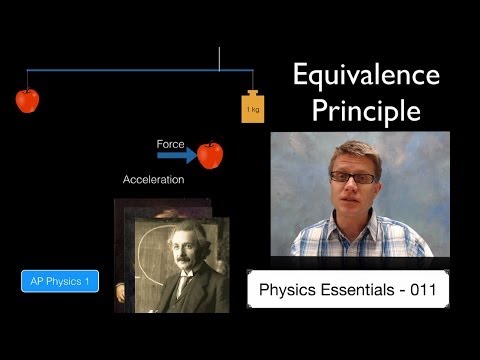
Equivalence Principle
Topic 7.2.6 - Intro to General Relativity and the Principle of Equivalence
Classroom Aid - Equivalence Principle
Relativity 107a: General Relativity Basics - Equivalence Principle and Proper Acceleration
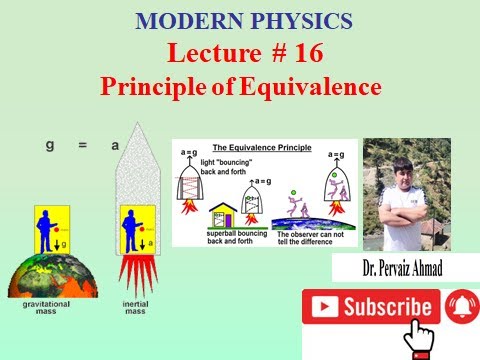
HTPIB33A Principle of Equivalence
Astronomy - General Relativity (3 of 17) What is the Equivalence Principle?
12.1 Equivalence Principle
Principle of Equivalence and Curvature of Light
Equivalence principle
Einstein’s PRINCIPLE OF EQUIVALENCE
General Relativity! Einstein's Equivalence Principle and the Curvature of Space-time | Doc Phy...
Principle of Equivalence
Einstein's principle of equivalence passed a quantum test
Principle of Equivalence
The Equivalence principle!
The Principle of Equivalence
Science of Motion 7: Principle of Equivalence
6. Falling Catboys and the Principle of Equivalence
Principle of Equivalence: 'Galileo Experiment' at Home
Astronomy - General Relativity (4 of 17) The Equivalence Principle: Proven in 1919
Six Sigma In 9 Minutes | What Is Six Sigma? | Six Sigma Explained | Six Sigma Training | Simplilearn
#short working principle of Transformer
Thevenin's Theorem - Circuit Analysis
Комментарии
 1:20:06
1:20:06
 0:06:16
0:06:16
 0:10:34
0:10:34
 0:05:56
0:05:56
 0:25:03
0:25:03
 0:03:21
0:03:21
 0:07:25
0:07:25
 0:01:58
0:01:58
 0:06:07
0:06:07
 0:00:15
0:00:15
 0:09:57
0:09:57
 0:14:39
0:14:39
 0:12:31
0:12:31
 0:01:50
0:01:50
 0:45:41
0:45:41
 0:00:16
0:00:16
 0:05:44
0:05:44
 0:03:49
0:03:49
 0:46:32
0:46:32
 0:00:41
0:00:41
 0:05:31
0:05:31
 0:08:59
0:08:59
 0:00:10
0:00:10
 0:09:23
0:09:23