Solving equations with lambert w function #shorts

preview_player
Показать описание

  1. Dr Ji Tutoring
Рекомендации по теме
Solving 8 Equations 0:40:26

Solving 8 Equations w/ Lambert W function

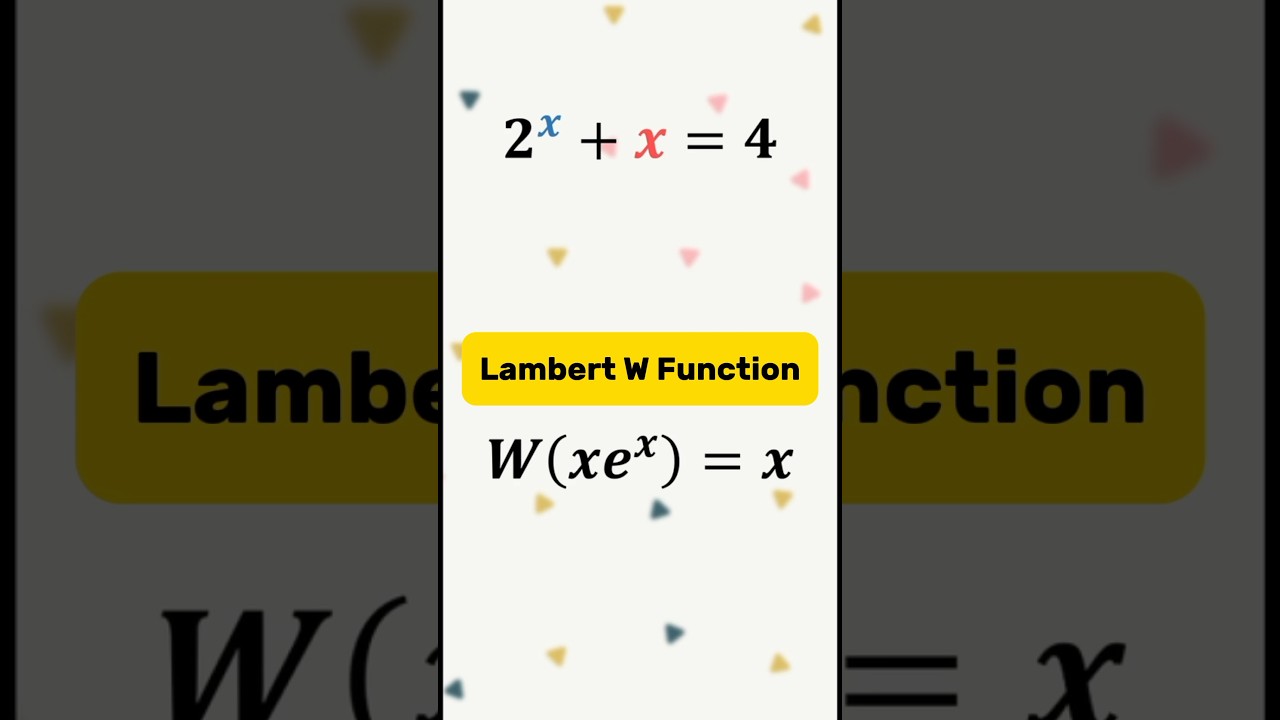
Solving equations with 0:01:00

Solving equations with lambert w function #shorts

Lambert W Function 0:14:35

Lambert W Function

Solving a tricky 0:03:45

Solving a tricky equation with the Lambert W function.

Lambert W Function 0:06:06

Lambert W Function solving exponential equations

Using the LAMBERT 0:25:14

Using the LAMBERT W FUNCTION find ALL solutions! / ( W_0 and W_-1)

Simple Yet Difficult. 0:03:39

Simple Yet Difficult. How to Solve Using Lambert W Function

Can you solve 0:05:13

Can you solve this exponential equation

Solve Math Olympiad 0:10:21

Solve Math Olympiad Problems with the POWER of Lambert W Function!

The Derivative of 0:00:59

The Derivative of the Lambert W Function #shorts

Lambert W Function 0:04:40

Lambert W Function - solving exponential equations

Yes, you could 0:05:46

Yes, you could solve it other ways but WHY do that when you have the Lambert W Function!?!?

See what Lambert 0:01:00

See what Lambert W function can do with x^x=3

What is the 0:07:50

What is the Lambert W Function (Introduction )/ Part 1

Lambert W Function 0:11:24

Lambert W Function (No real solution???)

Find X (Lambert 0:04:43

Find X (Lambert W Function - application)

Solving Equations with 0:01:31

Solving Equations with the Lambert W function

you haven't seen 0:00:53

you haven't seen this before! @blackpenredpen

Solving Equation With 0:04:29

Solving Equation With Lambert W function | Lambert W function

Solving Exponential Equation 0:00:58

Solving Exponential Equation (using Lambert W function)

How to Apply 0:10:20

How to Apply Lambert W Function To Solve Exponential Equations With Variables in the Base & Expo...

Cool Identity of 0:00:55

Cool Identity of the Lambert W Function #shorts

How to Solve 0:07:02

How to Solve Equations using the Lambert W or Product Log Function. ALGEBRA Challenge

People Say 'YOU 0:00:33

People Say 'YOU CANNOT FACTOR x^2+1'

Комментарии
Автор

it feels like the Lambert w function is a pretty useful exercise for spotting invisible things that will lead to solutions. The math is crazy, and this skill is not something humans are designed for.

In schools teachers can't present information and in college they expect you to be a genius and get everything straightaway without any practicing

cmcumm
Автор

There's no explanation as to why the given solution is helpful/useful for anything though.

MsHojat
Автор

Thanks for NOTHING! That explanation says nothing about what exact # will solve the equation.

StevenTorrey
Автор

Why is it +4 and not -4 if we moved 4 to the left

kami
Автор

a^x + x = b
x = b - [w(a^b • lna)]/lna

2^x + x = 4
So x = 4 - [w(2^4 • ln2)]/ln2
= 4 - [w(16ln2)]/ln2

cosmolbfu
Автор

It seems like the exponent needs to match the added x term to work out. I made a gen solution doing the same process.

AB^(Cx^n) + Cx^n = D

x^n = [-W(AB^D * lnB)/lnB + D] / C

If there’s a way to do it where they don’t need to match as Cx^n, what is it?

darcash
Автор

This is tantamount to nonsense, let take an equation that you can easily solved using numerical method fanny around with it to get it define in terms of a function you can determine using numerical methods.

cupidstunt
Автор

I don't have a clue what that whole explanation is. What i do know is: 2X+X=3X. So so X is 1.66667 in my head

MarkGlowInTheDark