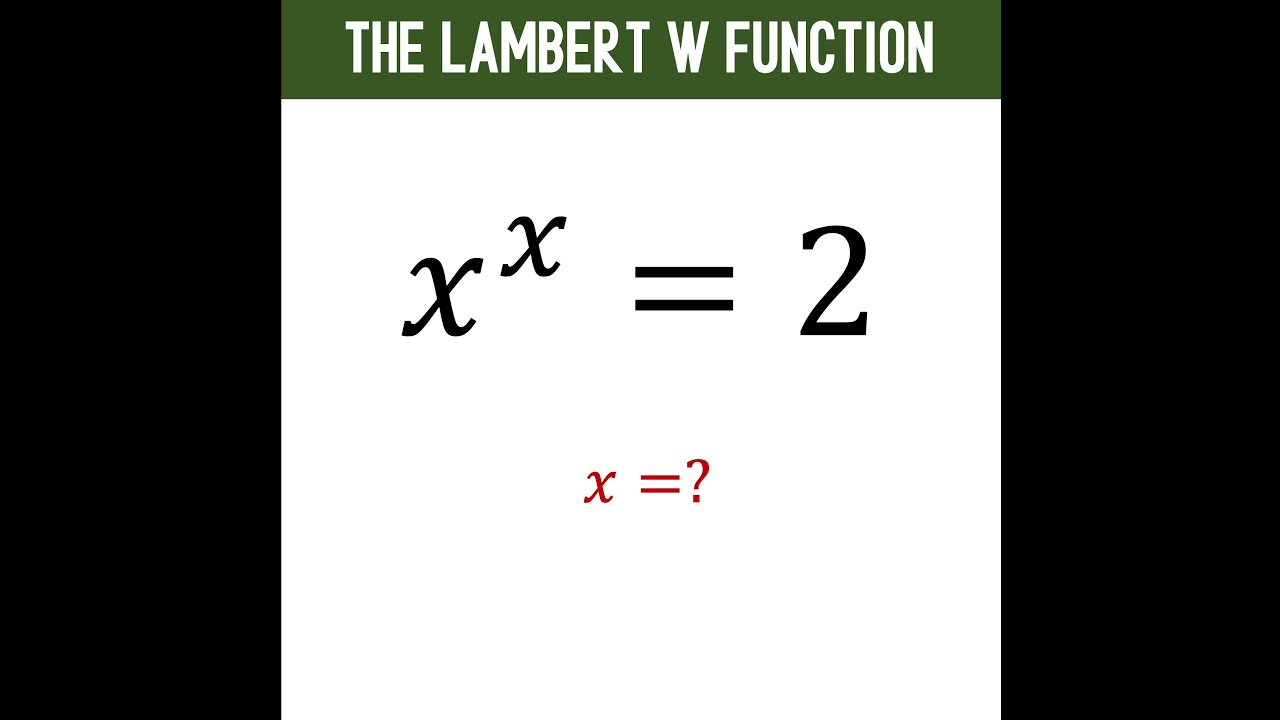
Simple Yet Difficult. How to Solve Using Lambert W Function

A Simple Equation That Turned Out to Be Needing an Unfamiliar Function to Be Solved Algebraically #algebra | The Lambert W Function

[XanderAnimations]

Fun fact: e^W(lnx) is the formula to find the *Super Square Root!*

Notice how x^x is a tetration equation, it's x tetrated to 2 or x^^2.

The solution to x^^2 = a is the super square root (ssqrt) of a, or e^W(lna)

[jim]

Cool beans! Never knew about the Lambert W function. This was a very helpful introductory video to the function. Thanks.

[buckyjennings]

What is the point if you still need a computer program to give you the value?

[ayushoriginal]

Simply assume x = 2
Thus square root both side you get the answer...
Which is x = √2
I.e 1.414 approximately

[souzasilva]

How do you find 16 in Lambert's formula? Not how you find the indices 0 and -1 of W.

[homoexhumo]

I set x=1 and n=2

Then I apply the recursive relationship:

x=(n^(1/x) + x)/2

As it stands, this method converges fairly quickly to the more general problem x^x = n for some range of real values ​​of n. It has been many years since I worked on this problem, and I don't remember much of the details, except that the above method undergoes many changes depending on the range of values ​​of n. The boundaries of these values, if I remember correctly, depend on the constant e. In general, this method and its variants also work for complex values ​​of n.