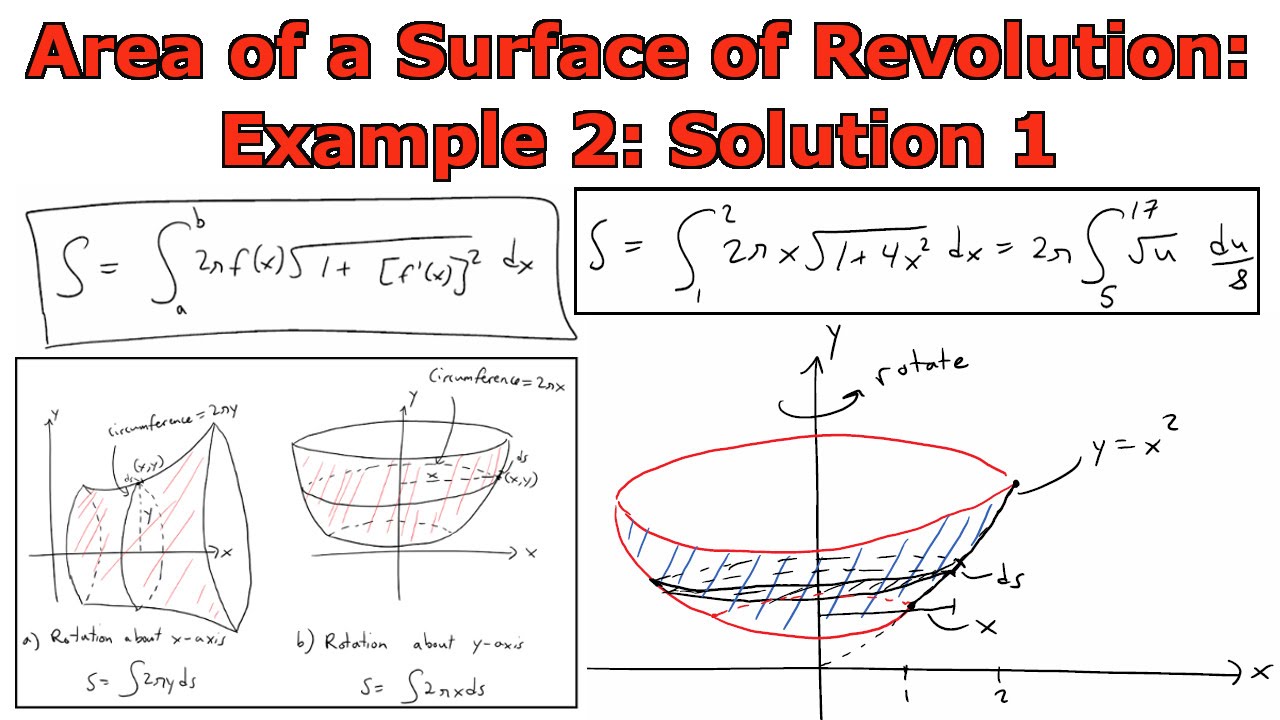
Applications of Integrals: Surface Area: Example 2: Solution 1

In this video I go over another example on determining the area of a surface obtained by rotating a curve about an axis. In this example, I calculate the surface area of a shape formed by rotating the arc of the parabola y = x^2 from the point (1, 1) to (2, 4) about the y-axis. As a check of the answer obtained, I calculate the shape of a cylinder as well as a frustum of a cone that is similar to the shape we are trying to calculate, thus the resulting surface areas should all be similar as well.

The solution I use in this video uses the surface area formula in which the arc length is written using dx as opposed to dy. In my next video I solve this same example using dy in the arc length formula instead so stay tuned for that video!

Related Videos:

------------------------------------------------------

[mes]

I don't always determine the surface area of a shape but when I do I usually solve it using dx in the arc length formula ;)