filmov
tv
Intuition: Why the Lorentz Transformation is Linear

Показать описание
One of the most important starting points for deriving the Lorentz transformation is the fact that it is a linear transformation. Why do we get to assume that? Here we explain the intuition behind why we expect the Lorentz transformation to be linear, using the coordinate systems of special relativity and how rulers and clocks change between inertial reference frames.
0:00 Introduction
4:48 T(u+v) = T(u)+T(v)
15:13 T(cv) = cT(v)
Subscribe to see more new math videos!
Music: OcularNebula - The Lopez
Intuition: Why the Lorentz Transformation is Linear
The Lorentz Transformations - Intuitive Explanation
Lorentz Transformations | Special Relativity Ch. 3
Introduction to the Lorentz transformation | Special relativity | Physics | Khan Academy
Introduction to the Lorentz transformation
Uncanny Relativity: Lorentz Transformations - the easy intuitive way
Spacetime rotations, understanding Lorentz transformations
Deriving the Lorentz Transformations | Special Relativity
Length contraction: the real explanation
How Special Relativity Makes Magnets Work
The physical meaning that Einstein attributes to Lorentz transformations
Discovering Lorentz transformation with Albert Einstein
Derivation of Lorentz Transformation (Lecture #02b of a course on Relativity & Cosmology)
Relativity 104a: Special Relativity - Lorentz Transformation Geometry (no equations)
Deriving the General Lorentz Transformation | Special Relativity
4.5 Lorentz Transformation
Evaluating a Lorentz transformation | Special relativity | Physics | Khan Academy
Lorentz Transformations VS Galilean Transformations | Special Relativity
Lorentz Transform Derivation part 1: Problem With Galilean Transforms
Relativity 104b: Special Relativity - Lorentz Transform Equations Derivation
What is the Lorentz Transformation?
Why is Relativity Hard? | Special Relativity Chapter 1
Algebraically manipulating Lorentz transformation | Physics | Khan Academy
Relativity: how people get time dilation wrong
Комментарии
 0:20:19
0:20:19
 0:16:56
0:16:56
 0:12:18
0:12:18
 0:08:20
0:08:20
 0:04:13
0:04:13
 0:07:01
0:07:01
 0:15:37
0:15:37
 0:17:35
0:17:35
 0:11:23
0:11:23
 0:04:19
0:04:19
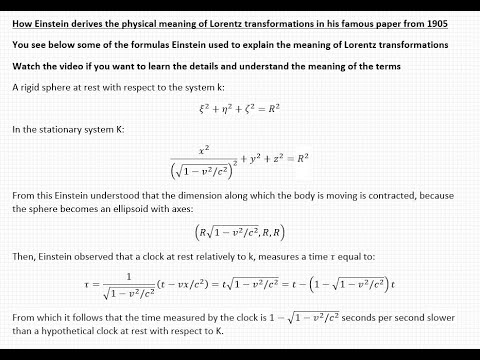 0:07:06
0:07:06
 0:36:06
0:36:06
 0:11:59
0:11:59
 0:15:19
0:15:19
 0:08:24
0:08:24
 0:06:54
0:06:54
 0:08:40
0:08:40
 0:02:26
0:02:26
 0:07:13
0:07:13
 0:17:23
0:17:23
 0:04:21
0:04:21
 0:04:50
0:04:50
 0:08:00
0:08:00
 0:11:07
0:11:07