Complex Analysis: Residue Theorem Proof

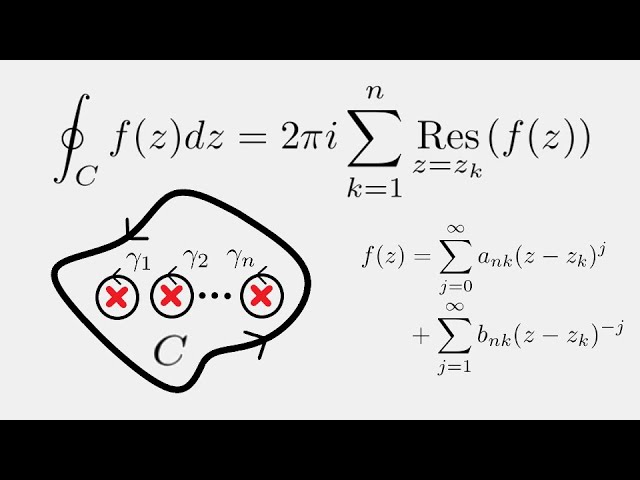
Показать описание

Today, we derive Cauchy's Residue Theorem, one of the most useful results in complex analysis.

Рекомендации по теме

Комментарии

But how you get on how to calculate the residue? It's kind of magic this "definition" of the bik...
Do you have any idea on how Cauchy figure it out that bik it's a residue and how it was calculated?

wduandy

Great stuff. Complex analysis is crazy!!!

tomatrix

As an engineer I see it in a different manner,
In the integral of this product F(z). dz rotates then the answer is zero and if it does not then over one revolution the integral is a constant multiplied by 2 pi.

If F(z) has many poles the say one being
K/ (z-a) then the integrating around the pole a the product K dz/(z-a) does not rotate and it has a value while while going around point a all the other integrals will be insignificant,

Then the procedure is repeated around pole, b and pole c where by the total summation is

2pi( summation of the residues)
If the denominator 1/(z-a)^n is has n not equal to 1 then dz/ ( z-a) ^n rotates and the integral is zero,

It is all a case of watching whether the integral rotates of whether it does not rotate. and it will be a linear integral related to the circumference of the circle, .

carmelpule

The concept and proof of the residue theory is trivial to understand

It's difficult to execute. That's how I found your video. I think math people don't understand the difficulty for the rest of us. There isn't a direct line from proof -> execution for the majority of ppl

wilurbean

Wow, perfect, neat, concise and very simply written. Non comment, awesome. Thanks a lot.

annacersongor

Extraordinary explanation. This video should be the first result When searching for residue theorem

dosame

I’m very enthusiastic about this topic. Furthermore, I’m also enjoying your sophisticated content. G’day and keep uploading more videos about contour integral.

captainkim

You: that's me ! (Be sure and also be relax, I didn't think it's Joker! 😀😀)
When I watching your video, I just enjoyed (it's me🤓, when I'm watching your videos 🤓)
Thank you so much

wuyqrbt

Hello Dear *QN³*
Please make a video about *Laurent Series* .
Thank you so much

wuyqrbt

Very useful and concise presentation to proof the Cauchy Residue thrown utilizing Laurent series.

saeida.alghamdi

Complex Analysis: Residue Theorem Proof

Complex Analysis: Residue Theorem Proof

Cauchy's Residue Theorem with Proof | Complex Analysis #17

Residue Theorem and Proof

Cauchy's Residue Theorem with Examples | Complex Integration | Complex Analysis #16

Complex Analysis 34 | Residue theorem

Complex integration, Cauchy and residue theorems | Essence of Complex Analysis #6

Cauchy's Residue Theorem Proof (Complex Analysis)

Complex Analysis L09: Complex Residues

Cauchey Residue Theorem - proof

Mod-04 Lec-12 Proofs of Two Avatars of the Residue Theorem for the Extended Complex Plane

The residue theorem part 1 | Complex Analysis | LetThereBeMath |

Evaluating Real Integrals With Cauchy's Residue Theorem - Complex Analysis By A Physicist

Complex Analysis 35 | Application of the Residue Theorem

How to find the Residues of a Complex Function

Complex Analysis L10: Cauchy Integral Formula

The residue theorem -- Complex Analysis 22

Cauchy's Residue Theorem - Complex Analysis By a Physicist

Cauchy Residue Theorem, Introduction

Cauchy residue theorem|Statement and Proof|Complex Analysis|Theta Classes

Basic Complex Analysis - Unit 3 - Lecture 17 - Residue Calculation at Simple Pole

Inverse Laplace Transform Using The Residue Theorem

Complex Analysis: Integral of sin(x)/x using Contour Integration

Basic Complex Analysis - Unit 3 - Lecture 4 - Cauchy's Residue Theorem

Residue theorem for real integrals 1 | Complex Analysis | LetThereBeMath |