Random Clifford circuits for quantum coding against Pauli noise using a tensor-network decoder

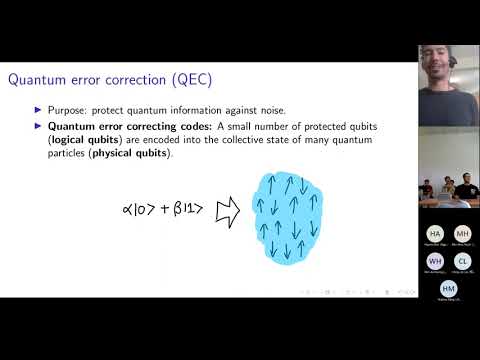
Recent work [M. J. Gullans et al., Physical Review X, 11(3):031066 (2021)] has shown that quantum error correcting codes defined by random Clifford encoding circuits can achieve a non-zero encoding rate in correcting errors even if the random circuits on n qubits, embedded in one spatial dimension (1D), have a logarithmic depth d=O(logn). However, this was demonstrated only for a simple erasure noise model. In this work, we discover that this desired property indeed holds for the conventional Pauli noise model. Specifically, we numerically demonstrate that the hashing bound, i.e., a rate known to be achieved with d=O(n)-depth random encoding circuits, can be attained even when the circuit depth is restricted to d=O(logn) in 1D for depolarizing noise of various strengths. This analysis is made possible with our development of a tensor-network maximum-likelihood decoding algorithm that works efficiently for log-depth encoding circuits in 1D.