QIP2021 | Epsilon-nets, unitary designs and random quantum circuits (Michal Oszmaniec)

Authors: Michal Oszmaniec, Adam Sawicki and Michal Horodecki
Affiliations: Center for Theoretical Physics, Polish Academy of Sciences | Center for Theoretical Physics, Polish Academy of Sciences | International Centre for Theory of Quantum Technologies, University of Gdansk

Abstract
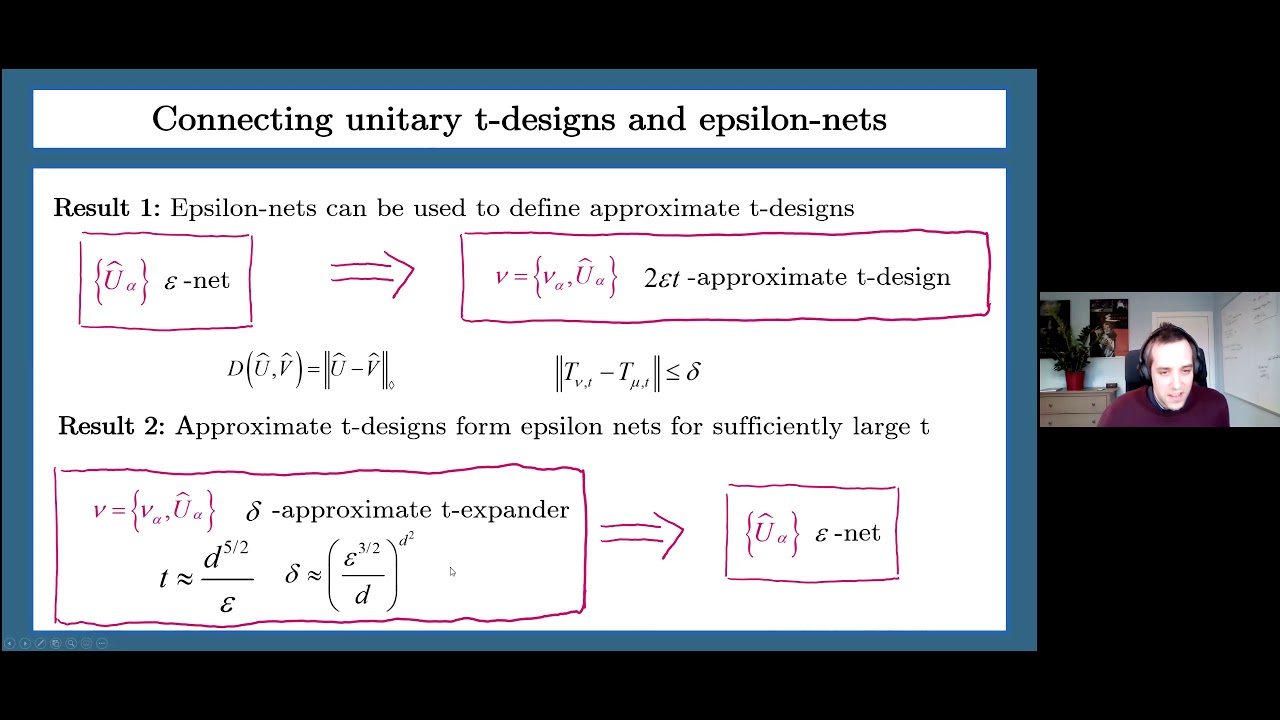
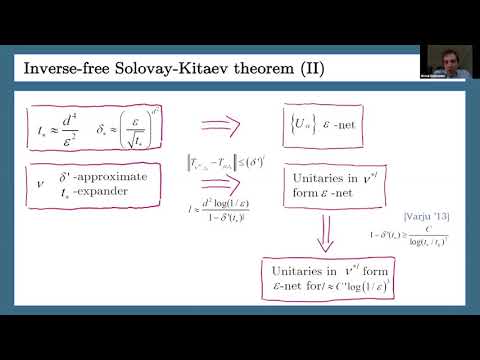
Epsilon-nets and approximate unitary t-designs are natural notions that capture properties of unitary operations relevant for numerous applications in quantum information and quantum computing. The former constitute subsets of unitary channels that are epsilon-close to any unitary channel in the diamond norm. The latter are ensembles of unitaries that (approximately) recover Haar averages of polynomials in entries of unitary channels up to order t. In this work we systematically study quantitative connections between these two notions. Specifically, we prove that, for a fixed dimension d of the Hilbert space, unitaries constituting delta-approximate t-expanders form epsilon-nets for t~(d^(5/2))/epsilon and delta~[(epsilon^(3/2))/d]^(d^2). We also show that epsilon-nets can be used to construct delta-approximate unitary t-designs for delt~epsilon*t, where the notion of approximation is based on the diamond norm. Finally, we prove that the degree of an exact unitary t-design necessary to obtain an epsilon-net must grow at least fast as 1/epsilon (for fixed dimension) and not slower than d^2 (for fixed epsilon). This shows near optimality of our result connecting t-designs and epsilon-nets. We further apply our findings in conjunction with the recent results of Varju 2013 in the context of quantum computing. First, we show that that approximate t-designs can be generated by shallow random circuits formed from a set of universal two-qudit gates in the parallel and sequential local architectures considered in Brandao-Harrow-Horodecki 2016. Importantly, our gate sets need not to be symmetric (i.e. contains gates together with their inverses) or consist of gates with algebraic entries. Second, we consider a problem of compilation of quantum gates and prove a non-constructive version of the Solovay-Kitaev theorem for general universal gate sets. Our main technical contribution is a new construction of efficient polynomial approximations to the Dirac delta in the space of quantum channels, which can be of independent interest.

Get entangled with us!