🔵11 - Homogeneous First Order Differential Equations (Solved Examples)

Показать описание

In this video, we shall study homogeneous differential equations and solve a couple of them
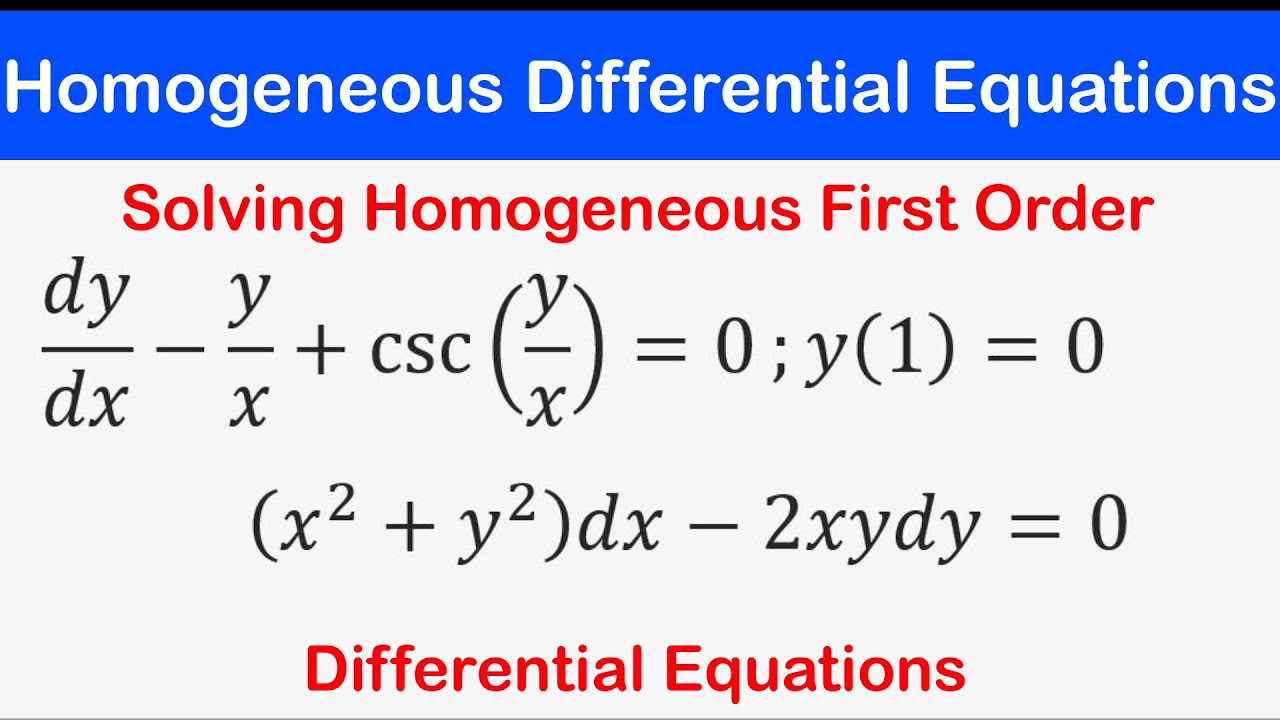
A differential equation of the form M(x,y)dx + N(x,y)dy is said to be homogeneous if the functions M and N are homogeneous functions of the same degree.
Alternatively a differential equation of the form
dy/dx = F(x,y) is said to be homogeneous if the function F(x,y)
is homogeneous of degree 0.
Homogeneous differential equations are not of variable separable type but can be made separable by plugging in two equations;
1. y = vx
2. dy/dx = v + xdv/dx

After substitution the differential equations becomes separable

In this lesson we shall solve three major examples

00:00 - Homogeneous Differential Equations
04:01 - Ex 1
17:34 - Ex 2
31:01 - Ex 3

Playlists on various Course
1. Applied Electricity

2. Linear Algebra / Math 151

3. Basic Mechanics

4. Calculus with Analysis / Calculus 1 / Math 152

5. Differential Equations / Math 251

6. Electric Circuit Theory / Circuit Design

Make sure to watch till the end.
Like, share, and subscribe.
Thank you.

Рекомендации по теме

Комментарии

You out here saving lives. Really glad i found this channel thanks a lot fam

kakabraza

if we use the constant c instead of ln(c) the answer will be different . so which one is correct

wondifrawterefe

Why is lnc on the left and not the right?

thee_pauline

My guy you're very good 😮
Keep it up bro

ephraimdanso

Please what if we have more than two terms containing x and y. How will you go about it?

edwardwiafeaidoo

I want to get something right here, so if you find out the equation is homogeneous then we do substitution before preceeding to the method of separations.

Kelvin-cs

The degree of the third example was not stated? Or its not needed in this instance ?

obedaboagye

Thanks so much for your videos, but please why do you use ln(c) instead c after the integration, is it the same?

GillsOsei-bekl

I doubt your answer for Example 2, becase you was suppoused to replace back c^2, when using the initial condition, for now you just found the value of c_1, the one you introduced.

jekoniandemulunda

please can v be replace by any other variable in the equation y=vx

SALIMAWEDADIBAMADDAH

🔵11 - Homogeneous First Order Differential Equations (Solved Examples)

🔵11 - Homogeneous First Order Differential Equations (Solved Examples)

How To Solve First Order Homogeneous Differential Equation

Solving Homogeneous First Order Differential Equations (Differential Equations 21)

Homogeneous First-Order Differential Equations (Introduction)

Homogeneous First-Order Differential Equations (Examples)

Homogeneous Differential Equations First Order3 Examples

the differential equations terms you need to know.

Substitutions for Homogeneous First Order Differential Equations (Differential Equations 20)

Basic Engg. Mathematics [ UNIT-V Chapter-1 Lec-4 Probability ] #racevabiharsemester

DIFFERENTIAL EQUATIONS | First-Order Homogeneous Equations

How to solve first-order Homogeneous differential equation

First Order homogeneous differential equation.mp4

01 - Linear First-Order Homogeneous Equations

Homogeneous first order ODE | Homogeneous differential equations | Math 02/07

🔵10 - Homogeneous Functions (Intro to Homogeneous First Order Differential Equations)

Differential Equations - 10 - First-Order Homogeneous Equations

First Order Homogeneous Differential Equations Lesson 2

First Order Homogeneous differential equations- Problem # 1

Homogeneous first order differential equations #ODEs #examples

Homogeneous first order ordinary differential equation

Determine if a First-Order Differential Equation is Homogeneous - Part 1

1st yr. Vs Final yr. MBBS student 🔥🤯#shorts #neet

First Order Linear Differential Equations

DiffEq: First Order - Homogeneous Example 1 [Not SAT]