filmov
tv
Geometric Algebra and Grading

Показать описание
One of the most important aspects of geometric algebra is the grading. However, for many people, this is the first time they encounter the concept of grading, so they don't know the relevant definitions. In this short, I show these definitions, and how they imply that while geometric algebra is both an algebra and a graded space, it is not a graded algebra.
Also, I know that under the Z/2Z grading, GA is a graded algebra. However, we don't use that grading too much, and the N-grading is much more useful.
Patreon Supporters:
Christoph Kovacs
David Johnston
Jason Killian
p11
Richard Penner
Rosario
trb
Also, I know that under the Z/2Z grading, GA is a graded algebra. However, we don't use that grading too much, and the N-grading is much more useful.
Patreon Supporters:
Christoph Kovacs
David Johnston
Jason Killian
p11
Richard Penner
Rosario
trb
Geometric Algebra and Grading
Geometric Algebra vs. Clifford Algebra
Quaternions Are Not Four-Dimensional Objects
An Overview of the Operations in Geometric Algebra
A Swift Introduction to Geometric Algebra
All Of Algebra 1 Explained In 5 Minutes
Maxwell's equation in geometric algebra
The Hardest Math Test
Grade 9 Maths Numeric and Geometric Patterns Lesson 2 - Number Pattern with Constant Ratio
All Motion Is Just Reflection
Duality transformations in geometric algebra
2.2 An Outline of Geometric Algebra | Geometric Algebra for Physicists
BASIC Algebra Equations - Quick Practice
Memorization Trick for Graphing Functions Part 1 | Algebra Math Hack #shorts #math #school
QED Prerequisites Geometric Algebra 9: Multivector Structure
What’s the area?
is geometry easier than algebra?
QED Prerequisites Geometric Algebra 6 - Multivector Products
QED Prerequisites Geometric Algebra 8 Better notation for basis vectors
Geometric Algebra in Julia with Grassmann.jl | Michael Reed | JuliaCon 2019
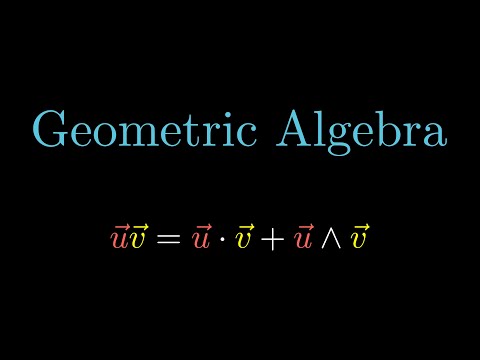
Geometric algebra
Can you guess the math formula?
Common Algebra Mistakes #1 #Shorts #algebra #mistake #mistakes #math #maths #mathematics #education
Algebra: FOIL Method #Shorts #algebra #math #maths #mathematics #education #learn
Комментарии
 0:00:49
0:00:49
 0:00:54
0:00:54
 0:00:41
0:00:41
 0:40:35
0:40:35
 0:44:23
0:44:23
 0:05:00
0:05:00
 0:09:28
0:09:28
 0:00:28
0:00:28
 0:21:07
0:21:07
 0:15:58
0:15:58
 0:18:23
0:18:23
 0:02:38
0:02:38
 0:00:41
0:00:41
 0:00:15
0:00:15
 0:58:11
0:58:11
 0:00:42
0:00:42
 0:00:08
0:00:08
 0:58:35
0:58:35
 0:55:15
0:55:15
 0:13:51
0:13:51
 0:15:44
0:15:44
 0:00:53
0:00:53
 0:00:12
0:00:12
 0:00:18
0:00:18